labeattie
Structural
- Aug 27, 2014
- 43
Hi all,
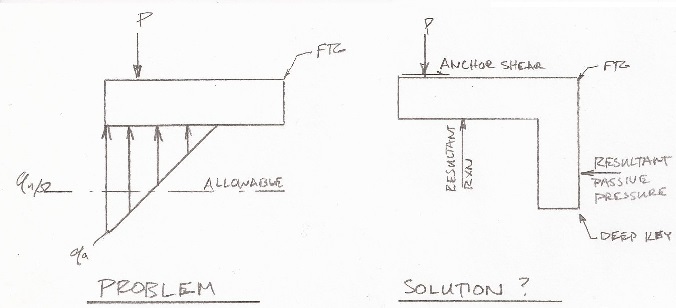
I'm having a frustrating problem that I would think has an easy answer. I'm designing a wall footing for bearing pressure right now, and can only increase the footing dimension in one direction. Imagine my surprise when my calculations say adding a foot in one direction of a footing increases the maximum pressure. I don't really believe this can be true. So I attached a basic calculation showing 3 cases, all with the load inside or at the kern point, that show adding footing area is increasing my applied bearing pressure. Please tell me what I'm doing wrong here, unless the calculations are correct, in which case I would need some hard convincing that more footing means more pressure.
Thanks in advance,
labeattie
I'm having a frustrating problem that I would think has an easy answer. I'm designing a wall footing for bearing pressure right now, and can only increase the footing dimension in one direction. Imagine my surprise when my calculations say adding a foot in one direction of a footing increases the maximum pressure. I don't really believe this can be true. So I attached a basic calculation showing 3 cases, all with the load inside or at the kern point, that show adding footing area is increasing my applied bearing pressure. Please tell me what I'm doing wrong here, unless the calculations are correct, in which case I would need some hard convincing that more footing means more pressure.
Thanks in advance,
labeattie