Burner2k
Aerospace
- Jun 13, 2015
- 193
Wanted to get some additional information on how to model the following in FEM.
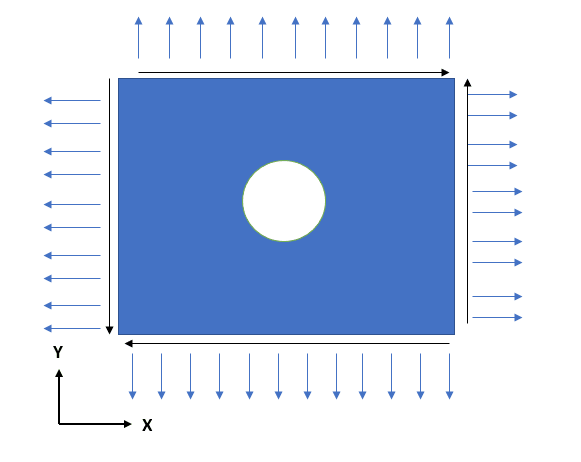
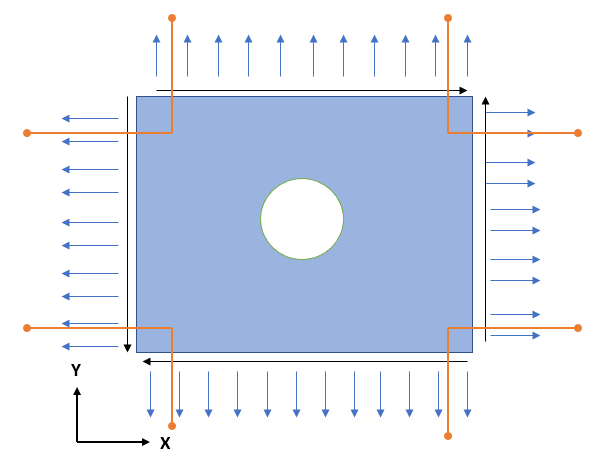
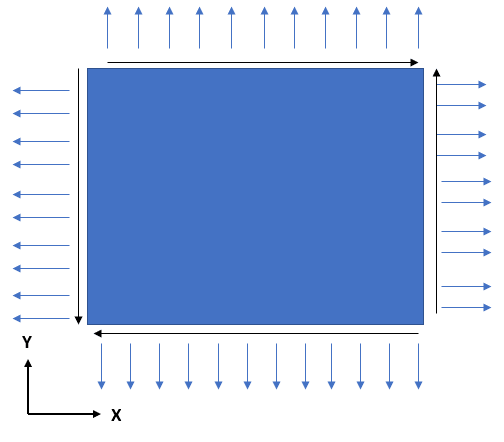
If I want to simulate a plate with biaxial loading with or without shear acting along the edges, what are the different possible boundary conditions which can be specified to capture the deformation pattern accurately (sides of the plate having no curvatures when deformed)?
Thanks...
If I want to simulate a plate with biaxial loading with or without shear acting along the edges, what are the different possible boundary conditions which can be specified to capture the deformation pattern accurately (sides of the plate having no curvatures when deformed)?


Thanks...