AS4100/NZS3404 k_t factor in this situation

[Agent666]

Hi all

A question about the application and determining the k_t factor from table 5.6.3(1) of AS4100 or NZS3404, just something I was wondering if anyone else had come up against this scenario, and how you may have treated it?:-

Brief background to the question, writing a few python scripts to assess some steel code requirements and this kind of thing always gets me thinking about some of the fringe cases to ensure the programming logic treats them appropriately.

This particular case is something I've never really thought of previously. It isn't based on some real situation. Mostly theoretical I guess if this scenario ever came up in real design (possibly continuous crane runway beams is one real world example).


Consider the case of a monosymmetric beam member segment with end restraints combination such that k_t does not equal 1.0, with reversing moment and inflection point somewhere out in the span with no other intermediate restraints. t_f in the two equations is defined as the critical flange thickness. With the reversing moment diagram, both bottom and top flanges are the critical compression flanges at some point in the segments span. So which flange thickness to use in the equations for k_t?

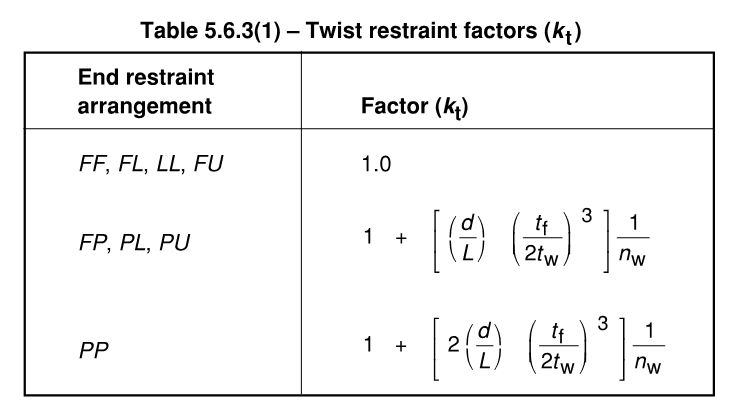
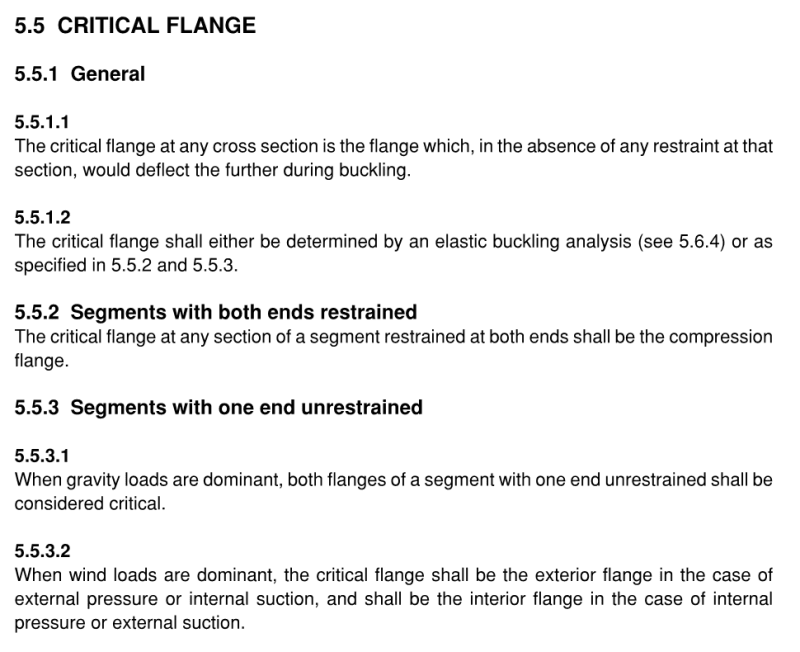
Strictly speaking codes refer to critical flange in section 5.5 as that which deflects furthest, appreciate we could do a buckling analysis for our loading/restraint scenario to get the end answer directly that would take account of the end capacity in this scenario, or at the least confirm which flange is the critical one for the segment based on the greatest deflection criteria. The definition in 5.52 doesn't help us here.

For purposes of my python script I'd probably just take the first point below and use this conservative answer (nice and easy, well aware doing anything else is probably over thinking things). But I could see for cases where most of the segment length has the critical flange being the smaller thickness flange that there must be some benefit that might be able to be taken account of if another method exists without having to do a rational buckling analysis to prove this.

1. Just take the most conservative value based on thickest flange. i.e. k_t is largest for most conservative effective length (L_e).
2. Some other formulation, rule, working from first principles, other known equation, weighted average, etc? Basically I don't know, hence the question!

Don't believe I've ever seen anything discussing this particular aspect, so reaching out for any thoughts/references/etc?

Thanks for any replies!

 

[Settingsun]

I'd have thought it would be the unrestrained flange for cases where that is the same flange at both ends. I think the equation came from analysis of simply supported beams with bottom flanges bolted down to the supports so the critical flange is the unrestrained flange for sagging moment, and the restrained flange (kt=1.0) for hogging.

Beyond that, probably outside the cases that the equation came from so go conservative.

 

[Settingsun]

Thinking more about the case where the restrained flange swaps from end to end, my guess is weighted average based on moment-area, but I have no evidence. We might be able to reason our way to something reasonable by working from the spring-support/flange stiffness fundamental basis.

Thinking yet more, we might need to look at 5.6.1.2 before deciding which is conservative. Run both cases to conclusion then take the smaller capacity.

 

[Agent666]

I hadn't got as far as thinking of the next step in the code process, but the more I think about it due to the 5.6.1.2 clause you brought up and different signs on the monosymmetry section constant, I'm thinking the only way to do it accurately is via the buckling analysis route where one flange isn't the critical flange for the entire segment with a monosymmetric beam. I don't think it's as simple as evaluating worst case for each flange being critical (though may be conservative, but won't know this unless you end up doing the buckling analysis).

I'll have a look at appendix H as may be able to be calculated directly for this condition without resorting to 3rd party buckling analysis.

 

[Agent666]

As an update for anyone that follows, appendix H is a little confusing in its application when it applies to monosymmetric beams, as it still requires k_t for working out L_e (i.e. L_e = k_t * L in applying these provisions). So you are back to my original query about what is this k_t factor is for a monosymmetric beam if both flanges are critical at some point along the beam?!

So my conclusion is that you really need to do a flexural torsional buckling analysis in your software of choice (i.e. one that's capable of doing this). Comparing the results from this case with the reference alpha_m = 1.0 case in your software will allow you to determine alpha_m & M_os & M_ob by applying clause 5.6.4 directly, then you can work out alpha_s using M_oa and hence the design capacity phi_M_bx for whatever loading/restraint conditions you might have on a monosymmetric beam.

 

[Settingsun]

You are probably correct. This is from the Australian AISC (now ASI) 'Design of Portal Frame Buildings'. It doesn't address the kt factor but the conclusion is pretty damning even without looking at the Le calculation in detail. It goes on to propose alternative design rules for monosymmetric crane beams but they only cover simply-supported beams at a quick glance.

 

[Agent666]

I have come across that in the past when doing some work on crane runway beams. Totally agree that unless you have an alpha_m that is in table 5.6.2 then you need to do a buckling analysis to determine the alpha_m for your unique loading diagram. But I am not sure if they are saying the whole procedure including use of M_o eqn with beta_x is flawed or not even if your moment diagram shape is in table 5.6.2.

Someone here in NZ actually did a curve fitting exercise and came up with the following relationship for alpha_m for a segment unrestrained at the end. It's used in some local software for checking steel members. Might be an interesting exercise to see if it is actually in agreement with a buckling analysis. I've looked at it in the past with regard to a few known other moment diagrams and it did a pretty good comparison. But the person who did it may have only used the same known relationships to curve fit the equation, and it may not represent points between that well.