Capping a B31.3 piping jacket

[acollins1]

I'm trying to calculate the required thickness on an end cap for our vessels and I was looking for a way to do this. I've been using ASME sec VIII div I UG-34 for the design of flat heads, but the issue is that this end plate has a concentric pipe that is bored out of it.

The end plate is essentially a large washer that is single fillet welded at the ID of the jacket pipe and the OD of the inner pipe.

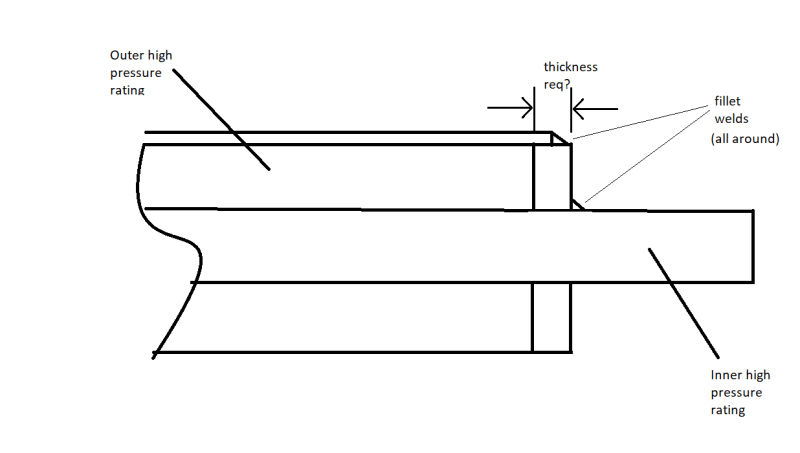
The simple relationship I've found for the minimum end cap thickness is t=d*sqrt(CP/SE) , where d is the end cap diameter, C is the attachment factor from UG-34 (0.33 I'm assuming), P is design pressure, S is allowable stress, and E is joint efficiency (I'm assuming 0.7 for partial RT).

I'm not sure if I can simply use an equivalent diameter relationship to account for the area differential from a full end cap or if I need to go through a more extensive calculation process. Conservatively, not accounting for the inner pipe at all, I get a min thickness of almost an inch, which goes down quite a bit if I use an equivalent diameter or hydraulic diameter instead for variable "d".

Thanks in advance for any advice!

 

[SnTMan]

acollins1, you might review Appendix 9. Consult your Table of Contents.

Pay attention to requirements for weld details.

Regards,

Mike

The problem with sloppy work is that the supply FAR EXCEEDS the demand

 

[KevinNZ]

If the outer and inner pipes are different temperatures then stress the end cap and weld could be very high.

 

[r6155]

@ acollins1
This weld of flat plate is not allowed. See Figure UW-13.2

Regards

 

[LittleInch]

Depending on the sizes, why not use a flange and a blind flange drilled out or a hub flange or a reducing flange.

There are many posts here about drilling and welding pipes through a blind flange.



Remember - More details = better answers
Also: If you get a response it's polite to respond to it.

 

[MJCronin]

Consider terminating the jacketed piping with a "pipe cap" instead of a flat plate.

https://qualitysteamequipment.com/swaged-jacketed-piping/

There are several advantages to this standard configuration using butt welded pipe caps instead of a flat plate

The most significant of which is not relying on the strength of fillet welds loaded in the "weak direction" on the flat plate.

MJCronin
Sr. Process Engineer

 

[XL83NL]

Is this a vessel or a pipe? The title says B31.3 but it’s nowhere referenced in the OP.

Huub
- You never get what you expect, you only get what you inspect.

 

[acollins1]

Thanks for the direction, all things considered each response was very helpful.

Appendix 9 was a huge help. I am now between using a 9/16" washer style flat plate with a double fillet weld at the inner pipe or using a pipe cap bored out for 4" pipe and applying a single weld there. Following the guidance of requirements in B31.3 and ASME Sec VIII I've gone through extensive calcs and determined that either case will work and it primarily comes down to practicality. I'm have a PE in my company review my calcs for accuracy as well just in case.

To answer the other questions: the inner pipe is at cryogenic temperatures and will have internal bellows rated to the pressure. I've accounted for the pressure thrust as well in my calculations. The outer vessel is designed to be at vacuum and contain any potential leaks that may occur, which is why the jacket also has to be rated for high pressure. If the jacket is exposed to high pressures, the bellows thrust force due to pressure will equalize (though I realize the relatively small axial spring force will still be present.) The end plate will never actually see both load cases simultaneously, but I designed as if it would anyway to be conservative.

 

[r6155]

For this service all welds should be full penetration.

Regards