Centrifugal pump Static Pressure

[tomm0005]

Hello, this is the second question in a short time, but hopefully it will be the last one.
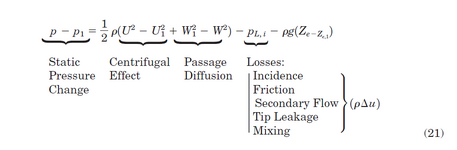
My doubt is related to centrifugal pumps. According to my notes, in a centrifugal pump static pressure rises both in the impeller and in the volute, however, searching on the internet it seems that static pressure only increase in the volute by converting kinetic energy into pressure energy. By analyzing the problem I came to the conclusion that maybe in the impeller both kinetic and pressure energy increase and than further kinetic energy is obtained by slowing down the fluid in the volute, is it correct? In this case, where does pressure energy in the impeller come from? Is it developed by the force exerted by the blades on the fluid?

 

[LittleInch]

T
My way of understanding this, which may be mistaken, is that there is always some internal fluid flow and recirculation within the pump and hence the conversion of kinetic energy to static energy still applies.

Also you need to think about what happens as flow reduces to zero on the outlet. Just because flow goes to zero doesn't mean that pressure suddenly dies when that last 0.01% of flow stops?

The reality is that dead head pressure is the highest pressure you get in most centrifugal pumps. Axial and mixed flow can be different.

 

[goutam_freelance]

The static pressure rises at impellers due to the following effects:
1. Centrifugal effect
The energy imparted to water at the impeller partly increases the water velocity and partly increases the static pressure. This energy increase is reflected in static pressure rise.

2. Passage diffusion
The water's relative velocity with respect to the impeller reduces as the water moves towards the periphery of the impeller. This results in a static pressure rise.

3. Impeller losses
This contributes negatively to the static pressure rise.

3. Static head.
This can be neglected for most pumps.

Of course, the final static pressure rise happens at diffusers by conversion of velocity head at impeller exit to static pressure.

 

[bimr]

The fact is that pumps create flow, not pressure. Pressure is the resistance to flow.

Consider a hydraulic hand pump, when the lever is moved up and down, fluid is removed from a reservoir and sent to the connected equipment through a hose. Suppose the hose from the pump is not connected to the equipment while the handle is cycled. The handle moves easily and the fluid inside the hose moves freely because there is no resistance. Pressure does not increase.

https://www.pumpsandsystems.com/characteristics-centrifugal-pumps

 

[LittleInch]

bimr - the question is what happens when there is no flow? Is there also no pressure?

OPD pumps operate in a different fashion to centrifugal pumps so not sure this is the best example to use.

 

[Snickster]

I believe any pressure developed in the impeller before exiting the periphery of the impeller is transient and does not contribute to the pressure produced at the discharge of the impeller in the volute due to conversion of kinetic energy, of the fluid exiting the impeller, to pressure. I have no references that discuss development of pressure head that contributes to pump differential head that occurs inside the impeller.

I imagine that there is flow friction in the impeller that creates a pressure gradient radially which contributes loss of efficiency. There also would be an increase in pressure due to the force of the impeller on the fluid due to accelerate in the direction of impeller rotation. However, only velocity of fluid at exit of impeller is converted into pressure head otherwise the pump affinity laws would not work.

 

[Compositepro]

It is centripetal acceleration/centrifugal force that creates pressure in a centrifugal pump. Physics tells us that centripetal acceleration is indistinguishable from gravity. If you swing a bucket of water around in a circle, on the end of a chain, the pressure at the bottom of the bucket increases. Then add a second bucket and chain to balance the first bucket. Then add more buckets until the circumference of the circle is filled with buckets touching each other. In principle, it is that simple. If you poke a hole in the bottom of a bucket you get flow. The flow versus pressure argument is just like the question of which came first, the chicken or the egg. It is not very helpful to understanding. They are directly related at all times. You cannot have one without the other.

 

[bimr]

This is what physics tells us. The fluid velocity is at 90 degrees to the centrifugal force:

 

[goutam_freelance]

I have no references that discuss development of pressure head that contributes to pump differential head that occurs inside the impeller.

Refer Pump Handbook by Karassik section 2.1