struggle67
Structural
- Mar 29, 2013
- 116
Hi
I am new to columns or any vertical member design. I only know some of the basic theories.
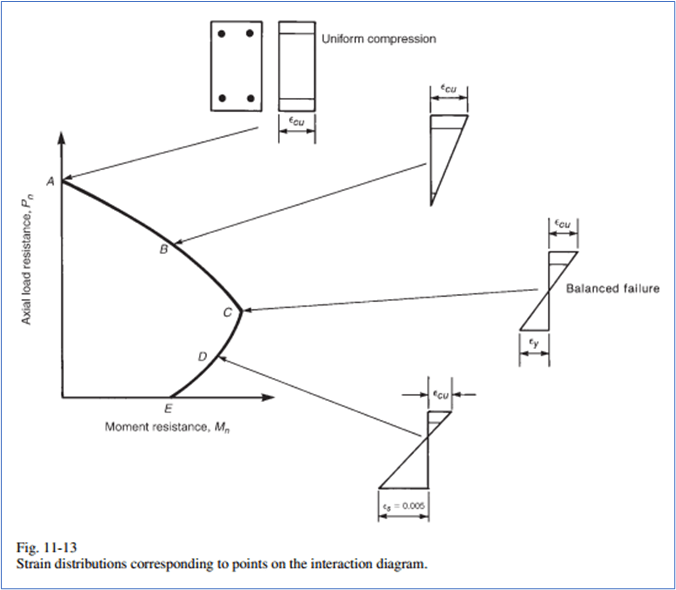
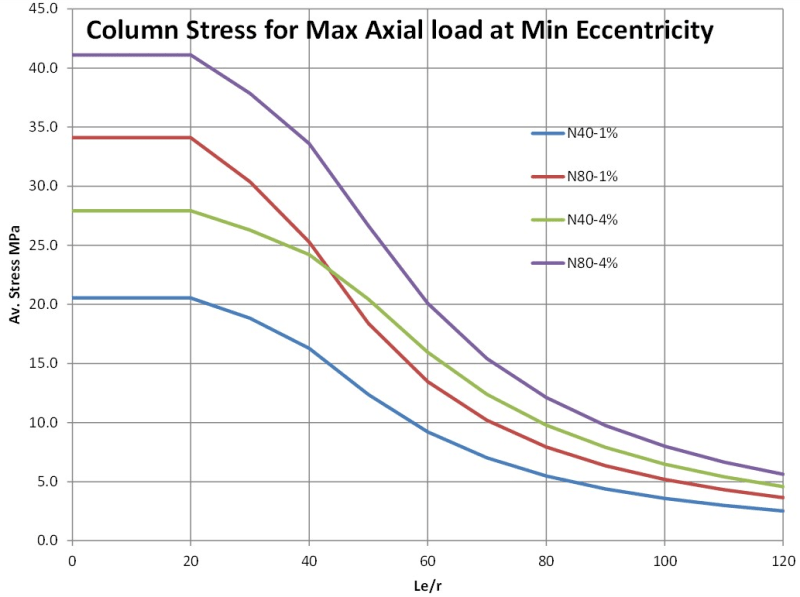
Let’s say I am free to choose whatever column size I want. May I know based on your experience, where is the most economical point (NEd, MEd) on the column-interaction diagram? Reinforcement ratio? 2%? And why?
Thanks, and Best Regards,
I am new to columns or any vertical member design. I only know some of the basic theories.
Let’s say I am free to choose whatever column size I want. May I know based on your experience, where is the most economical point (NEd, MEd) on the column-interaction diagram? Reinforcement ratio? 2%? And why?
Thanks, and Best Regards,