sendithard
Industrial
Sorry for the bad pic, at work, don't have time to open saladworks.
I'm staring at this cylindrical part it has opposing cutouts on both sides and it is a feature of size...+/- .001 on the width size, so you get some bonus. A is the outside cylinder, B is top plane, C is some internal plane for clocking.
I'm not sure you need the Datum B for this callout, but that isn't my question at the moment.
My question is you collapse parallel planes until it hits the highs of these opposing surfaces and you make a midplane out of that. Great...well is the center plane tolerance zone simply the tallest two parallel objects you can fit into these cutouts, or is it 3.14159 mm tall...you get the idea. I don't see a definitive height this centerplane begins and ends at.
Thanks.
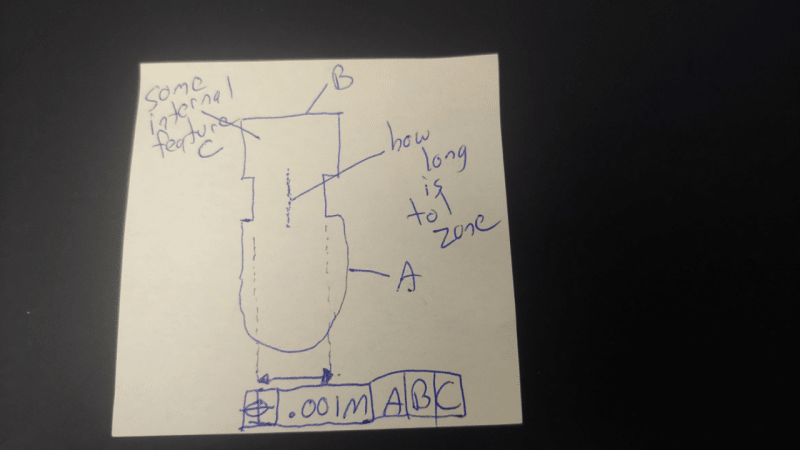
I'm staring at this cylindrical part it has opposing cutouts on both sides and it is a feature of size...+/- .001 on the width size, so you get some bonus. A is the outside cylinder, B is top plane, C is some internal plane for clocking.
I'm not sure you need the Datum B for this callout, but that isn't my question at the moment.
My question is you collapse parallel planes until it hits the highs of these opposing surfaces and you make a midplane out of that. Great...well is the center plane tolerance zone simply the tallest two parallel objects you can fit into these cutouts, or is it 3.14159 mm tall...you get the idea. I don't see a definitive height this centerplane begins and ends at.
Thanks.

