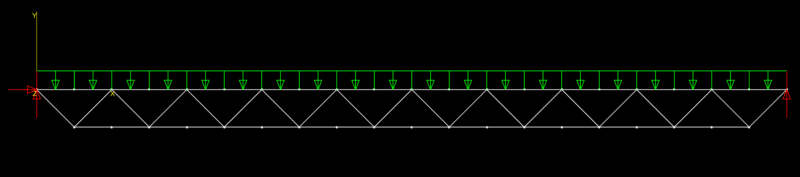
You could probably make a PhD out of this, by the barrel-scraping standards of today. But for design, I'd treat it as a lean-on (or pull-on) buckling problem. Agent666's blog has a post on the subject.
I suspect 271828's method is equivalent but using stresses instead of forces.
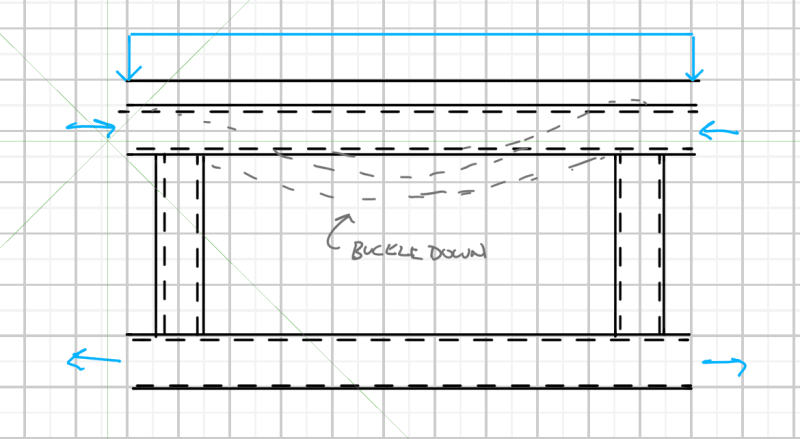
Given the proportions, I don't think the slab necessarily precludes buckling of the chord in the plane of the truss. The slab stiffness is similar to the chord stiffness, not orders of magnitude greater. Stiffness is the first key issue. You need to consider the potential for cracking in the slab's transverse direction due to the loading on the deck, ie cracks that aren't parallel to the truss. If all the chord load were sustained, creep would also come into it, but not if there's a decent reduction from short-term to sustained chord force as the lean-on force will fall away quickly when the chord isn't close to buckling.
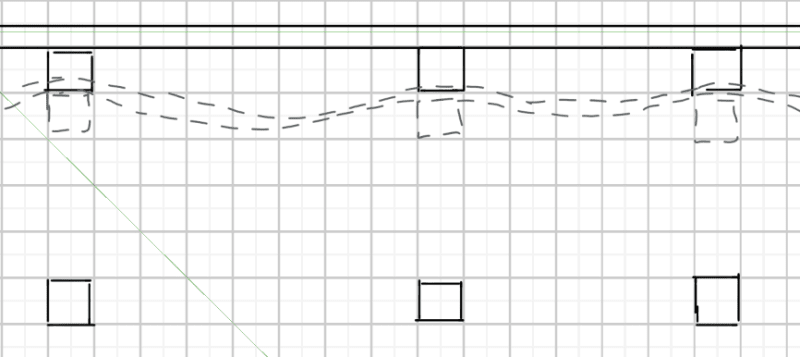
The second issue is making sure the connections between slab and truss are in effective positions. You want some connections midway between the panel points so the chord can pull on the slab if it buckles away, and also connections near the panel points so the slab has a reaction point for the case where the chord buckles towards the slab.
Lastly, you need enough strength and stiffness locally in those connections. I'm picturing the metal deck provides little stiffness in the slab's transverse direction (parallel to the truss) due to thin cross-section without corrugations that way. So you need to be confident the concrete is engaged if the chord is pulling away from the slab, both at the panel points or midway between. Some deck profiles don't give me much confidence of that but maybe there's literature. Also, how far does that thin sheet need to span from a puddle weld to a corrugation?
The good news is that restraint forces are fairly small, so most systems have some chance of functioning as bracing, at least partial bracing (see Agent666's first example).