I-Beam LTB Length at support under hogging moment 2

Hi

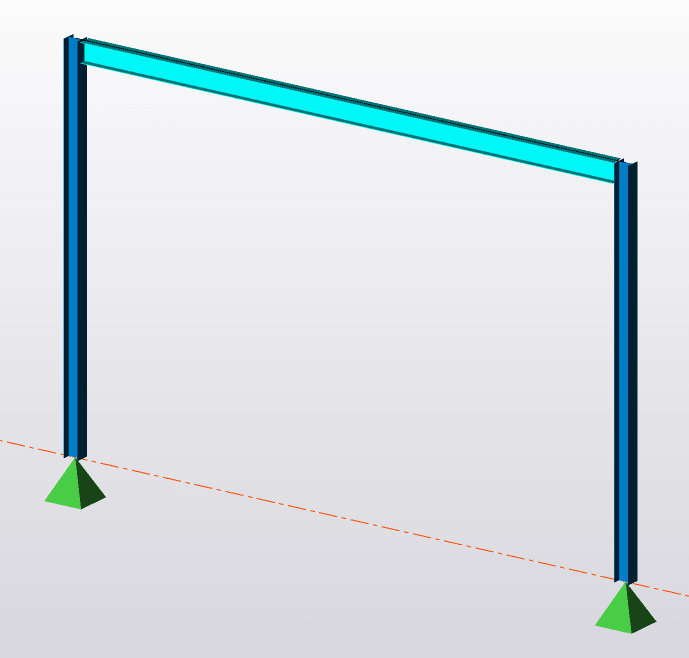
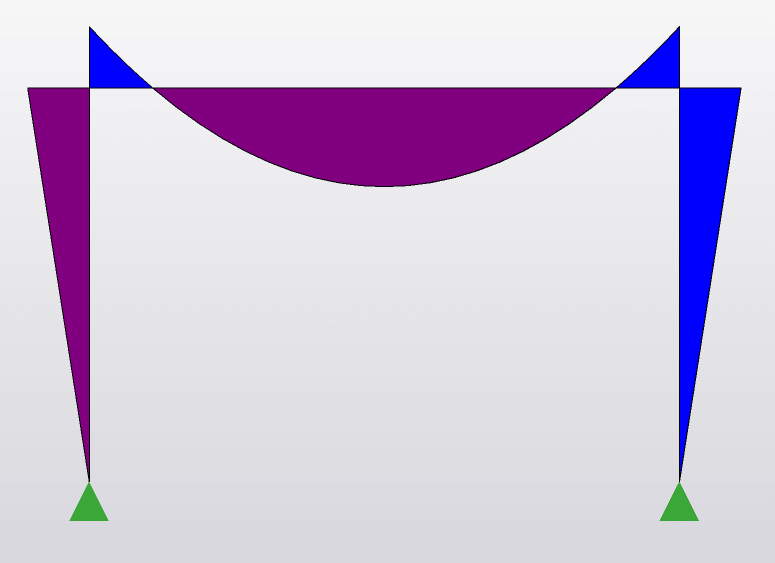
If I have a very simple steel frame with 2 steel columns and one 1 I-beam (UB) and BMD as shown below, how do I decide my beam critical length for LTB resistance at support under hogging moment? My software is using full span length but I was wondering whether it should be shorter as my bottom flange at mid-span will be under tension. Should it be the length from maximum moment to zero moment (point of contraflexure)?

Thanks

 

[Settingsun]

Do you want the long version or the summary?

 

[hetgen]

It's the distance between the columns.

OR
read this thread

https://www.eng-tips.com/viewthread.cfm?qid=459248

 

[struggle67]

steveh49
if summary can help me to decide l_cr logically in the future, I would like a summary

Thanks hetgen

read this thread pc

https://www.eng-tips.com/viewthread.cfm?qid=459248

wow does that thread end somewhere? I am still scrolling

 

[phamENG]

struggle - to boil it down to simplistic terms, LTB is more-or-less caused by the compression flange buckling like a column. When you consider column buckling, what do you use for your length? I realize you can "shorten" it with a K factor for fixity, but keep in mind that the K factor looks at fixity in the direction of buckling. In this case, you likely have a pin in the week axis while your strong axis is fixed. So lateral buckling of the "column" that is your compression flange would have a K = 1.

If you have a diaphragm or discreet braces, you can reduce your l_u. Otherwise, it's the distance between the columns.

 

[KootK]

1) In most practical situations, your beam will have some bracing which will have a large effect on LTB. If you'd like us to consider something other than a completely unbraced beam, you'll have to let us know what you're really working with here. It's very common, for example, to have tightly spaced bracing on the top flange.

2) A long version of the LTB story can be found here: Link. There is some truly excellent information in there if you have the patience for it.

3) To some extent, the answer to your question depends on what steel code you're using (US / Aussie / Eurocode). The approaches taken for LTB in those standards are not always identical.

4) The fact that much of your bottom flange will be in tension will indeed have a massive impact on your LTB capacity, for the better. We studied that explicitly, and numerically, in the thread that I referenced.

5) It sounds as though you are going down the road of wondering whether or not your inflection points can be considered to be LTB bracing. That is certainly not the case in North American practice and you can find an excellent article about that here: Link. Basically, [K] values need to reflect the actual shape that the bottom flange would assume when it buckles laterally as part of the LTB phenomenon. And inflection points do not convert the buckling shape into three half sine waves as one would expect if the inflection points were truly brace points. The bottom flange will still buckle as a single half sine wave from support to support. Some approaches will indeed reduce [K] to account for some of the bottom flange being in tension but that tends to get a bit awkward since, in those situations, [K] is kind of being fudged to account for things other than what it was originally design for (creating an analog between the real buckled shape and a reference pin-pin column buckled shape).

That's about as short as I can make the story without omitting parts of it that I feel are critical.

 

[struggle67]

Thanks, PhamENG KootK

If you'd like us to consider something other than a completely unbraced beam, you'll have to let us know what you're really working with here.

Most of the time I will have some kind of LTB restraints to the top flange either purlins or floor slab.

depends on what steel code you're using (US / Aussie / Eurocode)

I am using Eurocodes.

The fact that much of your bottom flange will be in tension will indeed have a massive impact on your LTB capacity, for the better. We studied that explicitly, and numerically, in the thread that I referenced.

Thanks

The bottom flange will still buckle as a single half sine-wave from support to support. Some approaches will indeed reduce [K] to account for some of the bottom flange being in tension but that tends to get a bit awkward since in those situations

I can imagine the buckle shape from column to column and inflection point not being a LTB restraint but I was just wondering shouldn't the fact that not the entire bottom flange is under compression and part of it is under tension help reduce L_cr?

Seems like either it is K=1 or I have to go through your thread.

Thanks

 

[hetgen]

@struggle67 If you're designing according to the Eurocode check pg 20-27 in the pdf link below, it's a detailed worked example published by SCI (The Steel Construction Institute).

https://www.steelconstruction.info/images/5/50/Sci_p364.pdf

You can download the freeware "LtBeam' used to calculate Mcr from the link below.

https://www.cticm.com/logiciel/ltbeam/

 

[KootK]

I can imagine the buckle shape from column to column and inflection point not being a LTB restraint but I was just wondering shouldn't the fact that not the entire bottom flange is under compression and part of it is under tension help reduce Lcr? Seems like either it is K=1 or I have to go through your thread.

If you look into the Yura document that I posted, you'll see that they account for this benefit via the Cb factor which, at least per US standards, is a more rational way to go about it than is modifying the buckling length to something other than the actual buckling length. That said, I'm sure that you'll want to stick to stuff that jives with the Eurocode.

Based on some detailed work in the thread that I referenced, I've come to the conclusion that it's nearly impossible to LTB a normally proportioned gravity beam of this sort if it has relatively tightly spaced top flange bracing.

 

[struggle67]

Thanks KootK & Hetgen

you'll see that they account for this benefit via the Cb factor which, at least per US standards, is a more rational way to go about it than is modifying the buckling length to something other than the actual buckling length

Yes that makes a lot of sense. I recalled now I think there is one similar factor in Eurocode (C₁).

 

[wrxsti]

this is also a good article on inflection point

https://engineervsheep.com/2019/inflection/

i think the page is run by a forum member here