LTB elastic critical moment - depends on major axis stiffness? 2

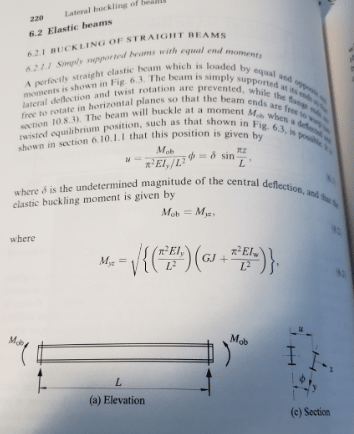
[Settingsun]

I found this equation in a book called "Structural Design from First Principles" by Byfield. I've never come across the (1-Iz/Iy) term in the denominator. Does anyone know another source for this? It would predict that beams don't suffer LTB when bent about minor axis as per usual understanding, but seems a fairly major point to leave out even for major axis bending if it's true.

 

[ClearCalcs]

I've got a few thoughts:

-It's always conservative to ignore this factor (since Iy > Iz by definition)

-Realistically it's not doing much for typical beams. The highest increase in critical moment will be for sections with relatively high weak axis inertia, which means you've already got a high Mcr and your yield strength or deflection is far likelier to govern. These sections would be more suited for columns anyways.

-Going through the entire AISC database (see attached), the maximum possible increase in Mcr is 29%, but that's for the very heavy W14s that you'd only see for columns. Looking at typical beam shapes, say a W12x30, you're only getting a 5% increase in Mcr.

This leads me to believe that code-writers omitted it for simplicity and slight conservatism.

-Laurent

www.ClearCalcs.com

 

[Settingsun]

Hi Laurent, (better late than never)

You're right. I overlooked the square root which makes the effect smaller.

I found another source of the equation which credits Timoshenko and Gere "Theory of Elastic Stability", though I haven't been able to find out in there. It [Timoshenko] mentions the effect and gives some numerical examples which don't quite match this equation but the trend is there.

I still find it odd that this is almost unmentioned in books (if correct) as it shows mathematically that LTB doesn't happen for minor axis bending. Never seen the maths behind it before.

 

[KootK]

I found another source of the equation which credits Timoshenko and Gere "Theory of Elastic Stability", though I haven't been able to find out in there.

Can you point us to that other source that you found? This is of interest to me too. I just scanned my 2nd edition of the Timoshenko reference and couldn't find anything suggesting that the strong axis moment of inertia is involved in the LTB formulation. Perhaps the "credit" was just about Timoshenko's stuff as a starting point which was later rearranged.

I still find it odd that this is almost unmentioned in books (if correct) as it shows mathematically that LTB doesn't happen for minor axis bending.

I don't feel that the equation does show that. Rather, I think that it just shows that LTB for weak axis loading simply happens at a higher load than it does for strong axis buckling. And that's be expected since weak axis LTB involves:

1) Rotating the beam up onto it's strong axis and, therefore;

2) Decreasing vertical deflection under load and, therefore;

3) Increasing potential energy for this component of LTB rather than decreasing it.

How is Iw defined in the original equation? Straight up warping constant?

 

[KootK]

Or are you assuming that, because weak axis buckling would produce a negative number under the square root for this formulation, that means that weak axis LTB isn't possible?

 

[Settingsun]

Here's the other source:

https://files.engineering.com/getfi...Strength_vs_Restraint_Torsional_Stiffness.pdf

I was thinking that the zero in the denominator for equal moments of inertia about both axes would cause the buckling moment to become infinite, and then imaginary for weak axis bending. But maybe I got carried away and it's just outside the limits of applicability of the equation (if it's in fact correct).

The part of Timoshenko that I thought was related is between equations 6-23 & 6-24 in my version where it talks about needing to include deflection in the plane of the web into the differential equations. It doesn't derive the result but gives some example numbers for rectangular cross-section as it becomes more stocky. The example numbers don't quite follow the equation being discussed in this thread though.

 

[Enable]

Thanks to Ingenuity I picked up a copy of The Behavior and Design of Steel Structures to AS4100. See a few excerpts below where it discusses some factors left out in design as well as the exact issue under discussion here. As Steve suggests the limit of Iy approaching Ix ---> Mcr = infinity > Mp and hence does not buckle.

 

[Settingsun]

Thanks Enable. And of course Trahair covers it...

This is from his other book that is focused on buckling:

 

[KootK]

Nice!

I still find it odd that this is almost unmentioned in books (if correct) as it shows mathematically that LTB doesn't happen for minor axis bending. Never seen the maths behind it before.

I agree, this would seem to be exactly that.