Neutral Displacement Current Limiting Reactor

[EddyWirbelstrom]

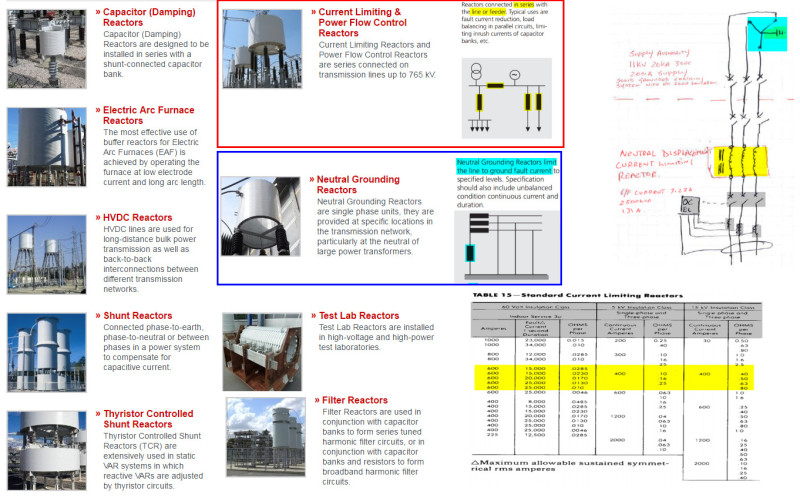
The dataplate of a 3 phase Neutral Displacement Current Limiting Reactor shows:
- Limited earth fault current = 7.22 A
- Throughput kVA = 7.22 A
- System volts = 11000 V
- System amps = 131 A

The 3 phase Neutral Displacement Current Limiting Reactor ( NDCLR ) is intended to reduce earthfault current on a solidly earthed system as shown in attached file.
The NDCLR is intended to be used because voltage drop across an isolating transformer would be unacceptably high.
However the NDCLR would also have a reactance !

What would typical z1, z2 and z0 and x1/r1, x2/r2, xo/ro be ?

Can anyone explain how a NDCLR has negligible positive sequence ( and negative sequence ? ) impedance while having a high zero sequence impedance ?

Assuming z1 = z2 = 0, then Ik1 = ( 3/zo )*Ibase
zo = 3 * Ibase / Ik1 = 54.4321 pu on 2500 kVA base
Zn = ( 54.4321 / 3 )* ( 11000^2 / 2500000 ) = 878.1717 Ohm
Ik1 = ( 11000 / sqrt(3) ) / 878.1717 = 7.231 A

 

[magoo2]

Sounds like a zig-zag grounding transformer. You might want to do a search on that.

 

[cuky2000]

I am familiar with neutral reactor and a series CLR. However, I have to confess that I have difficulty to understand to correlate the diagram with what is described as “Neutral Displacement Current Limiting Reactor”. The diagram appears to be a series CLR.

EddyWirbelstrom, could you please help us to understand better what this is with respect to typical reactor classifications shown below?

 

[EddyWirbelstrom]

The attached file describes the principle of operation of the Current Limiting Neutral Displacement Reactor (CLNDR) as follows:
The three individual phase windings are on one core.
Under balanced three phase operation, the vector sum of positive sequence flux is zero.
The only positive and negative sequence impedance would be due to leakage flux, say 1.0%.
Under single phase to earth fault, the zero sequence flux flows around the air gapped core.
The variable gap is adjusted to vary the reluctance of the zero sequence flux path to limit earth fault current to the desired value.

If the CLNDR limited earth fault current to a known value, then the zero sequence impedance could be calculated as follows :
Ik1 = 3 / ( z1 + z2 + zo )
Assume z1 = z2
zo = 3 / Ik1