Stress linearisation is not required when there is no peak stress

[DriveMeNuts]

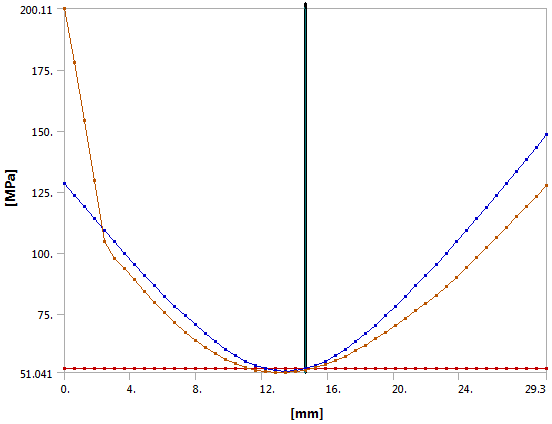
When a vessel shell has a bracket welded to its outside surface which has an external load applied to it, there are membrane, bending and peak stresses on the outside surface of the vessel shell, local to the bracket. Linearization is required to establish the linearized Membrane plus Bending stress at the outer surface. This is shown on the left hand side of the graph below.

My query relates to the inside surface of the vessel shell where there is no structural discontinuity and therefore no peak stress as per the right hand side of the graph. To calculate the Membrane plus bending stress on the inside surface do you re-use the linearized stress tensor components used for the outside surface and calculate a linearized M+B stress for the inside surface (the blue line)? Or do you accept that there is no peak stress on the inside surface and therefore just use the total stress (the brown line)?

 

[BJI]

Linearization will give you a membrane stress, and a bending stress at the inside and outside surface. Given that there is no peak stress on the inside, you would expect the linearized membrane plus bending to closely match the total stress, unless the mesh is relatively coarse. If you refine the mesh around the peak location you should see this influence drop out of your linearized result.

 

[DriveMeNuts]

The peak stress associated with the structural discontinuity causes the individual linearized membrane and bending stress components to be higher or steeper respectively. As a result, when calculating the equivalent stress on the right hand side, the linearized value is always greater than the total value.
It is due to the maths of linearization. Mesh density doesn't affect it.
As this example is completely in bending, both sides should have the same value. As the total value on the right is the same as the linearized value on the left, it seems reasonable to say that linearization is not required on the side with no structural discontinuity.....for this specific example.
I was wondering if anyone else has experience with this or knows the industry standard convention.

 

[TGS4]

Just follow the rules and perform the linearization. It will give you the correct answers. Stop trying to over-think it.

 

[DriveMeNuts]

Upon carrying out further investigation, WRC 429 accepts the linearization method of judging "Linearity of the distribution of stresses" which is essentially what I have explained above, however recommends the use of non-judgement mathematical approach like the through thickness integration method used by ASME VIII Div 2....even if it is conservative.