aSperling
Structural
- May 4, 2016
- 5
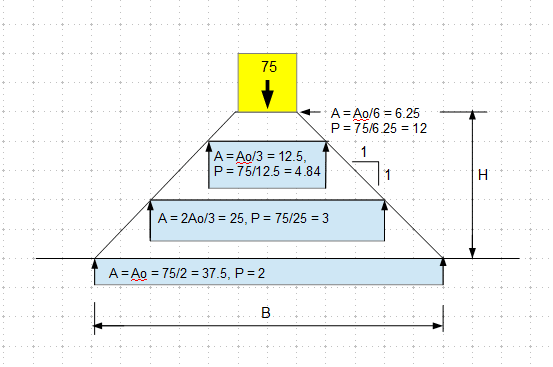
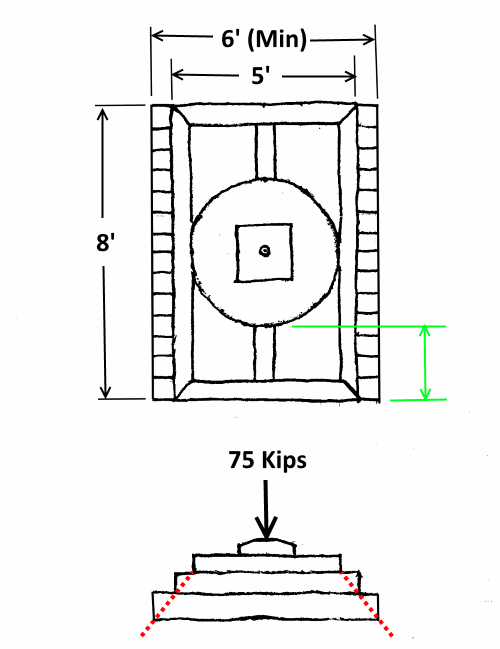
I am working on a lift plan that involves outrigger loads being applied to existing foundations. My max. outrigger load is 75kips and the original building Engineer on Record has indicated that the maximum applied UDL is 2000 psf which is governed by subgrade bearing pressure. Given this I want to distribute the point load over a 5'x8' area. The EOR has indicated that I am to provide calc's to prove that the UDL from the crane outrigger is distributed over the area required.
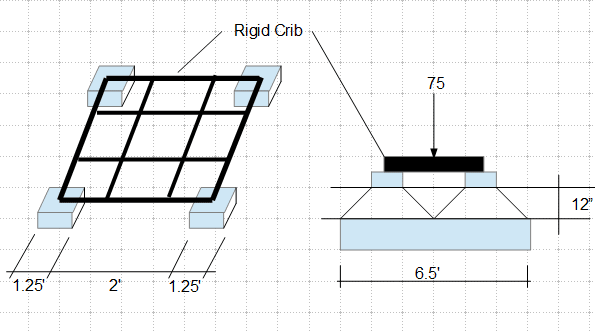
I have a plan on how I want to do it. From top down I would use the 18" outrigger shoe on a 4' diameter aluminum outrigger pad (honeycomb design, 6" deep section). The outrigger pad would be placed on 4' wide row of 8' long railway ties. The ties would be placed on steel rig mats on the concrete floor.
My intuition tells me this would do an adequate job of spreading that point load out; but how does one go about proving this numerically?
Since the 12" concrete structural slab would be stiffest element can I even count on the 7" thick railway ties assisting in load distribution? Would the concrete slab not need to deflect in order for the load to spread out thru the wood layer? The wood/concrete comparison is the most extreme difference in stiffness; but I am wondering what the principal is that could be used to look at the aluminum/steel layers as well.
Thanks in advance for any guidance on this.
I have a plan on how I want to do it. From top down I would use the 18" outrigger shoe on a 4' diameter aluminum outrigger pad (honeycomb design, 6" deep section). The outrigger pad would be placed on 4' wide row of 8' long railway ties. The ties would be placed on steel rig mats on the concrete floor.
My intuition tells me this would do an adequate job of spreading that point load out; but how does one go about proving this numerically?
Since the 12" concrete structural slab would be stiffest element can I even count on the 7" thick railway ties assisting in load distribution? Would the concrete slab not need to deflect in order for the load to spread out thru the wood layer? The wood/concrete comparison is the most extreme difference in stiffness; but I am wondering what the principal is that could be used to look at the aluminum/steel layers as well.
Thanks in advance for any guidance on this.


![[idea] [idea] [idea]](/data/assets/smilies/idea.gif)