VFD Effects on Motor Heat Output 1

[Quiny96]

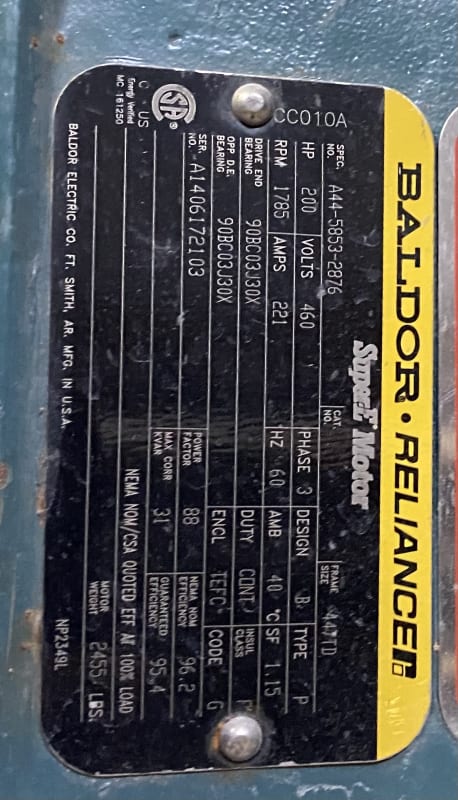
To find average heat losses on a motor, it can be quickly determined using the rated HP and efficiency.

EX. A 200 HP @1800 RPM and 95% eff.
200 HP x (1-.95) = 10 HP
10 HP x 2550 = 25,500 BTU/hr
(25,500 BTU/hr) / (12,000 BTU/hr) = 2.125 Tons

But what if the motor is run off a VFD? What factors must be taken into account when determining heat loss?
VFDs can run motors at far under their rated RPM, does that make the efficiency and resulting heat output of the motor very high as well?
Unless I am mistaken, VFDs can run motors at constant Torque while fluctuating HP input. Which leads me to the following conclusion...

EX. Lets say the same motor from above is ran off a VFD at 1000 RPM
Could the equation HP = (T x N)/5252 be used with constant torque to find the HP loss and convert it to Heat output?
Where T is torque and N is RPM

For instance at rated speed:
T1 = (HP1 x 5252) / N
T1 = (200 HP x 5252) / 1800 RPM = 589 ftLbs

If Torque is constant with VFD, then T1 = T2, therefore:
HP2 = (T1 x N2) / 5252
HP2 = (589 ftLbs x 1000 RPM) / 5252 = 82.9 HP

To find losses: 200HP-82.9HP = 117HP = 24.88 Tons

Does this train of thought work, or am I missing the bigger picture of how a VFD effects the motors efficiency and heat output?

 

[electricpete]

Your approach doesn't look right to me.
> To find losses: 200HP-82.9P =...
Why are you considering 200HP. It's just a rated mechanical output, it's not relevant to any other operating condition.

Maybe someone else can give you an outline of how to estimate things (I'd have to collect my thoughts and I think I'd overcomplicate things like I did in my first draft). Do you have a motor nameplate or data sheet?


=====================================
(2B)+(2B)' ?

 

[Compositepro]

One ton of refrigeration is 12,000 btu/hr.

The error appears to be that a VFD is used to slow a motor from 1800 to 1000 RPM, which results in a a reduction in mechanical hp from 200 to 82.9. which results in the power going to heat.

Ah, now that Pete has pointed it out the error is that the OP thinks an electric motor rated at 200 hp always outputs 200 hp. This is a common misconception.

Quiny, there are many types of electric motors. The most common is an induction motor. Its speed is determined by the powerline frequency. If there is not mechanical load on the motor it will turn at slightly less than 1800 rpm on 60 cycles, and use almost no power. As mechanical load is put onto the motor it slows downs and will draw more power to try to maintain speed. It will continue to do this up to its rating of 200 hp. As the motor odraws more power, it gets hotter. Above 200 hp, the motor will over-heat. At 200 HP it will be turning at about 1750 rpm. A vfd (variable frequency drive) converts a standard constant speed induction motor into a variable speed motor.

You will generally see 1750 rpm on the name plate of the motor. Only synchronous motors actually turn at 1800 rpm. Go learn-up about electric motors. It will seem overwhelming at first but you just have to remember that they are just devices designed to produce mechanical power from magnetic forces created by electricity.

 

[waross]

First, your basic losses depend on current, I[sup]2[/sup]R.
At full load current, the I[sup]2[/sup]R at the basic frequency in Watts will be relatively constant.
But, Constant current implies constant torque. As the speed and frequency drop, the HP drops.
As a result as the speed drops and the HP drops the constant loss becomes an increasingly greater percentage of the HP.
There are some second order effects. There will be increased current at a given frequency due to distortion power factor caused by harmonics excited by the PWM switching.
My thoughts are that the distortion PF current will be at 90 degrees to the real current and may have little effect on the resultant current.
I am somehow reluctant to equate motor losses to tons of melting ice, but that's just me. Whatever cools your beer.
Back to you Pete. Do you have any thoughts about Watt losses caused the distortion power factor component of the current?

Bill
--------------------
Ohm's law
Not just a good idea;
It's the LAW!

 

[Quiny96]

Pete,

Here is the nameplate for the motor.

My first example was worked using roughly the info on the nameplate. The train of thought is that if at a full load of 200 HP the efficiency is rated for 95.4%, then an inequality can be formed to determine the losses (assuming they are all heat) and converting it to the tonnage required to cool the motor.

My thoughts are that the resulting tonnage of 2.125 Tons of heat output seems far too low, leading me to believe the VFD running the motor may be playing a factor to increase the losses of the motor.

In addition, the VFD runs the motor between 740 RPM and 1450 RPM. I am unsure how to determine the efficiency of the motor with the additional variables of the VFD and lowered RPM.

Thank you everyone for all the helpful replies and food for thought.

 

[waross]

1785 RPM is 15 RPM slip. That is a slip frequency of 0.5 Hz. The rotor will see 0.5 Hz at full torque across the speed range.
So rotor losses will not change.
Torque is related to current and so at rated current the stator losses will be the same.
That is the losses expressed as Watts or tons of melting ice. The losses expressed as a percentage, roughly inversely related to speed.


Bill
--------------------
Ohm's law
Not just a good idea;
It's the LAW!

 

[EdStainless]

Running this motor on a VFD at 1785rpm will have higher losses (and be a bit hotter) than running it on line.
As Waross said this due to waveform issues (not sin wave and harmonics).
These loses get worse as the frequency varies from 60Hz.
In VFD rated motors there is usually an eff vs freq curve that you can look at.

= = = = = = = = = = = = = = = = = = = =
P.E. Metallurgy, consulting work welcomed

 

[jraef]

Evidence to the fact that efficiency of the motor is worse with a VFD is that motor mfrs who rate their motors to be used with VFDs, will tell you that a motor with a 1.15 Service Factor will have only a 1.0 SF if run from an inverter. In other words you CANNOT run a motor into the SF when using a VFD, because you are ALREADY consuming the "fudge factor" of thermal capacity that is involved in the 1.15SF rating. You could therefor infer that the motor losses are roughly 15% higher if the motor is run from an inverter.

I've seen a report on motor efficiency when using VFDs, I think put out by the US DOE "Energy Matters" program. I'll try to find it.


" We are all here on earth to help others; what on earth the others are here for I don't know." -- W. H. Auden

 

[waross]

When looking at losses of variable speed motors at different speeds, be sure to recognized the difference between per-unit losses and losses expressed in Watts.

Bill
--------------------
Ohm's law
Not just a good idea;
It's the LAW!