I am working as an intern on a kinematics exhibit for the Oregon Museum of Science and Industry and need to create a pair of elliptical gears. I am having trouble getting two ellipses (with or without teeth) to stay tangent to each other throughout a full revolution when mounted on fixed centers (not foci). Is there a standard formula for the ellipse itself? Is it possible that it isnt an ellipse at all?(a spline maybe)I am using mastercam and solidworks to create the geometry and toolpaths.
Eng-Tips is the largest engineering community on the Internet
Intelligent Work Forums for Engineering Professionals
-
Congratulations waross on being selected by the Eng-Tips community for having the most helpful posts in the forums last week. Way to Go!
Creation of geometry for elliptical gears 7
- Thread starter skunkmilk
- Start date
-
1
- #2
Look around this site and possibly give them a call as they will probably give you some help for a museum project.
-
1
- #3
EnglishMuffin
Mechanical
Assuming you are allowing one gear to drive the other, the ellipses are the same size, and are initially arranged such that their major axes are orthogonal to each other, with the major axis of one ellipse intersecting the center of the other, I can't see your problem. But for some arrangement other than that, there might be one.
See for example :
But I must confess I have no clue what a theoretically correct tooth form would be like, or how it might be easily generated with ordinary machinery, other than edm or stereolithography. Most probably, for many purposes you could get by with an involute approximation.
See for example :
But I must confess I have no clue what a theoretically correct tooth form would be like, or how it might be easily generated with ordinary machinery, other than edm or stereolithography. Most probably, for many purposes you could get by with an involute approximation.
-
1
- #4
Hi skunkmilk,
I picked this up over at:
"A book that talks about non-circular gears. Gear Geometry and Applied Theory by Faydor L. Litvin, 1994, published by Prentice Hall.
The evolute for non-circular gears is dependent upon the circumference of the gear. Left and right sides of the tooth may have different evolutes. These could be generated, but I may just do an approximation to save on the integrals. The evolute for a circle of corresponding curvature radius at point on the gear where the tooth is to be added, can be used to approximate the actual evolute."
I met some of the old original gear heads in Chicago several years back. They used to get together and play poker, and my gear vendor took me by to meet a few of them. Some of them had worked out the math for the volute pattern for oval and other odd gear jobs by hand.
I picked this up over at:
"A book that talks about non-circular gears. Gear Geometry and Applied Theory by Faydor L. Litvin, 1994, published by Prentice Hall.
The evolute for non-circular gears is dependent upon the circumference of the gear. Left and right sides of the tooth may have different evolutes. These could be generated, but I may just do an approximation to save on the integrals. The evolute for a circle of corresponding curvature radius at point on the gear where the tooth is to be added, can be used to approximate the actual evolute."
I met some of the old original gear heads in Chicago several years back. They used to get together and play poker, and my gear vendor took me by to meet a few of them. Some of them had worked out the math for the volute pattern for oval and other odd gear jobs by hand.
-
1
- #5
Skunkmilk has discovered the 'eliptical gear sets' are not truly eliptical. Take note of the text in the graphic on the cunningham links above - Eliptical Bilobes. If you model elipses with a very large major axis and a very small minor axis and roll them together on a fixed center distance, you should be able to visualize the lobes.
-
1
- #6
Check out "Mechanisms and Mechanical Devices Sourcebook" by Chironis and Sclater
It has a section on "noncircular gears"
Some gear types are:
1. Two ellipses rotating about a foci
2. 2nd order elliptical gears rotating about their geometric centers
3. Eccentric circular gear rotating with its conjugate
Googling some of the gear types might be useful.
It has a section on "noncircular gears"
Some gear types are:
1. Two ellipses rotating about a foci
2. 2nd order elliptical gears rotating about their geometric centers
3. Eccentric circular gear rotating with its conjugate
Googling some of the gear types might be useful.
-
2
- #7
Another example from an excellent book, Mechanisms, Linkages and Mechanical Controls - McGraw-Hill, 1965.
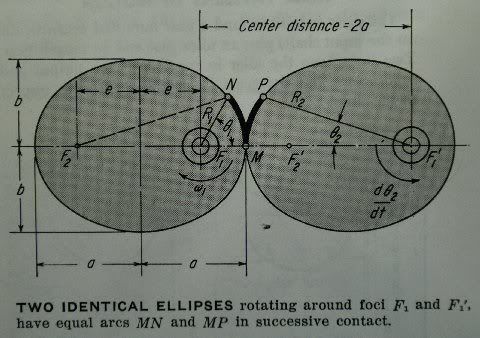
Other variants of Noncircular Gears Systems from the same book at this URL, it's a large picture, so I've put it here.
Try this site as well, there is a description of how the tooth profiles were found for each radius, and how they were cut.
Other variants of Noncircular Gears Systems from the same book at this URL, it's a large picture, so I've put it here.
Try this site as well, there is a description of how the tooth profiles were found for each radius, and how they were cut.
As Nicholas P. Chironis edited the 1965 book that I referred to, I'm not surprised that some parts of that earlier work are used in the book that is available at Amazon, for a reduced price of $72.78.
The 1965 book is virtually all mechanically based, copies of it can be bought at abebooks.com, starting at $30.00
- Status
Similar threads
- Locked
- Question
- Locked
- Question
- Locked
- Question