Continue to Site

Eng-Tips is the largest engineering community on the Internet

Intelligent Work Forums for Engineering Professionals

Register Log in
  • Congratulations KootK on being selected by the Eng-Tips community for having the most helpful posts in the forums last week. Way to Go!

Development Length, Transverse Reinforcement Index Clarification

Status
Not open for further replies.
Quade999

Quade999

Civil/Environmental
May 29, 2020
61
Hi Everyone,

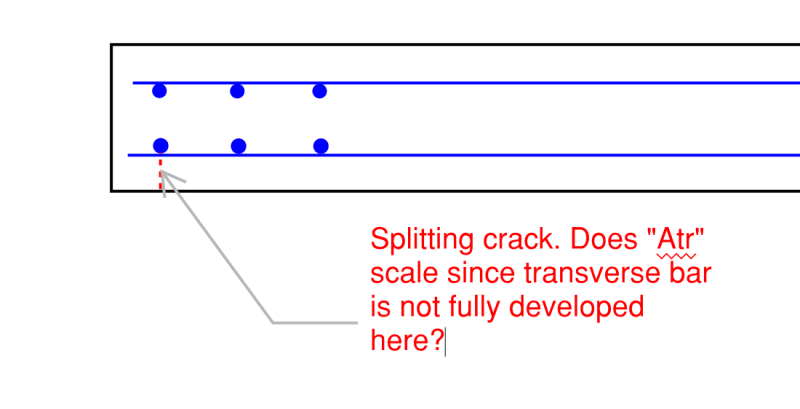
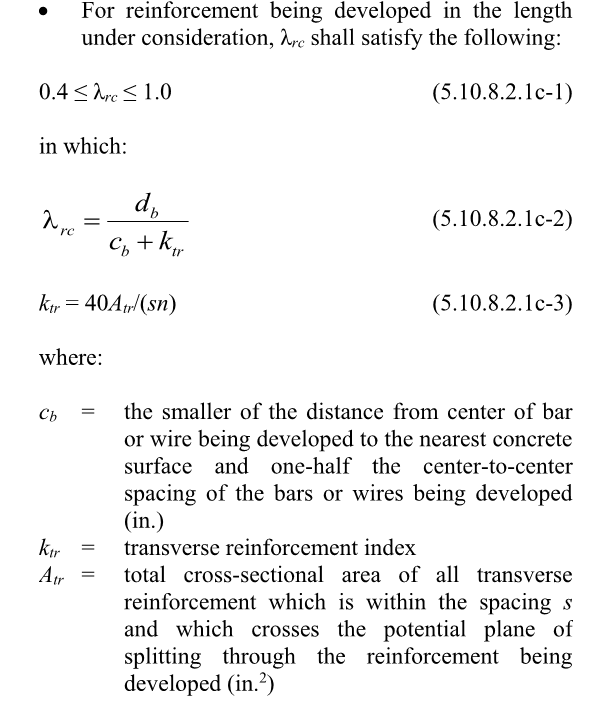
When calculating the development length of a straight bar you are allowed to take into account the transverse reinforcement index which gives a benefit when transverse reinforcement crosses the splitting crack location. However, AASHTO or ACI does not clarify if the area of transverse reinforcement crossing the crack location should be scaled downwards if it is not fully developed at this location. Take for instance, a slab as shown below, where I am determining the development length of the bar at the edge, and the crack crosses a transverse bar that is not fully developed. When calculating Atr, can I use the full area of the transverse bar, or does it have to scale? Since the transverse reinforcement index calculation doesn't take into account yield strength I would assume you can use the full area, as the calculation would provide the same results for 60ksi or 100ksi yield strength.

1_ibzgoe.png

2_on9kr5.png

3_y58yny.png

4_t9h24o.png


Thanks
 
Replies continue below

Recommended for you

Sort by date Sort by votes
In your example of a bottom layer of bars I wouldn't consider the straight bottom layer bars to count at all for this benefit to be taken advantage of. Nor do I believe you can account for partial development.

My interpretation is that transverse reinforcement is confining reinforcement, with legs returning into the concrete which by definition is fully developed (like assumed for a stirrup or tie), A_tr is the area of reinforcement crossing the split, for example this is what your example 3 is saying to illustrate my point regarding your bars not being effective at all. 4 stirrup legs cross the potential split in example 3, if the bottom bar carried through then zero bars anchored into the concrete core cross this potential plane of splitting (n=0).

 
Upvote 0 Downvote
I don't think that it would need to be a stirrup to count as transverse reinforcement. It specifies transverse bars (Doesn't specify that the transverse bar has to be a stirrup). In the definition of Abtr it says the area of an individual transverse bar crossing the plane of splitting (a bar placed 90 degrees to the longitudinal reinforcement would be called a transverse bar). If a crack plane crosses a straight transverse bar, I believe that bar would try to resist that crack provided it is fully developed on each side.
Also, Canadian code used the same equation but is just converted to Metric and it states that the Atr is area of reinforcement that crosses potential splitting crack.
5_qz84kl.png
 
Upvote 0 Downvote
So in your case what bars cross a straight horizontal split like example 3. Nothing right? Thats the point I was trying to make, that you need bars crossing the potential splits between bars. You don't have it despite having transverse reinforcement. You have to investigate all possible splitting arrangements.
 
Upvote 0 Downvote
Without a support at the slab edge, the transverse bras seem lightly stressed, thus the crack under the longitudinal bar is unlikely to occur. Also, we have to think the collective strength, rather than one or two bars. I've no doubt that the equations were developed through numerous experiments and studies for quite broad situations, including the one you are addressing, unless you have the study of your own indicating otherwise.
 
Upvote 0 Downvote
I apologize. I misunderstood your comment and didn't do a well enough job of explaining. I was only looking at the situation where the splitting crack goes from the center of the bar to the nearest concrete surface (bottom of slab). The assumption I intended to make was that the distance between adjacent bars is very large and thus not a governing case (I completely agree that splitting between bars has zero benefit from transverse bars). Basically, what I was saying was that in my slab drawing, Case 1 would have Atr = 1 transverse bar, and Case 2 or Case 3 would have Atr = 0.
 
Upvote 0 Downvote
Status
Not open for further replies.

Similar threads

struct_eeyore
  • Question
min. development length
Replies
5
Views
2K
EngDM
EngDM
IreStruEng
  • Question
Development Length Question
Replies
5
Views
4K
KootK
KootK
slickdeals
  • Locked
  • Question
Development length schedules
Replies
6
Views
221
bugbus
bugbus
ENGJP22
  • Locked
  • Question
Development Length in Seismic Regions
Replies
1
Views
156
struct_eeyore
struct_eeyore
ChickenBake
  • Locked
  • Question
Understanding Development Length 1
Replies
17
Views
414
Celt83
Celt83

Part and Inventory Search

Sponsor

Back
Top Bottom