Hi,
I need to calculate the Bending Force required to bend a reinforced bar bundle.
The bundled bar is in 12M in length with each individual bar having a diameter of 32mm having 15 Bars in the bundle which weighs approx 1136 kg. The yield strength is 650 MPa.
How to calculate the bending force when it's a bundle and not a single bar
Need some help formulating and calculating it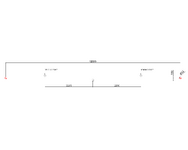
I need to calculate the Bending Force required to bend a reinforced bar bundle.
The bundled bar is in 12M in length with each individual bar having a diameter of 32mm having 15 Bars in the bundle which weighs approx 1136 kg. The yield strength is 650 MPa.
How to calculate the bending force when it's a bundle and not a single bar
Need some help formulating and calculating it

