Calculating Resistance and Reactance from positive sequence fault current 1

[asela115]

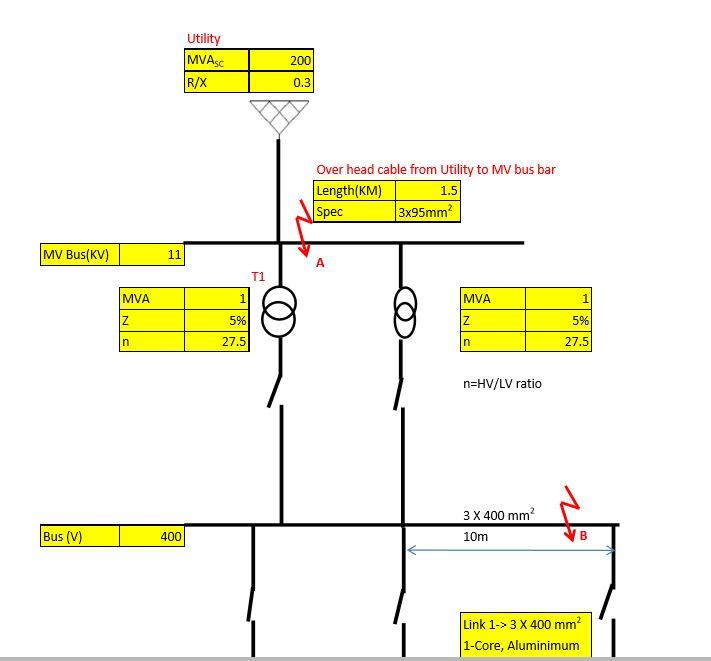
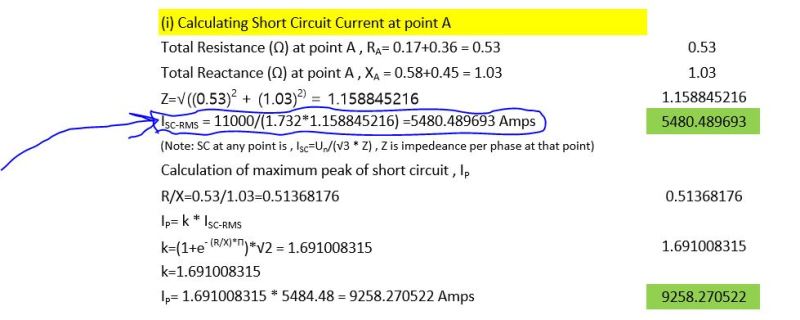
Dear All,

I know following two parameters

a) Maximum three phase fault current in amperes (A) and phase angle in degrees (B).

I want to calculate the positive sequence resistance (R) and reactance (X) from above two variables for a symmetric system. I couldn't figure out a way to find R and X values using just these two parameters. However I noted somebody has calculated this using following formula for same purpose (unfortunately couldn’t verify who did this in my workplace).

R = A * COS (PI()*B/180) X = A * SIN (PI()*B/180) where A is the three phase fault current and B is the phase angle

If someone can guide me to figure out the correct formula and the relation above, please

Thanks

 

[waross]

A word picture;
Construct a right triangle with hypotenuse = A (Amperes)
and
Angle = B
Hypotenuse = current = 1/Z = (1/Impedance)
Base = Real Current component = 1/R = (1/Resistance)
Altitude = Reactive Current component = 1/X = (1/Reactance)
You should be able to solve this with reciprocals, sines and cosines.

R = A * COS (PI()*B/180) X = A * SIN (PI()*B/180) where A is the three phase fault current and B is the phase angle

Is this the formula when the angle is reported in radians?

Bill
--------------------
"Why not the best?"
Jimmy Carter

 

[ZeroSeq]

Type this into google:

symmetrical components filetype:xlsx

There are a few good excel calculators out there that are very simple.

 

[asela115]

Hi Waross,

thanks for the explanation
is it like , 1/Z = (current / voltage)
(do i need to have voltage as well?)

 

[7anoter4]

In my opinion, if you intend to calculate the impedance in ohm-not in p.u.-then you’ll need the voltage.
A=V/(R+Xi)=V(R-Xi)/(R^2+X^2) then:
Iactiv=V*R/(R^2+X^2) Ireact=-V*X/(R^2+X^2) TAN(B)=Ireact/Iact=-X/R
A=SQRT(Iactiv^2+ Ireact^2)
A=V*SQRT(R^2+X^2)/(R^2+X^2)=V/SQRT(R^2+X^2)
X=TAN(B)*R
Then:
A=V/R/SQRT(1+TAN(B)^2) and from here:
R=V/(A*SQRT(1+TAN(B)^2)
(1+TAN(B)^2)=1+SIN^2(B)/COS^2(B)=1/COS^2(B)
R=V/A*COS(B) X=V/A*SIN(B)
B has to be radians then if B it is done in degrees then B[rad]=pi()/180*B[degrees].

 

[jghrist]

I agree with 7anoter4 as long as you use phase-neutral voltage for V.

 

[7anoter4]

Thank you, jghrist and sorry for the delay.
You are right. Since this is a symmetric 3 phases system [direct sequence system] the rated voltage has to be phase-to-phase voltage. Then the involved voltage here has to be V/sqrt(3).

 

[dogzzz]

Hi !!
I will explain in a reverse way , by taking an example . Just ignore the material which is not relevant to you .
Suppose you have to calculate the 3 phase short circuit at point A as shown below .

As shown in blue is how we calculated the short circuit current , but in your case you already know the SC current , thus
Z = Un/(1.732*Isc).
Once you have Z , then you can calculate , R/Z=Cos(theta)
As you said you already have theta.

I hope this will help.