Calculating Shear Stress of 4130 Steel Tubing 3

[JackRu]

Hi all,
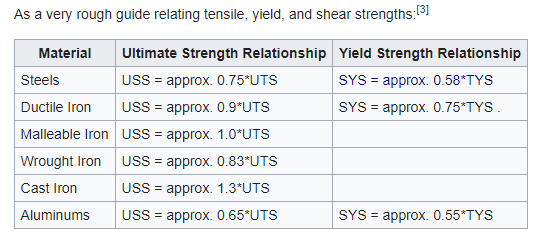
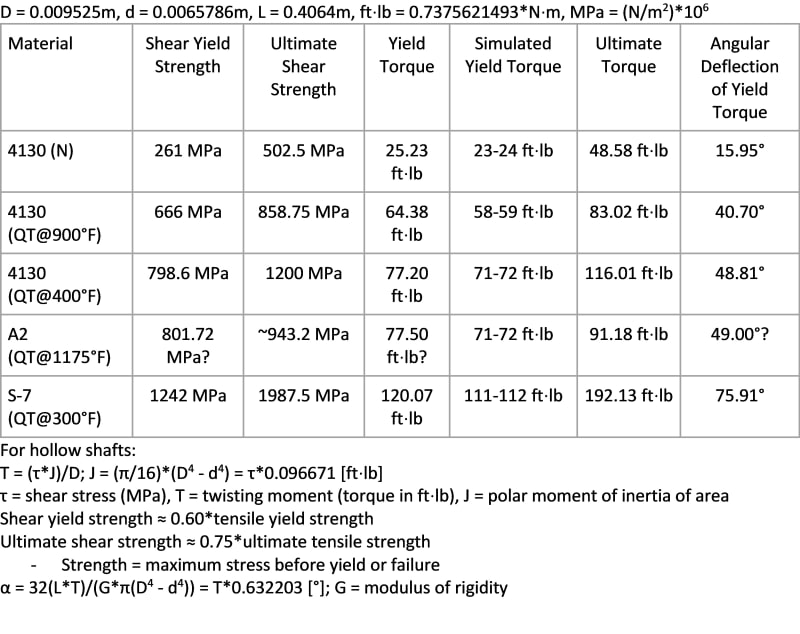
Do you know how I might go about calculating the maximum shear stress of a 16"-long, 0.375" OD x 0.259" ID Q&T 4130 tube? It has a yield strength of 1110MPa and ultimate tensile strength of 1145MPa. I am trying to calculate the maximum permissible torque that can be applied before yielding. If I approximate the shear stress as (1/sqrt(3)) * ultimate tensile strength, then the max torque comes out to 64ft-lbs. A SolidWorks simulation gives 58-59ft-lbs as the correct figure.

Thanks,
Jack

 

[r13]

Use the formula "T[sub]max[/sub] = (π/16)τ[sub]max[/sub](D[sup]4[/sup] - d[sup]4[/sup])/D"

 

[STrctPono]

A few comments...

You say that you are "trying to calculate the maximum permissible torque that can be applied before yielding" but you are multiplying the allowable von mises stress criterion by the ultimate tensile strength. These two statements do not go hand in hand and perhaps you can recheck which of these statements is true. Are you performing a linear analysis under static loads? Is the 58-59 ft-lbs of torque from SolidWorks a force demand or a capacity? Your yield strength of the material is 97% of the ultimate tensile strength!? Are you sure?

If you're following AISC allowables (which I'm guessing you're not) then we call the allowable stress your critical stress and can be as high as 0.60Fy (~1/sqrt(3)) but should be taken as the higher of the following:

Fcr = (1.23E)/[(sqrt(L/D))*((D/t)^(5/4))]
or
Fcr = (0.60E)/[(D/t)^(3/2)]

Tn = FcrC

L = length of member
D = outside diameter of tube
C = Torsional Constant of section

A strength reduction factor is then applied to this based on the combination of your loads.

 

[JackRu]

retired13,
This is correct, I am using the formula Tmax = (π/16)τmax(D4 - d4)/D, but do not know the value of either Tmax or τmax. Do you know how I might go about calculating τmax given the dimensions of my pipe and its material? It is essentially a part of a hollow auger that is hand-screwed into living wood.

 

[JackRu]

STrctPono,
I come from an electrical engineering background, so unfortunately I am at a pretty basic level when it comes to mechanical engineering. Could you explain how to calculate the max permissible torque the pipe can withstand without permanent distortion and/or breakage? I understand that the shear strength is typically estimated based on a material's ultimate tensile strength? I believe I am doing a linear analysis under static loads (although the pipe is allowed to have an angle of deflection). Also, I'm not too familiar the difference between force demand and capacity or with shorthand such as Fy, Fcr, E, and Tn. All of the spec sheets for 4130 steel show the yield and tensile strengths to be within a close range (ex:

http://www.matweb.com/search/DataSheet.aspx?MatGUID=284aa856470341acbf3c16bc90849ea1).

 

[r13]

This is what I would use:

USS: Ultimate Shear Strength, UTS: Ultimate Tensile Strength, SYS: Shear Yield Stress, TYS: Tensile Yield Stress

 

[desertfox]

Hello jackRu

If you use the table above posted by retired13 you need to put a safety factor on the yield shear stress ie divide the SYS by 2 and use that figure to calculate maximum torque.



“Do not worry about your problems with mathematics, I assure you mine are far greater.” Albert Einstein

 

[JackRu]

Thanks retired13. This is what I came up with:

I am trying to replicate an increment tree borer. Note that I was told the A2 tool steel hardened to 48 HRC is the material of the actual increment borers, which have been field-tested to 90ft-lbs.

 

[drawoh]

JackRu,

4130 steel is documented on Matweb.com. Just type "steel[ ]4130" in the search window. For AISI 4130 Steel, water quenched 855°C (1570°F), 480°C (900°F) temper, 13 mm (0.5 in.) round, I get an ultimate tensile strength of 1145MPa, and a tensile yield of 1110MPa. They don't mention shear strength.

For steel 4130 WQT 1100, my machine textbook (V.M. Faires) lists an ultimate shear strength of 95ksi[ ](650MPa). There is a note stating that yield strength in torsion frequently falls between 0.5s[sub]y[/sub] and 0.6s[sub]y[/sub]. They suggest using[ ]0.6s[sub]y[/sub].

It really helps if you discuss this stuff at work with someone who has, for some reason or other, acquired a textbook on machine design.

--
JHG

 

[r13]

I think drawoh's finding, 0.6s[sub]y[/sub] is the prevailing consensus.

 

[JackRu]

Here is the updated table based on your recommendations

It appears that quenching 4130 at 1575°F and tempering it at 400°F would achieve similar torque values to the actual material used, AISI A2. The manufactures of the increment borer claim that it can withstand up to 90 ft-lbs of torque (field tested). My only concern is that 4130 would become too brittle at such a low tempering temperature (near its maximum hardness) and will not have enough tensile toughness to withstand such a high torque. Any thoughts on this? Would these values help in gauging the toughness of 4130 under these conditions: qt@400°F - elongation in 2" is ~15% and its reduction in area is ~42%, Izod impact is ~31 ft-lbs/in?

Thank you for your responses, they have shed quite a bit of light on the situation.
- Jack