yoshimitsuspeed
Automotive
- Jan 5, 2011
- 191
So my partner and I have come to a little bit of a disagreement. He is generally the one to go to on topics of maths and physics but I am quite sure I got him on this one.
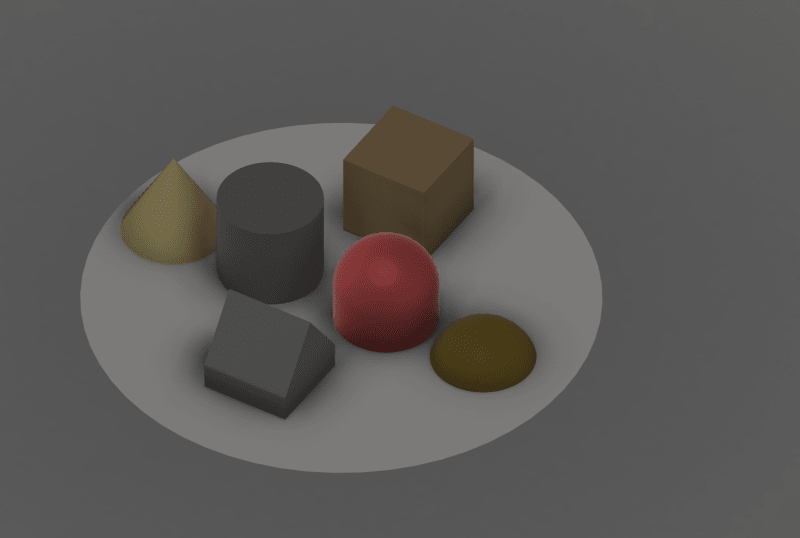
We are creating a dome form using a bladder shaped much like the red container in the image. It has a cylinder section with a hemispherical dome on top. The bladder will be bolted to a cement pad under a steel hoop. The fasteners are close enough that they are quite overkill but I still want to make sure our numbers are correct, plus just to make sure we are doing all calculations properly for future knowledge.
Here is where the disagreement lies. We are trying to calculate uplift on the bag. I am convinced the uplift depends solely on the pressure in the bag and the area on the ground. I believe the uplift should be calculated by the area times the pressure. In which case since all of the containers in the image have the same sectional area on the ground they should all see the same amount of uplift, though understandably the forces on a specific area of the square containers may be different. Therefore I would calculate uplift for the cylinder the same as I would the tall dome on a cylinder or the short dome.
His argument is that the pressure is only pushing straight up at the center of the dome and therefore the uplift would be about half that of the cylinder.
Perhaps this is more of an issue of communication or ability to explain our views so maybe just seeing some other perspectives or better explanations of the scenario would help.
To give a real world example by my calculation if we have a 3.6 meter diameter dome that's 10.18 square meters. If we fill the dome with 10 KPA that would be 10,000 N/m2 times 10.18 we would have 101,800 newtons of upward force on the dome. If we have 20 anchors then each anchor should see 5090 newtons of tensile force or thereabouts not counting other stresses from the base being pushed outward and whatnot.
Does that math in fact check out and can you confirm that the geometry of the top of the container is irrelevant to the lifting force on the perimeter of the container? And if so perhaps you can explain better than I can in a way that will help my partner understand why.
We are creating a dome form using a bladder shaped much like the red container in the image. It has a cylinder section with a hemispherical dome on top. The bladder will be bolted to a cement pad under a steel hoop. The fasteners are close enough that they are quite overkill but I still want to make sure our numbers are correct, plus just to make sure we are doing all calculations properly for future knowledge.
Here is where the disagreement lies. We are trying to calculate uplift on the bag. I am convinced the uplift depends solely on the pressure in the bag and the area on the ground. I believe the uplift should be calculated by the area times the pressure. In which case since all of the containers in the image have the same sectional area on the ground they should all see the same amount of uplift, though understandably the forces on a specific area of the square containers may be different. Therefore I would calculate uplift for the cylinder the same as I would the tall dome on a cylinder or the short dome.
His argument is that the pressure is only pushing straight up at the center of the dome and therefore the uplift would be about half that of the cylinder.
Perhaps this is more of an issue of communication or ability to explain our views so maybe just seeing some other perspectives or better explanations of the scenario would help.
To give a real world example by my calculation if we have a 3.6 meter diameter dome that's 10.18 square meters. If we fill the dome with 10 KPA that would be 10,000 N/m2 times 10.18 we would have 101,800 newtons of upward force on the dome. If we have 20 anchors then each anchor should see 5090 newtons of tensile force or thereabouts not counting other stresses from the base being pushed outward and whatnot.
Does that math in fact check out and can you confirm that the geometry of the top of the container is irrelevant to the lifting force on the perimeter of the container? And if so perhaps you can explain better than I can in a way that will help my partner understand why.