Tyannotti
Structural
- Apr 20, 2020
- 2
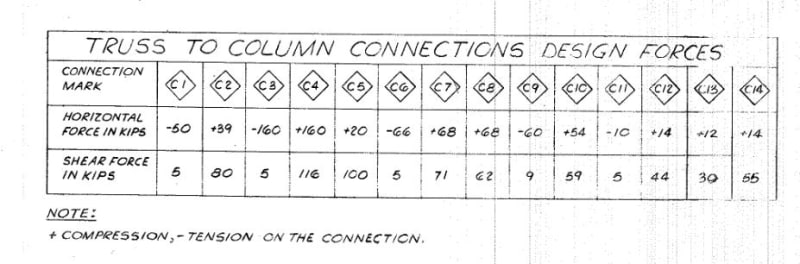
Does anyone out there know how to use just the tension and compression loads to find the total capacity of the girder in PLF? Or to convert to equal point loads?
See the attached sketch.
According to the attached drawing the reaction is 99 kip - correct? So, that would work out to 2,148 PLF capacity (99,000 lbsF x 2/92'-2") - correct?
But I want to get the capacity (or point loads) by using and verifying the tension and compression forces (when given).
In other words, if all I had was the tension and compression forces - what would the capacity be in PLF?
See the attached sketch.
According to the attached drawing the reaction is 99 kip - correct? So, that would work out to 2,148 PLF capacity (99,000 lbsF x 2/92'-2") - correct?
But I want to get the capacity (or point loads) by using and verifying the tension and compression forces (when given).
In other words, if all I had was the tension and compression forces - what would the capacity be in PLF?