Robert Soley
Structural
- Nov 11, 2018
- 12
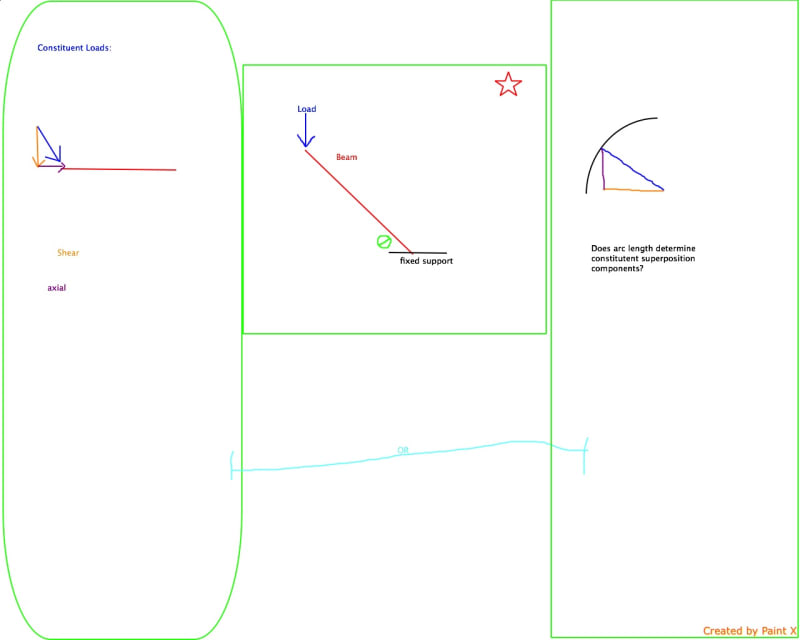
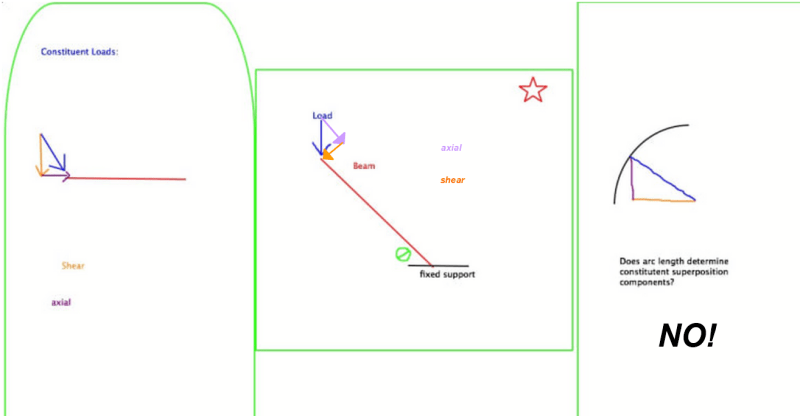
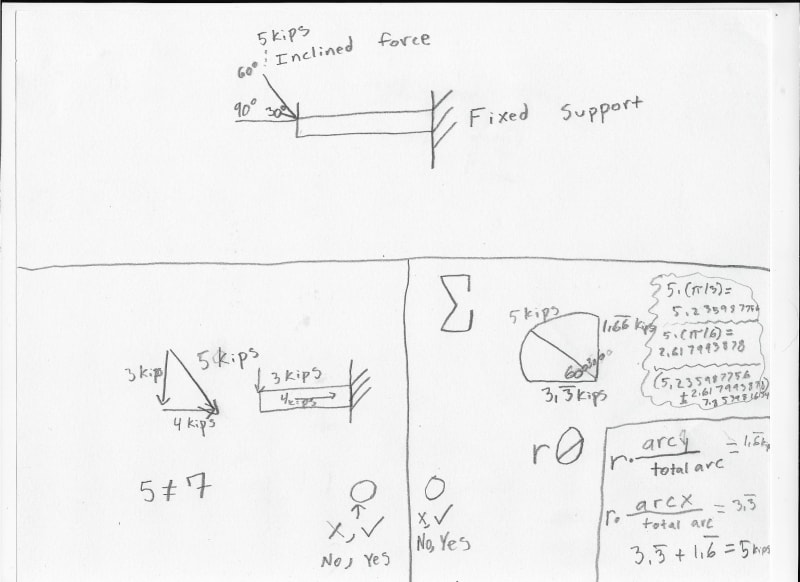
I posit it's the right side, you find the percentages & then calculate from total load; but I have no way to test this.
Follow along with the video below to see how to install our site as a web app on your home screen.
Note: This feature may not be available in some browsers.