Deflection of sistered composite wood beams

[EngDM]

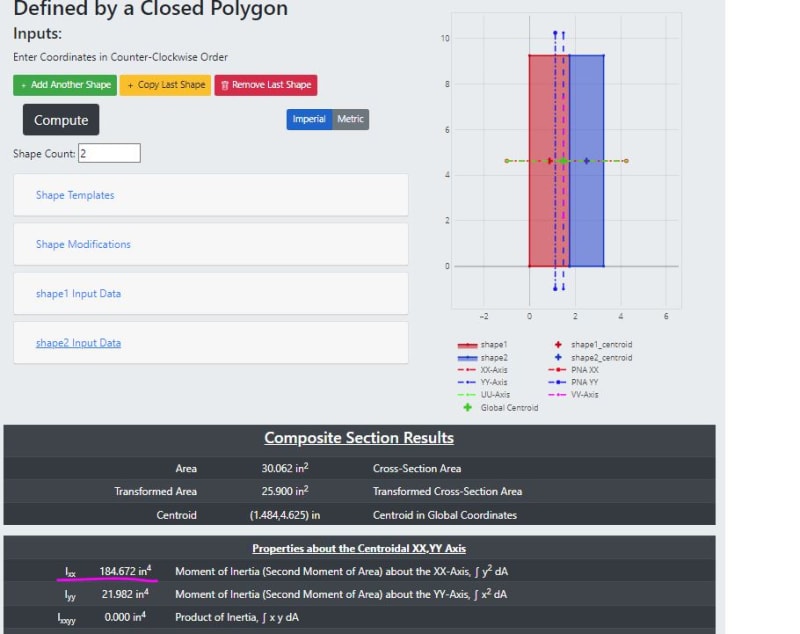
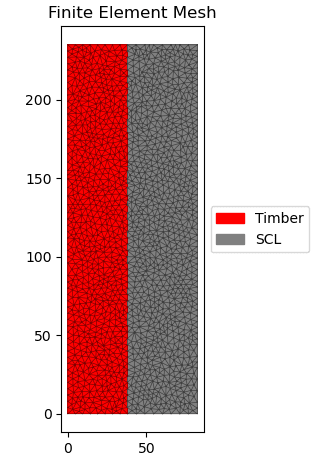
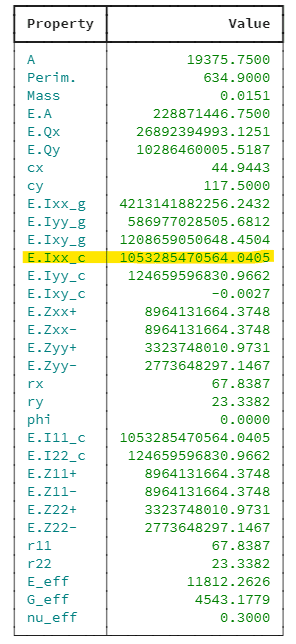
I have come across this a few times now where an existing building is being given an addition which has a much higher roof. We plan to reinforce the existing 2x10 roof joists with LVL's as the drifting snow load is quite large. However, how does one assess the deflection accurately given that the E's are different? They are sistered side by side, would it be as simple as finding the weighted EsI for the combined shape?

In the past I have just sized the new reinforcing to take the entire deflection, which is way over-designed if deflection is what governs in my opinion, but it is quick.

 

[driftLimiter]

what exactly did you model with sectionproperties?

The important part of what has been said is that if you look at them individually then it shouldn't be the same result as if you look at them as a composite.

I would expect the full composite behavior to be more favorable in terms of deflection. The reason why many people just look at them as fully non-composite beams is that the connection between the plys can be difficult to achieve the full composite.

If you put both sections into a section analysis software and include the difference in E in that analysis, it should provide a fully composite result based on the transformed section properties.

 

[Celt83]

Here is an example:
Assumptions:
2.0E Weyerhaeuser for the LVL
E[sub]lvl[/sub] = 2,000 ksi
Fb[sub]lvl[/sub] = 2.6 ksi
Ixx[sub]lvl[/sub] = 115.42 in[sup]4[/sup]

No.2 SPF 2x10
E[sub]210[/sub] = 1,400 ksi
Fb[sub]210[/sub] = 0.875 ksi
Ixx[sub]210[/sub] = 98.932 in[sup]4[/sup]

Transformed Ixx, assuming E[sub]lvl[/sub] as the basis:
Ixx[sub]composite[/sub] = 184.672 in[sup]4[/sup]
E[sub]composite[/sub] = 2,000 ksi

Deflection Formula for a simply supported uniform loaded beam:
delta = (5*w*L[sup]4[/sup]*1728) / (384*E*I) = (22.5*w*L[sup]4[/sup]) / (E*I)

EI[sub]composite[/sub] = 369,344 k*in[sup]2[/sup]
delta = 6.09188e-5 *w*L[sup]4[/sup]

Load distributed based on member stiffness:
∑ EI = 369,344
EI[sub]lvl[/sub]/∑ EI = 0.625
EI[sub]210[/sub]/∑ EI = 0.375

delta[sub]lvl[/sub] = 0.625*22.5*w*L[sup]4[/sup] / EI[sub]lvl[/sub] = 6.09188e-5 *w*L[sup]4[/sup]
delta[sub]210[/sub] = 0.375*22.5*w*L[sup]4[/sup] / EI[sub]210[/sub] = 6.09188e-5 *w*L[sup]4[/sup]

 

[EngDM]

what exactly did you model with sectionproperties?

See screenshot below:

2x10:
E = 9500MPa
1-3/4"x9-1/4" 2.0E LVL:
E = 13789MPa

When I run the 5*w*l^4/384EI equation with the EI in the screenshot under the same loading as when I did the original approach to split the loading based on stiffness to match deflection, I get a much larger deflection when using the composite EI.