Direct analysis: Notional load instead of Tau_b factor

[Settingsun]

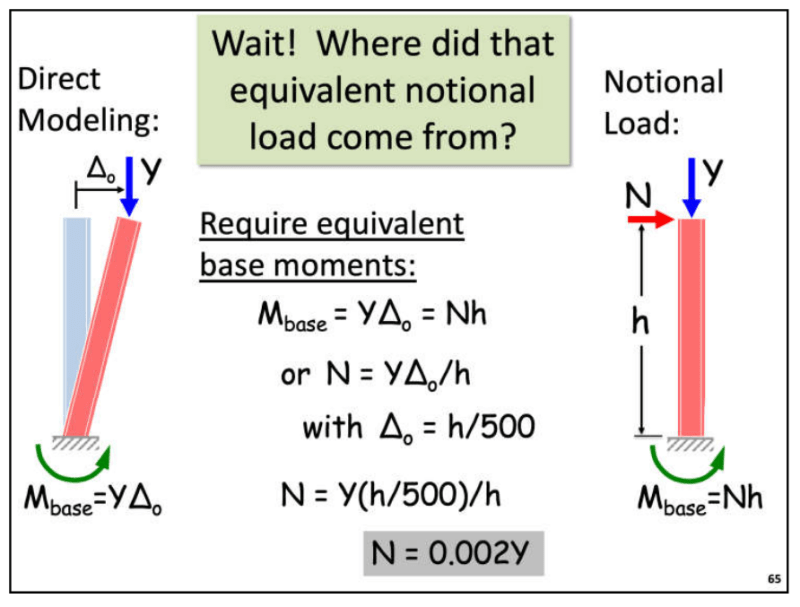
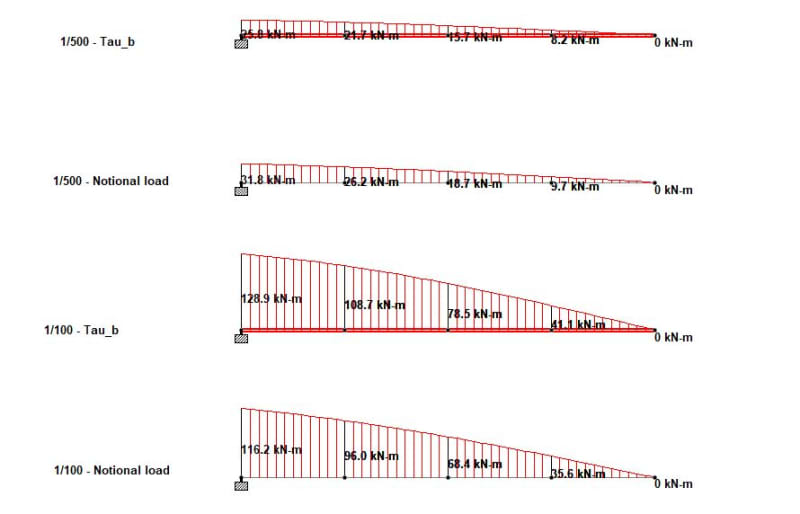
Does anyone know the derivation of the additional notional load that can be used instead of the Tau_b stiffness reduction factor? I want to know whether the notional load should be increased if the out-of-plumb is more than the assumed L/500.

 

[Ingenuity]

steveh49: No 'kicks at this can' so far, so I will give it a go:

Notional loads can be applied as part of the initial system imperfections (out-of-plumbness) OR, if you do not use notional loads, you can model the imperfections directly in the analysis.

AISC 360-16 Chapter C, clause 2b (c) states:

So, you if have a situation where your out-of-plumbness is say H/250, then you notional load coefficient would simply double to 0.004


The derivation of the notional load for an assumed H/500 out-of-plumbness is as follows (from Ziemian):

Tau_b accounts for adjustment to stiffness and is not related to notional loads, so regardless if you use direct modelling or notional loads, you still have to consider τ_b (although τ_b may be 1 if your required axial strength to cross-section compressive strength is less than 0.5).

AISC 360-16 Specification and Commentary is available for free here: Link

 

[JLNJ]

If memory serves, the Tau_b is to account for the frame being less stiff in real life than the simplified version we assume in our analyses. We model moment connections as perfectly and ideally rigid but they are actually more flexible than we normally account for.

 

[Settingsun]

Hi both, thanks for the replies.

Ingenuity, I'm referring to C2.3(c) which gives 0.001*axial force as an additional notional load which allows Tau_b to be taken as 1.0, removing the dependency on axial stress.

JLNJ, the commentary says it's a safety factor for slender columns (elastic buckling) which matches your understanding. For more stocky columns, it puts it entirely down to inelasticity (premature yielding).

I was sceptical that the 0.001 load would be enough in the case of largish lateral loads so ran the cases shown in the image, hopefully explained well enough. The axial force is 70% of yield so Tau_b = 0.84. The length of the cantilevers is such that the axial load is 40% of the Euler load. I ran 1/500 (top two cases) and 1/100 (bottom two cases) notional loads for out-of-straight effects; and Tau_b vs additional notional load. For 1/500, the additional notional load is 50%, but only ~10% for the 1/100 case.

[Edit: Staad tricked me yet again by changing all stiffness back to 205GPa as is typical for steel rather than the reduced values - have I mentioned how much I hate this software?] I've updated the image above and the results are still comparable but whether Tau_b or notional load is more conservative depends on the magnitude of the other transverse loading, pretty much as you would think.

 

[JoshPlumSE]

Let me take a stab at this:

1) Derivation of Notional Load based on L/500 out of plumbness:
Ingenuity is correct about this in that the derivation of the 0.002 notional load (AISC section C2.2b, equation C2-1) is based on the L/500 out-of-plumbness and can be adjusted in cases where the use of a different out-of-plumbness is justified.

2) Purpose of Tau_b:
I don't believe JLNJ is quite correct when he asserts that Tau_b is related to the connection stiffness. Instead, it is an attempt to approximate the fact that most steel columns will experience INELASTIC buckling. Therefore, an analysis which captures geometric non-linearity (e.g. P-Delta) which does a good job of capturing elastic buckling would still not be sufficiently accurate to capture the behavior of most steel columns. That's why the Tau_b factor was introduced. I'm sure there are some good technical articles about this out there, but I don't have them off hand. I'd look for some co-authored by Don White (of Georgia Tech) between 1998 and 2005.

There were originally TWO Tau values, this is discussed in the commentary to section C2.3. One for intermediate or stocky columns (where inelastic buckling governed) and one for very slender ones (where elastic buckling governed).

3) The use of Notional Load to use a constant Tau_b:
This is based on section C2.3 where it says (paraphrasing), "it is permissible to use Taub = 1.0 if an additional notional load of 0.001*gravity loads is applied at all levels, in all load combinations".
Note that this applies to ALL load combinations, whereas the original notional loads only applied to gravity only load combinations (unless your 2nd order drift is quite high).

The purpose of this clause, I believe, was to allow a simpler design method for engineer who didn't want to (or couldn't) adjust the stiffness of their structural members based on axial load. That's really convenient because it allows for a non-iterative analysis.

To me, this 3rd item is the basis of the OP's original question and I don't have a great answer to how this was derived. I don't believe that there is anything in the code that would prevent him from using this in lieu of Tau_b if the out-of-plumbness were greater or less than L/500. Personally, I would use the 0.001 value for any out-of-plumbness that is less than L/500 and adjust it to 50% of the other notional load if out-of-plumbness were greater than L/500.

But, I would want to check my 2nd order amplification factor. If it is greater than 1.7, then I'd want to do the Tau_b adjustment.... Heck, if it were greater than 1.7, then I'd want to change my structure and stiffen it up!