Navigation
Install the app
How to install the app on iOS
Follow along with the video below to see how to install our site as a web app on your home screen.
Note: This feature may not be available in some browsers.
More options
Style variation
-
Congratulations MintJulep on being selected by the Eng-Tips community for having the most helpful posts in the forums last week. Way to Go!
You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
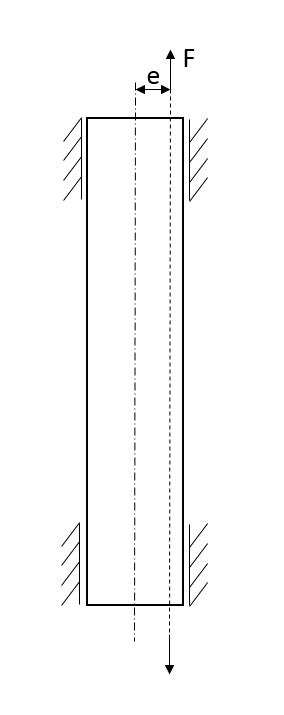
Eccentrically loaded beam moment distribution
- Thread starter Chris10B
- Start date
- Status
- Not open for further replies.
if fixed at both ends then the "beam" is unloaded.
how does the beam interact with the rest of the world ? You show (carefully) no contact at the ends. Say there's a shear connection at the bottom end, then this'll react the beam axial load and the beam will "cock" in the clearances and develop a lateral couple to react the offset load. If the shear attmt is inline with the load then no moment to "the rest of the world", but there'll be an internal moment in the beam.
clear as mud ?
another day in paradise, or is paradise one day closer ?
how does the beam interact with the rest of the world ? You show (carefully) no contact at the ends. Say there's a shear connection at the bottom end, then this'll react the beam axial load and the beam will "cock" in the clearances and develop a lateral couple to react the offset load. If the shear attmt is inline with the load then no moment to "the rest of the world", but there'll be an internal moment in the beam.
clear as mud ?
another day in paradise, or is paradise one day closer ?
If the ends are fully fixed, the beam does not feel the effect of the force F, i.e. the beam has no stress and no deformation.
If one end of the beam is fixed against rotation but free to move in an axial direction and the other end is fully fixed, the beam feels an axial stress of F/A but no bending.
What is the meaning of "overdetermined"?
BA
If one end of the beam is fixed against rotation but free to move in an axial direction and the other end is fully fixed, the beam feels an axial stress of F/A but no bending.
What is the meaning of "overdetermined"?
BA
Well, a beam fixed at both ends against rotation is theoretically indeterminate in the sense that the number of reactions exceeds those determined by statics alone but in this case, it is a trivial indeterminacy because the applied moment is resisted by the fixed ends.
If both end supports permit axial translation, the beam is unstable. The beam needs one reaction to prevent translation. It can be at an end or anywhere along the beam, but it is necessary for stability.
BA
If both end supports permit axial translation, the beam is unstable. The beam needs one reaction to prevent translation. It can be at an end or anywhere along the beam, but it is necessary for stability.
BA
- Thread starter
- #8
Re-reading this after myself, I really did not do a good job at explaining the load case!
I think the following diagram explains it better.
The case is of an eccentrically loaded tension specimen with the intention of superimposing tension and bending loads.
Fixed supports are actually roller supports, as displacement is allowed in x, but is fixed in y. Under tensile loading the beam center-line (red) should then deflect toward the overall center-line. As a result, there is a bending moment in the beam. What is the distribution of this bending moment along the beam in the x direction?
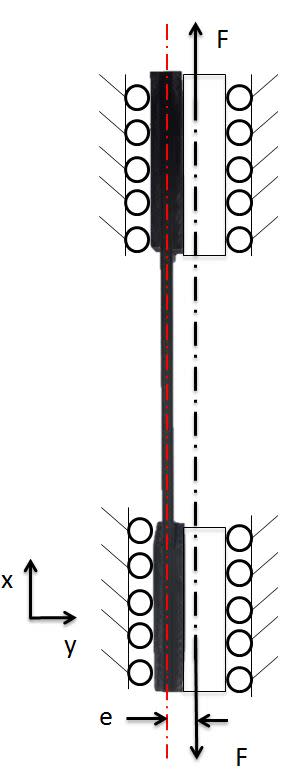
I think the following diagram explains it better.
The case is of an eccentrically loaded tension specimen with the intention of superimposing tension and bending loads.
Fixed supports are actually roller supports, as displacement is allowed in x, but is fixed in y. Under tensile loading the beam center-line (red) should then deflect toward the overall center-line. As a result, there is a bending moment in the beam. What is the distribution of this bending moment along the beam in the x direction?

ok, that's clearer !
there is some moment restraint at each end. Draw a FBD of 1/2 the specimen. In all likelihood the unsupported mid-span of the specimen will deflect towards the load line of action, reducing the off-set moment; this moment will divide itself between the ends and the mid-span.
Depending on what you're trying to test, you could put a hinge at the end of the specimen, then the specimen would be in tension and the off-set moment reacted at the ends (ie a simple tension test). If you remove the rollers, and maybe load on a single point, you'd see the ends rotate as the mid-span tries to unload moment.
another day in paradise, or is paradise one day closer ?
there is some moment restraint at each end. Draw a FBD of 1/2 the specimen. In all likelihood the unsupported mid-span of the specimen will deflect towards the load line of action, reducing the off-set moment; this moment will divide itself between the ends and the mid-span.
Depending on what you're trying to test, you could put a hinge at the end of the specimen, then the specimen would be in tension and the off-set moment reacted at the ends (ie a simple tension test). If you remove the rollers, and maybe load on a single point, you'd see the ends rotate as the mid-span tries to unload moment.
another day in paradise, or is paradise one day closer ?
I guess if the two ends of the member cannot rotate, there is NO moment in the member. Hook's Law - for internal force to occur in a member, you must have a corresponding deflection.
Since 0 rotation, then 0 moment.
The F x e moment is taken "out" by the boundary condition at that point since you dis-allow rotation in the member at the end.
Check out Eng-Tips Forum's Policies here:
faq731-376
Since 0 rotation, then 0 moment.
The F x e moment is taken "out" by the boundary condition at that point since you dis-allow rotation in the member at the end.
Check out Eng-Tips Forum's Policies here:
faq731-376
- Status
- Not open for further replies.
Similar threads
- Question
- Replies
- 3
- Views
- 2K
- Replies
- 3
- Views
- 5K
- Replies
- 11
- Views
- 1K
- Replies
- 9
- Views
- 8K
- Question
- Replies
- 21
- Views
- 5K