Effective Length situation 1

[BST-98]

Good day, All

My query is regarding this situation between steel beams supported at either ends with a column in the middle being braced along its weak axis.

If the beam can take 2% of the axial load applied on the column, does this mean that the column is therefore braced in it's strong axis and therefore the effective length for the strong axis is the same as the weak axis and design the axial load for a shorter length column than the full length?

For further context, the beam column connection is designed so that the flanges of the column are prevented from buckling.
To my understanding the answer above is yes, but I'm hearing mixed discussions and would like to clarify

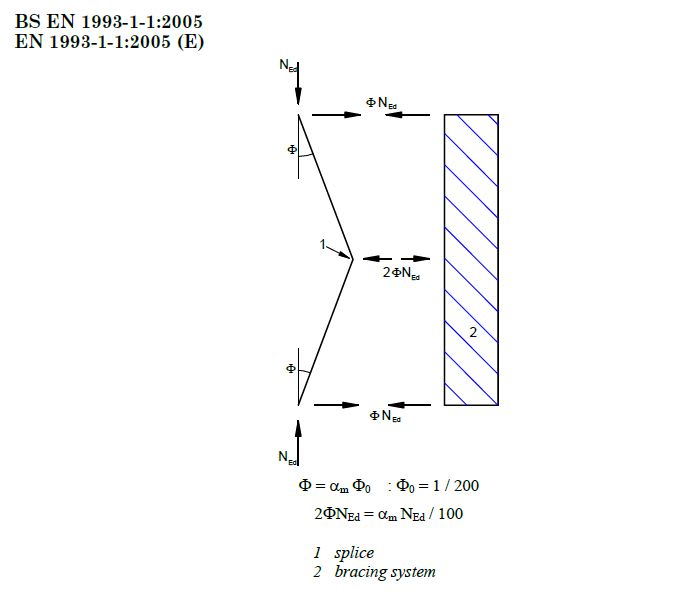
Image below is for reference, excuse the stick diagram.

 

[271828]

The beams will serve as nodal braces if they are strong AND stiff enough. This situation is evaluated using the AISC Specification Appendix 6.

 

[BAretired]

If the beam can take 2% of the axial load applied on the column, does this mean that the column is therefore braced in it's strong axis and therefore the effective length for the strong axis is the same as the weak axis and design the axial load for a shorter length column than the full length?

No, that is not what it means. The effective length for the strong axis is the full height of column or the spacing of strong axis bracing if applicable.

For further context, the beam column connection is designed so that the flanges of the column are prevented from buckling.
To my understanding the answer above is yes, but I'm hearing mixed discussions and would like to clarify

If the beam to column connections are rigid, the effective length for weak axis buckling is less than the spacing of beams, but that does not affect the effective length for strong axis buckling.

 

[human909]

You seem to be confusing Euler buckling (global column buckling) with lateral torsional buckling.

The normal approach would consider column unrestrained towards the top left and bottom right of the image. So the effectively lenght is that of a pinned-fixed column of full length with no influence of the other members,

If you wanted to dig deeper the other beams would provider some minor restrain in the other axis and you would get a small reduction of effective length. However calculating this confidently would not be simple.

 

[TLHS]

Are you asking if the beams will brace the column strong axis through bending in the beam? I'm assuming that's why you're trying to talk about the beam fixity. Are the beams continuous across the column or are they pinned there?

In theory you could brace via bending in the beams. It's pretty unlikely that you'll get the needed stiffness though.

Is this something you're constrained into doing for some reason? It's pretty unusual.

 

[Tomfh]

If the beams have sufficient out of plane strength/stiffness they can brace the column.

 

[BST-98]

Beam is continuous past the column.

It's an atrium area in a high rise supporting glass work. Architects don't want to see beams bracing in the strong axis.

 

[BAretired]

In that case, each beam spans the full length between fixed ends with a concentrated load at midspan equal to 2% of column axial load.
Deflection must be within limits prescribed by code. Alternatively, remove the column and design the beam to span full length.

 

[human909]

BARetired I am not sure that is wise or correct advice to be giving the user who seems to be out of his/her depth in calculating the effective length of the column.

2% strength capacity is not a sufficient check to determine whether the beams brace the column out of plane.Stiffness is.the primary factor and that check doesn't not take stiffness directly into account.

It is a check for bracing element in the plane of the restraint. As I and others have mention you need to check based on the stiffness of the restraining member.

 

[SWComposites]

Sounds like a situation where a FEM of the column+beam system should be used, with appropriate safety factors, to validate the column buckling capability.

 

[BAretired]

and BA[/color]]BARetired I am not sure that is wise or correct advice to be giving the user who seems to be out of his/her depth in calculating the effective length of the column.

2% strength capacity is not a sufficient check to determine whether the beams brace the column out of plane.Stiffness is.the primary factor and that check doesn't not take stiffness directly into account.

You may be right. I'm using a 20 year old CISC handbook which may have changed in the intervening years. There are two requirements listed to determine bracing requirements for a column. One is strength, the other displacement. The strength requirement is given as 0.02 times the factored compressive force, C[sub]f[/sub] at each brace point. The displacement of the bracing system, Δb in this case a beam at each brace point is expressed as a function of P[sub]b[/sub], β, Δ[sub]o[/sub], C[sub]f[/sub] and L. I assumed there was something equivalent in other codes. I do not know which code applies in South Africa, the home of the OP.

It is a check for bracing element in the plane of the restraint. As I and others have mention you need to check based on the stiffness of the restraining member. Displacement, I believe, is a measure of stiffness.

The Canadian code is based on work by George Winter and is based on an assumed initial misalignment of the column, Δ[sub]o[/sub], a factor β which varies for the number of equally spaced braces, and L, the length between braces.

My earlier post should have mentioned that a special limit on displacement should be applied.

 

[human909]

Yes. Most codes to.my knowledge use a strength approach with little comment on stiffness. I believe due to the complexities involved. I use AS codes and for me it is 2.5% strength check. But no comment made about the effectiveness of our of plane braces.

Yura has papers that has commented on stiffness based approaches.

I'd probably SW's approach and use a software approach with a healthy margin and be VERY careful about my assumptions of the rigidity of the connections.

 

[Tomfh]

In what sort of circumstances does bracing pass the 2 (or 2.5%) strength check but fail the required stiffness?

 

[HTURKAK]

The answer is yes. The beams at figure you have posted are fixed at one end and non-sway frame .
I am more familiar with Eurocodes and the requirement is , verification of columns are tied into all attached beams by a minimum resistance of 1.0 % of the column force.

Pls look to the attached doc. there are worked examples .

He is like a man building a house, who dug deep and laid the foundation on the rock. And when the flood arose, the stream beat vehemently against that house, and could not shake it, for it was founded on the rock..

Luke 6:48

 

[human909]

.The answer is yes.

Unless I'm mistaken with what you mean the answer is most certainly not yes and assuming so is a dangerously false assumption.

In what sort of circumstances does bracing pass the 2 (or 2.5%) strength check but fail the required stiffness?

Many circumstances,

Extreme ones can be a flexible cable or spring. The column can reach its critical buckling point before the brace reaches a load anywhere near 2%.

Less extreme cases are strong but flexible beams. If I wasn't posting from my phone I'd come up with a specific examples.

Preventing buckling is mostly about stiffness. Strength is almost a minor point in comparison, just to ensure that a stiff brace can handle the suitable loads.

 

[BAretired]

I am attaching the page in CISC with regard to the analysis of bracing.

 

[HTURKAK]

In general , i prefer not to reply unless my nickname is stated. In this case , i prefered to copy and paste of the relevant figure at EN 1993 .

φ0 is the basic value for global imperfection φ0 = 1/200




He is like a man building a house, who dug deep and laid the foundation on the rock. And when the flood arose, the stream beat vehemently against that house, and could not shake it, for it was founded on the rock..

Luke 6:48

 

[human909]

So let me clarify?

So are you both are suggesting stiffness doesn't matter? Only strength matters?

And//Or that a strength check is always sufficient no matter what circumstances?

And/Or be because the strength check is the only check explicitly required then under code then other checks are never needed?

All of these are quite radical suggestions to be made without evidence. Especially when that CISC explicitly mentions the need to limit deflection.

 

[BAretired]

and BA[/color]]So are you both suggesting stiffness doesn't matter? Only strength matters?
both? If that includes me, I can assure you, it is not something I am suggesting.

And//Or that a strength check is always sufficient no matter what circumstances? I'm not suggesting anything of the sort. A member in bending will have far too much deflection at midspan to effectively brace a column unless it is extremely stiff.

And/Or because the strength check is the only check explicitly required then under code then other checks are never needed?
Not true, codes I know require both strength and stiffness to be checked.

 

[human909]

I apologise for misinterpreting you BAretired.