Fluid flow 3 user defined generic 1

[dimitp]

Hi. Im using FF3 and want to see if a pump will be sufficient in different setups.

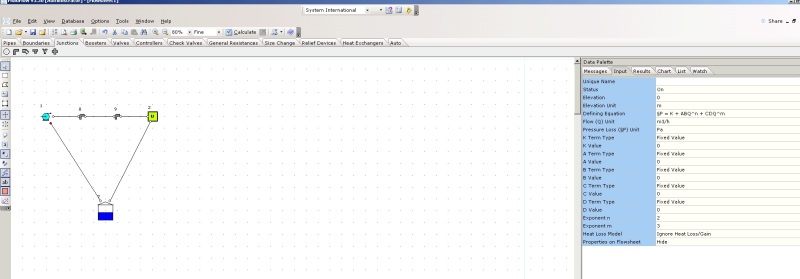
Behind the pump is a user defined generic with a defining equation: K+ ABQ^n+CD^m, where Q is given by what ever pump i put in

The input to the user defined generic i have is X*v^2 (where V is velocity) for the pressure drop. how do i convert this to fit FF3:s defining equation?

 

[BigInch]

It would be good to know what that equation is supposed to be equal to. What is it calculating, Head, or pressure increase?

A reasonably accurate function for a pump's Differential Head in terms of flowrate Q can be generated using a similar equation.

H(Q) = Hs + A Q^2 + B Q

Hs is pump differential head at a flowrate of 0, shutoff head.
A is usually a negative value.
You adjust those A and B coefficients to give you a curve that looks like your pump's H-Q curve.

Pressure increase is simply H(Q) * Specific gravity * Fluid Density

In your equation above, your AB corresponds to my A, your D corresponds to my Q and your C=1. I'd let n = 2, or thereabouts, and m = 1

 

[dimitp]

Hi again. Thank you for your input.

So to specify:

I want to use the functionality of FF3 (Fluid Flow 3) to determine if a specific pump can cope with different heights above a tank. the pump will supply a filter that will give a pressure drop. However i am unfamiliar with the Defining equation: §P = K + ABQ^n + CDQ^m , see picture:

The only thing i have in terms of pressure drop values from this filter is a modified Bernoulli’s eq: X*v^2 for different velocities, v. So the question is how do i use my pressure drop equation (X*v^2) in FF3?

 

[BigInch]

Solve for X in your dP = X * v^2
X = dP / v^2

in K+ ABQ^n+CD^m
K = 0
A = 1
B = X
Q = v
n = 2
CD = 0
m = 1

 

[dimitp]

Q=v : cant be correct. They are two different variables

Q= flow, witch to my knowledge is dependent upon the booster specified in FF3 and v is the mean flow velocity.

Am i missing something?

 

[BigInch]

Q = V * A
A is a constant
Therefore Q and V relate directly.

 

[dimitp]

Yes i have thought about that but the user defined generic is a special type of filter that has multiple areas. i guess i will have to go with multiplying by a mean A for this filter.
Thank you advance!

 

[BigInch]

Let A = 1 and your function will be in terms of Q instead of V.