teknomania
Mechanical
- Nov 22, 2007
- 26
Hi,
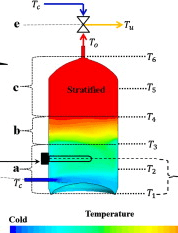
I try to model a sensible stratified storage tank by use of multi-node method (splitting tank into segments in 1D). At this stage, I am in focus on the heat loss from the storage tank (in fact from each of these segments).
So two case exists: (i) one idle period without charging or discharging so the stored medium loose heat to the environment, which I modelled by natural convection, and (ii) the other period is with charging/discharging, modelled by forced convection.
IDLE PERIOD - Natural Convection
I used the formulation 9-21 (shown below) from Yunus Cengel's heat transfer book for the natural convection: which is for vertical plates but stated to be valid for large storage tanks.
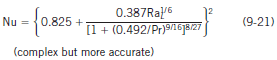
DIS/CHARGING PERIOD - Forced Convection
I tried the Nusselt correlations given in Correlations for Convective Heat Transfer. It doesn't matter which correlation I tried for laminar flows through the storage tank: at low flow conditions, the heat loss during dis/charging (forced convection) results in lesser value than natural convection! This does not sound correct to me! Asking you:
Is it possible physically to obtain a higher heat loss via natural convection when compared to a forced convection situation with the extremely low flow rate in a large cylindrical storage tank?
When I increase my flow rate, the forced convection becomes larger than the natural convection! The direction of flow was not included! Please see the observation results below:
System Description:
A storage tank at a diameter of 0.450 m and at a height of 5 m. Storage water medium at 60 °C and outdoor temperature at 20 °C. The total resistance (wall material, insulation, outer casing) is 2.54 °C/W.
Natural convection at idle periods results in: Qloss_natural=132.7 W
For a maximum charging rate at 0.0819 kg/s (5 liters/minute) ~ Re=103 [-]. At this maximum flow rate (5 l/m - 0.0819 kg/s) heat loss at forced convection was found to be Qloss_forced=89.1 W
Now pushing to upper flow rates just to see how forced convection changes the overall heat loss (the maximum flow is given as 5 l/m - 0.0189 kg/s cannot be exceeded normally!):
At a flow rate of 0.05 kg/s (Re=287) Qloss_forced becomes 108.5 W.
At a flow rate of 0.5 kg/s (Re=2890) Qloss_forced becomes 111.7 W.
Another question: Can any of you correct me that if these Nusselt correlations can be used for large storage tanks?
Thanks in advance. Regards.
“In life, the truest guide is science” – Mustafa Kemal Atatürk
I try to model a sensible stratified storage tank by use of multi-node method (splitting tank into segments in 1D). At this stage, I am in focus on the heat loss from the storage tank (in fact from each of these segments).
So two case exists: (i) one idle period without charging or discharging so the stored medium loose heat to the environment, which I modelled by natural convection, and (ii) the other period is with charging/discharging, modelled by forced convection.
IDLE PERIOD - Natural Convection
I used the formulation 9-21 (shown below) from Yunus Cengel's heat transfer book for the natural convection: which is for vertical plates but stated to be valid for large storage tanks.
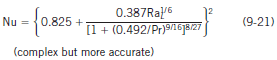
DIS/CHARGING PERIOD - Forced Convection
I tried the Nusselt correlations given in Correlations for Convective Heat Transfer. It doesn't matter which correlation I tried for laminar flows through the storage tank: at low flow conditions, the heat loss during dis/charging (forced convection) results in lesser value than natural convection! This does not sound correct to me! Asking you:
Is it possible physically to obtain a higher heat loss via natural convection when compared to a forced convection situation with the extremely low flow rate in a large cylindrical storage tank?
When I increase my flow rate, the forced convection becomes larger than the natural convection! The direction of flow was not included! Please see the observation results below:
System Description:
A storage tank at a diameter of 0.450 m and at a height of 5 m. Storage water medium at 60 °C and outdoor temperature at 20 °C. The total resistance (wall material, insulation, outer casing) is 2.54 °C/W.
Natural convection at idle periods results in: Qloss_natural=132.7 W
For a maximum charging rate at 0.0819 kg/s (5 liters/minute) ~ Re=103 [-]. At this maximum flow rate (5 l/m - 0.0819 kg/s) heat loss at forced convection was found to be Qloss_forced=89.1 W
Now pushing to upper flow rates just to see how forced convection changes the overall heat loss (the maximum flow is given as 5 l/m - 0.0189 kg/s cannot be exceeded normally!):
At a flow rate of 0.05 kg/s (Re=287) Qloss_forced becomes 108.5 W.
At a flow rate of 0.5 kg/s (Re=2890) Qloss_forced becomes 111.7 W.
Another question: Can any of you correct me that if these Nusselt correlations can be used for large storage tanks?
Thanks in advance. Regards.
“In life, the truest guide is science” – Mustafa Kemal Atatürk