steveh49 said:
But for the static assumption we make, the exact function of time doesn't matter. The load does.
I would say that the load history certainly matters if you wish to examine the interesting things that happen along the way to the final outcome, which I do.
steveh49 said:
Yes, if it gets that far. Namely, for the specific criterion of column end moment, the second-order effect overtakes the first order effect.
If it
doesn't get that far then it's a boring K=1 column and there's really nothing interesting to discuss. The notion of "overtaking" bothers me semantically. Maybe you're using at as I would, maybe you're not, it's difficult to tell. Clarifying:
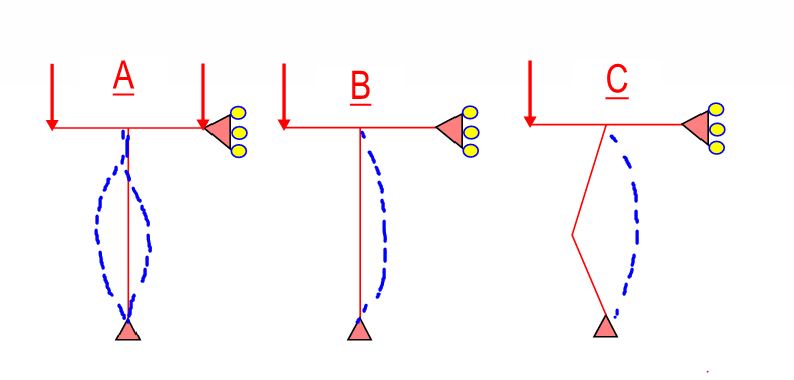
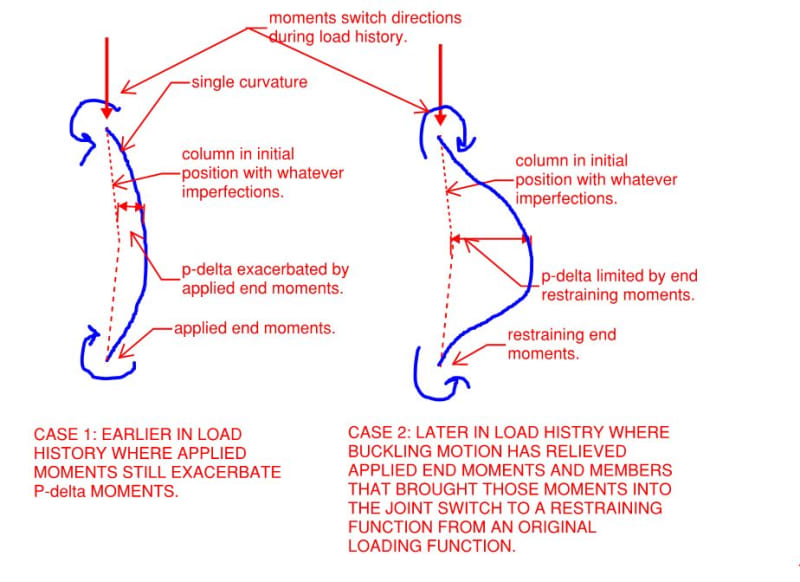
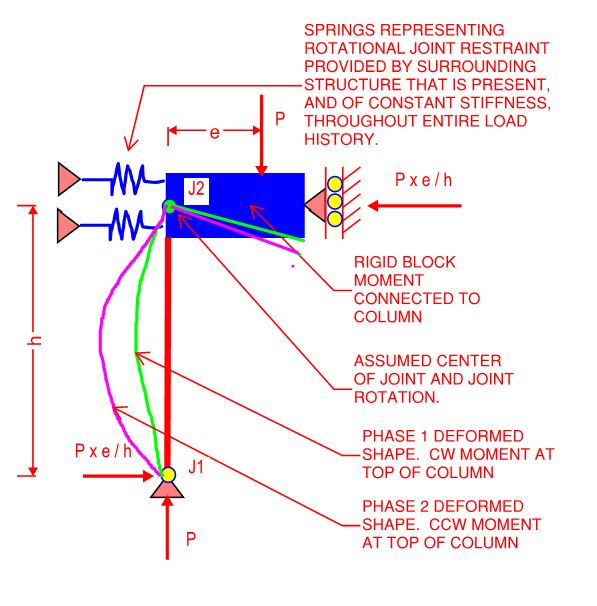
1) For all of phase one, the primary beam moment and second order moment are attempting to rotate joint J2 in the same, clockwise direction.
2) During phase one, as J2 rotates clockwise, that rotation causes the initial, primary beam moment to dissipate. The spring is being unwound. So, as the second order effect continues to grow, the first order moments are diminishing, eventually to reversal. So it's not the case that the second and first order moments are racing along in the same sense and the second order moment "overtakes" the first but, rather, the first order moment withdraws from the race and, eventually, reverses.
Is this what you mean when you use the term "overtake"?
Steveh49 said:
However, at every step of the way, the second order effect is reducing the column end moment compared to the first-order value.
I disagree. For all of phase two, the secondary moment and primary beam moment are equal and opposite. That's the the only way that equilibrium at J2 can be maintained. As such, as the second order moment grows, so does the primary beam moment. For phase one, yes, the second order effect is doing the "unwinding" that I mentioned in #2 above.
steve49 said:
And also reducing the mid-height moment compared to a pin-end column with eccentric load. The zero-crossing of the end moment is fairly arbitrary.
I disagree with that as well. If you examine my last sketch, it should be apparent that the mid-height column curvature increases at all points during the progression of the load history. And, obviously, more curvature begets more moment. The second order effect:
3) Reduces the mid-height moment
relative to what it would be in the absence of the second order effect but;
4) Does not reduce the absolute value of the mid-height moment. The mid-height moment just keeps growing as the load history unfolds.
In a very real sense, the column has to buckle as a K=1 column before continuing on to its later role as a K = 0.7-ish column. The end restraint that kicks in at the transition between phase one and phase two is what allows additional lateral movement to happen at column mid-heigh while still maintaining equilibrium.
steveh49 said:
The load increment is being delivered via the joint including first-order moment in our model, so its stiffness continues to exacerbate to some degree IMO (matter of perspective).
I disagree yet again. Even though column end curvatures reverse during the load history of the column, you were correct earlier in implying that the general trend of motion is constant always. Ergo each increment of additional load, at all times, produces these effects:
5) Additional, clockwise rotation of J2.
6) Additional, leftward lateral motion of all points along the column height.
The key here, I think, is to take that overall trend and parse it out into an examination of which members are doing what at any point in time. If you study the equilibrium of J2 during phases one and two, I believe that it works out like this:
BEM = Primary beam end moment acting on joint. That due to rotation of the joint.
CEM = Primary column end moment acting on joint. That due to rotation of the joint.
PD = P-Delta. Column end moment acting on joint due to eccentricity created by lateral column drift.
PHASE 1: PD(CW) + BEM(CW) = CEM(CCW)
PHASE 2: PD(CW) = BEM(CCW) + CEM(CCW)
In phase one, the beam (the "surrounding structure") is
assisting the second order column effect in encouraging the clockwise rotation of J2.
In phase two, the beam is
opposing the second order column effect in encouraging the clockwise rotation of J2. The second order effect alone is encouraging the clockwise rotation of J2 and the continued, leftward translation of the column between its ends.
In phase two, each increment of load is increasing the restoring, counter clockwise moment that the beam imposes on J2. That, precisely because of the negative apparent joint stiffness that you cleverly alluded to.
steveh49 said:
In terms of whether the stiffness is beneficial or not, I would propose the criterion of whether the end restraint (shorter effective length) gives greater capacity than longer effective length but no end moment (pin connection) - assuming that the structure remains stable with pin connections.
There should be no debate on that issue: the stiffness is always beneficial. That, because no matter what you assume about the end restraint, you simply do not have the option of shedding the moments. At best, you can:
1) cap the moments if cross sectional plasticization is relied upon.
2) transfer the moments to the column mid-height if if P-delta column motion is relied upon to relieve the end moments.
As always, no free lunch in Newtonian physics.