Gus14
Civil/Environmental
- Mar 21, 2020
- 194
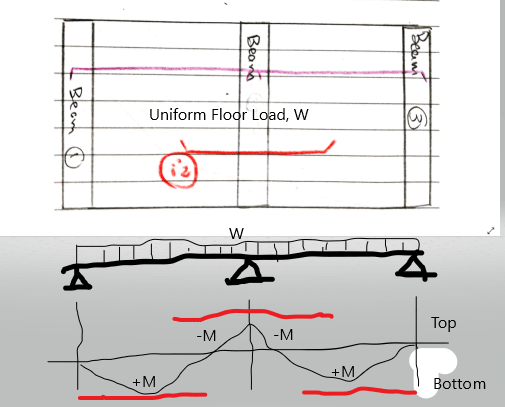
In school I learned to distribute loads from solid slabs to beams using tributary method i.e,(triangles and trapezoids). However we were always reminded that this method is only applicable while slabs are designed as simply supported but in reality we put slab top reinforcement above the beam in the middle which makes the slab behave as a continuous slab. should we remove the top slab reinforcement because this might increase the beam reaction? in the picture i'm talking about red steel.