Long Term Deflection Estimates 1

[Trenno]

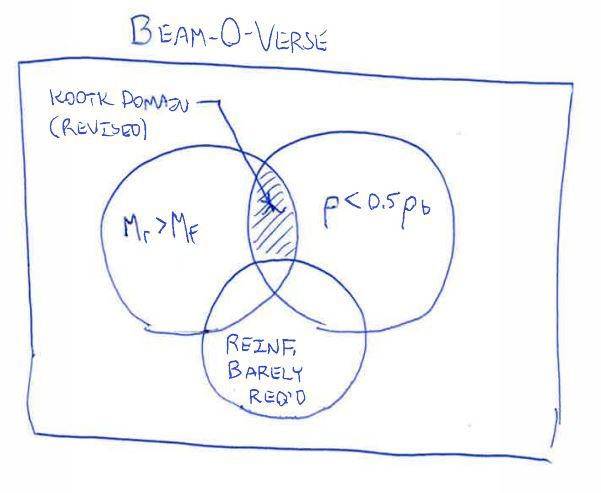
Hi all,

I know full well the ins and outs of long term deflection - creep, shrinkage, cracking, f'c, mix design, restraint, P/A, compression reo and the list goes on...

But what I'm asking here are people's 'back of the envelope' deflection estimates.

For example, I've seen people get a quick figure by factoring up the elastic uncracked deflections - 3G + 1.5Q. This can be back calculated from various code equations.

I've also seen a RAM Concept file with the load combo - 3.35G + 1.64Q (note the creep factor being set to 3.35).

Anyone have any reference material that quotes some of these elastic uncracked deflection multipliers, purely just to get ballpark figures?

Or do people more opt for span/depth ratios to give them a feel for a particular design?

 

[IDS]

an assumption not explicitly stated in the mental experiment that I posed above is this: longitudinal reinforcement was to be selected based on bending capacity. As the 0.5 rho_b algorithm is itself meant to be a rudimentary deflection check, the experiment loses all meaning if one assumes that a more detailed deflection assessment has already influenced the beam proportions.

OK, if you have two beams of the same span, and concrete type, and with the same reinforcement stress at every cross section, the one with the lesser depth will have a greater curvature, and will deflect more.

Does this mean that it is true to say "deflection problems are rarely encountered in beams with ro = 0.5ro.max"?

No it doesn't. I have encountered many deflection problems in beams and slabs with a reinforcement content much less than that. It may be true that if the beam/slab had been designed with a shallower depth and more reinforcement then the problems would have been even worse, but in that case it is likely the problem would have been recognised at the design stage, by application of the code simplified deflection calculations, which are reasonably accurate for heavily reinforced sections, and grossly inaccurate for lightly reinforced sections.



Doug Jenkins
Interactive Design Services

http://newtonexcelbach.wordpress.com/

 

[KootK]

But for less extreme changes in steel ratio, the effect will depend on whether you effect the change in steel ratio by changing the beam depth or beam width.

Per the criteria that I specified initially, the mental experiment involves modifying only the member depth and reinforcement ratio.

What it gets down to is there are too many variables involved to base it on a simplistic rule like this.

I disagree. For ordinary conditions, I've had excellent result with this algorithm.

Why not just calculate expected long term deflections based on a rational and proven model and get rid of the guesses.

Two reasons come to mind:

1) I understood the subject of this thread to be "rules of thumb" rather than "detailed deflection analysis".

2) Some folks, like me, rarely perform the detailed design of beams. Rather, I spot check the work of others. For that kind of deflection check, I find the 0.5_rho_b business invaluable. The usual situation is much simpler than the scenarios that you and IDS have been describing. Usually, it goes something like this:

a) Someone sizes a podium slab beam using normal span to depth ratios etc.
b) Because podiums see a lot more load than other floors, flexural design is challenged.
c) Because a prior depth commitment has been made, no beam depth change is possible.
d) Designer solves problem by widening the beam and packing it full of rebar including some top steel.
e) KootK reviews said beam and discovers that it's at 0.7 rho_b.
f) KootK grabs designer and says "are you sure this thing isn't a frickin' wet noodle?".
g) Designer scurries away and does the detailed, long-term deflection check that should have been performed in the first place.
h) KootK is right and something about the beam gets changed.

I have seen a lot of designs where long term deflections were a problem for lightly reinforced slabs and beams which indicates that the % reinforcement rule does not actually work for all cases.

Of course it doesn't work for all cases. Neither do the span-to depth ratios that some engineers find mildly useful. That's why it's a "rule of thumb". If it worked for all cases, we'd be calling it a "kick ass, all encompassing, stand alone deflection check".

I like to debate structural engineering theory -- a lot. If I challenge you on something, know that I'm doing so because I respect your opinion enough to either change it or adopt it.

 

[KootK]

Does this mean that it is true to say "deflection problems are rarely encountered in beams with ro = 0.5ro.max"? No it doesn't.

As I mentioned in my latest response to Rapt, my experience indicates that it generally does. Are there some exceptions to the rule of thumb? Well... yeah. However could there not be?

I like to debate structural engineering theory -- a lot. If I challenge you on something, know that I'm doing so because I respect your opinion enough to either change it or adopt it.

 

[IDS]

KootK - no-one is talking about rules that never have exceptions. The point is that in my experience, with the structures that I work on, the exceptions are not at all rare.

I see deflection problems with lightly reinforced slabs more frequently than heavily reinforced slabs.

Doug Jenkins
Interactive Design Services

http://newtonexcelbach.wordpress.com/

 

[KootK]

I feel as though we are telling two different stories here:

The KootK/Fanella Story

Flexural members proportioned such that flexural reinforcement is kept within a reasonable range seldom have deflection issues.

The rapt/IDS Story

Deflection calculations for lightly reinforced members can often be inaccurate and/or un-conservative.

I believe both of these stories to be generally true and feel that they are not mutually exclusive in any way.

Applied to conventional flexural members, the 0.5_rho_b business is essentially just an indirect way of encouraging the use of appropriately deep members. And, surely, we don't need to argue about whether or not structural depth is a good thing when it comes to limiting deflections? Right? Or does structural depth also not "have any relevance" when it comes to deflection control?



I like to debate structural engineering theory -- a lot. If I challenge you on something, know that I'm doing so because I respect your opinion enough to either change it or adopt it.

 

[rapt]

Kootlk,

2 Problems with your argument above,

1 The members we are saying have most problems and are handled worst by simplified deflection check methods are the ones that fit into your OK definition. Not sure how your logic is compatible with this.

2 The steel ratio logic does not require a depth increase. It will also be satisfied by a width increase! And depth is far more efficient than width in reducing concrete member deflections.

 

[KootK]

The members we are saying have most problems and are handled worst by simplified deflection check methods are the ones that fit into your OK definition. Not sure how your logic is compatible with this

The steel ratio logic does not require a depth increase. It will also be satisfied by a width increase! And depth is far more efficient than width in reducing concrete member deflections.

You may be erroneously assuming that the domain of consideration here is lightly reinforced members. It's not. The domain here is members that are lightly reinforced AND properly designed for flexure. There's a significant difference there.

With both of those criterion considered in tandem, the steel ratio logic does require a depth increase if one is even remotely interested in efficiency. This is because of the following relationship in which bending resistance varies with the square of depth:

Mr = rho x b x d^2 x constant (valid up to about 2/3 rho_b).

I like to debate structural engineering theory -- a lot. If I challenge you on something, know that I'm doing so because I respect your opinion enough to either change it or adopt it.

 

[IDS]

Mr = rho x b x d^2 x constant (valid up to about 2/3 rho_b).

Which could be written:

Mr = ast x b x d x constant

or for member supporting only its own weight:

Mr/M* = ast x b x constant

Doug Jenkins
Interactive Design Services

http://newtonexcelbach.wordpress.com/

 

[KootK]

I don't recognize the second form but the first should have the "b" cancelled out. Either way, where are you headed with this Doug?

I like to debate structural engineering theory -- a lot. If I challenge you on something, know that I'm doing so because I respect your opinion enough to either change it or adopt it.

 

[IDS]

Either way, where are you headed with this Doug

Just that the required steel area is proportional to d, not d^2, for constant load, or if the load is proportional to d then the required steel is constant.

But going back to your previous comment:

The domain here is members that are lightly reinforced AND properly designed for flexure.

So am I, and I presume rapt is too. Why would we concern ourselves with sections that didn't work for flexure?

Doug Jenkins
Interactive Design Services

http://newtonexcelbach.wordpress.com/

 

[KootK]

Just that the required steel area is proportional to d, not d^2, for constant load, or if the load is proportional to d then the required steel is constant.

Ok, I like algebra as much as the next guy. However, none of that changes the fact that moment resistance is proportional to d^2 once the constraint on reinforcement ratio is introduced.

So am I, and I presume rapt is too. Why would we concern ourselves with sections that didn't work for flexure?

I have no idea IDS. You guys really haven't given me much to work with here and I'm not clairvoyant. So far, I've provided anecdotes, a reference, an intuitive behavioural explanation, a mental experiment, and some math to support my claim. In contrast, you and rapt have weighed in with:

A) you don't like my idea and;
B) you know of some lightly reinforced members with deflection problems.

In the interest of debate parity, I'd love some additional info:

1) were the offending slabs properly designed for moment?
2) were the perceived deflection issues field problems or analytical shortcomings?
3) to what do you attribute the tendency for deflection issues in lightly reinforced members?
4) why are simplified deflection checks in AU unconservative for light reinforcing?
5) do you know of any publications that expand upon your concerns?





I like to debate structural engineering theory -- a lot. If I challenge you on something, know that I'm doing so because I respect your opinion enough to either change it or adopt it.

 

[Retrograde]

The Australian Code has a simplified formula for calculating the effective moment of inertia Ief for deflection calcs.

Ief = Icr + (I - Icr) (Mcr / Ms)^3

Where Ms = the service moment.

I believe that other Codes have the same or similar formulas.

I think that what RAPT and Doug are getting at is that if Mcr is close to Ms (ie. lightly loaded) this formula can be non-conservative and that a check based on 1/2_rho_b will not pick up the problem.

 

[IDS]

Ok, I like algebra as much as the next guy. However, none of that changes the fact that moment resistance is proportional to d^2 once the constraint on reinforcement ratio is introduced.

You were talking about design efficiency, so the point is that at constant steel area the moment resistance only increases in proportion to the depth, not depth squared, and if your load is proportional to depth the over-design factor doesn't increase at all. The structures I have been talking about were nowhere near the limiting reinforcement content anyway.

Quote (IDS)
So am I, and I presume rapt is too. Why would we concern ourselves with sections that didn't work for flexure?

I have no idea IDS. You guys really haven't given me much to work with here and I'm not clairvoyant. So far, I've provided anecdotes, a reference, an intuitive behavioural explanation, a mental experiment, and some math to support my claim. In contrast, you and rapt have weighed in with:

Well that's a pretty blinkered account of who has provided what, but never mind, I'll answer your questions anyway:

1) were the offending slabs properly designed for moment?

Yes. In fact the flexural design was satisfactory for the maximum moments occurring during construction, and also completed structure + live load, which are of the order of double the dead load only moment.

2) were the perceived deflection issues field problems or analytical shortcomings?

Construction was in accordance with specified requirements; so it's an analysis problem

3) to what do you attribute the tendency for deflection issues in lightly reinforced members?

Codes provide rules for "tension stiffening" after concrete cracking, but these rules tend to be un-conservative at moments just above cracking, and shrinkage effects tend to reduce the cracking moment to well below the value given by codes. Differential temperature can also have a significant effect.

4) why are simplified deflection checks in AU unconservative for light reinforcing?

Because our code requirements are based on those in ACI 318. The Eurocode rules work better.

Also the projects I have in mind are not just Australia. Also UK (worst case), Middle East, and USA and Canada.

5) do you know of any publications that expand upon your concerns?

Yes lots. Many papers by RI Gilbert, a fairly recent book by Gilbert and Ranzi, quite a bit around 2005 from Beeby, also just found this paper from some other Australian guy:

http://www.interactiveds.com.au/Publications/Deflections09.doc

Doug Jenkins
Interactive Design Services

http://newtonexcelbach.wordpress.com/

 

[dcarr82775]

I have used a creep multiplier of 4 for 2 way slabs instead of the more normal 3 per ACI.

 

[bowlingdanish]

I was of the understanding that the more reinforcement the better. Tension stiffening + compression reo stealing long term load from the concrete. I would definitely agree with KootK for transfer slabs, if they're not heavily reinforced the slabs are gonna to be huge so sweet for deflection. But I find non transfer lightly loaded slabs hardly need reo for flexure, I'd use mesh if they made it ductile, so are generally made thicker for deflection purposes.

Also, is anyone going to the Gilbert seminars 'Cracks and Crack Control in Concrete Structures' in Aus run by the concrete institute this month? Gonna get my ticket tomorrow

 

[KootK]

Well that's a pretty blinkered account of who has provided what, but never mind, I'll answer your questions anyway

Thanks for indulging me. The additional information is a great help.

You were talking about design efficiency, so the point is that at constant steel area the moment resistance only increases in proportion to the depth, not depth squared, and if your load is proportional to depth the over-design factor doesn't increase at all

I believe this statement to be in error. If designers assumed a particular A_s and then chose their member proportions accordingly then, yes, moment would be a linear function of depth. However, that's not how design generally unfolds. And it's certainly not how I would expect the 0.5_rho_p method to be applied. Instead of proportioning to a target A_s, designers would proportion to a target rho. In that scenario, moment increases with the square of depth per the relationship that I stated previously. Think of it this way:

1) If you just adjust "b" to hit the target rho, Mr is increasing as a result of additional steel.
2) If you adjust "d" to hit the the target rho, Mr is increasing as a result of additional steel and additional lever arm on that steel.

but these rules tend to be un-conservative at moments just above cracking

Now I see that you guys aren't concerned about lightly reinforced members, you're concerned about very lightly reinforced members. I guess another ride-along assumption in the 0.5_rho_b check might be that it only applies to members that actually require significant flexural reinforcing. I'll revise the domain as shown below which should still permit a fairly wide range of applicability.

I was of the understanding that the more reinforcement the better.

This certainly holds true when all other parameters are held constant. But, again, I'm not suggesting limiting reinforcement. I'm suggesting encouraging appropriate depth selection by limiting reinforcement ratio. There's a difference.

I like to debate structural engineering theory -- a lot. If I challenge you on something, know that I'm doing so because I respect your opinion enough to either change it or adopt it.

 

[rapt]

2 comments on all of the above, (probably repeating what I have said previously)

1 If you are going to control it all by adjusting depth, then there must be an appropriate width that should be used. What is it? Though not economical, width can be used to control the steel ratio to suit your initial requirement of limiting the steel ratio to less that .5. The rule is too simplistic to use reliably.

2 The paper you quoted a section out of required a steel ratio = .5. NOT <= .5. Agreed that as long as the width /depth ratio is logical (not sure what that is defined as) any member designed for strength with a steel ratio = .5 will probably be ok for deflections.
Our argument is that members with significantly lower steel ratios than .5 often have deflection problems, even if designed to the simplified code deflection rules (not simple span/depth ratios which should be removed from all codes). A major reason for this is things like Branson's formula for tension stiffening which is very un-conservative for lightly reinforced members and leads to gross under-estimation of expected deflections in those cases. Long term multipliers that allow for compression reinforcement effects cause similar problems. Combine the 2 with a lack of understanding or output from analysis software and you get gross under estimates of expected deflections which lead people to distrust calculated deflections.

 

[IDS]

Also, is anyone going to the Gilbert seminars 'Cracks and Crack Control in Concrete Structures' in Aus run by the concrete institute this month? Gonna get my ticket tomorrow

http://www.concreteinstitute.com.au/Cracking-and-Crack-Control.aspx

Not just Ian Gilbert talking about how to do the calcs, but also Michael van Koeverden talking about how to make concrete behave nicely and follow the calcs once you have done them.

Sydney people have missed it though, you'll have to go to Melbourne or Brisbane.

Doug Jenkins
Interactive Design Services

http://newtonexcelbach.wordpress.com/

 

[KootK]

If you are going to control it all by adjusting depth, then there must be an appropriate width that should be used. What is it?

It would be whatever it takes to resist the moment in conjunction with the selected depth.

Though not economical, width can be used to control the steel ratio to suit your initial requirement of limiting the steel ratio to less that .5.

Sure, one could go out of their way to produce a horrendously inefficient beam for which the 0.5_rho_b check would be unsuitable. As I said above, however, I don't see rare exceptions to a rule of thumb as being valid justification for wholesale abandonment of the rule of thumb. It's also important to remember that I'm a fairly capable engineer/human and it's not as though I'm putting my brain on ice while I'm performing these checks. If I see something stupid, I'll flag it as stupid no matter what my rule thumb tells me.

The paper you quoted a section out of required a steel ratio = .5. NOT <= .5

Is there any doubt in your mind that was a misprint? There's not a shred of doubt in my mind. It's hard to imagine that Dr. Fanella would intend for us to be designing all of our beams to exactly 0.5_rho_b.

Our argument is that members with significantly lower steel ratios than .5 often have deflection problems, even if designed to the simplified code deflection rules (not simple span/depth ratios which should be removed from all codes).

I certainly accept that argument and have learned a good deal about it through the course of this discussion and my related Googling. That said, I still do not accept the notion that reinforcement ratio is an irrelevant parameter when it comes to assessing deflection. Unless I have misread our discussion woefully, it seems to me that we agree that reinforcement ratio checks will correlate well with acceptable performance over a fairly broad range of application.

Also the projects I have in mind are not just Australia. Also UK (worst case), Middle East, and USA and Canada.

Certainly, it was not my intention to single out Australia as the only place on the globe where deflection problems arise. Still, I find it curious that:

1) I'm the only non-Aussie involved in this thread.
2) When I Google this issue, most of what is returned comes from Australian sources.

Did you guys experience some extraordinary deflection problems at some point in the past? Or do you simply benefit from having a local academic that has made this stuff her life's work?

I like to debate structural engineering theory -- a lot. If I challenge you on something, know that I'm doing so because I respect your opinion enough to either change it or adopt it.

 

[rapt]

Kootk
Answering your points in order (briefly in most cases)

1 That is the problem if there is a depth limitation, someone can control it with a wider beam - - we all agree this is illogical but it happens

2 See 1

3, No I do not think it was a misprint and I would not make that assumption. I would fined out from the author or use it if It fitted in with my observations.

5 With our history in PT design using Partial Prestress in Australia since the early 1970's, we have had to do proper deflection checks, allowing properly for cracking, tension stiffening, creep and shrinkage for PT design. So our designers have been exposed to those types of calculations for a long time. The PT industry in the USA went a different way and have not until recently made any attempt to do proper deflection calculations (until RAM Concept was basically forced to do it to sell internationally).
Because Australian designers were exposed to this for PT design and the same design tool was used for RC design (RAPT), they then did the same thing for RC design. Basically an L/D ratio is the starting point in deflection design in Australia, not the final design requirement.

Looking at other countries, UK and all countries using BS8110 essentially base all of their deflection calculations on L/D ratios, hardly ever calculating a real deflection, so they do not know what they are getting.
USA and countries designing to ACI318 basically use L/D ratios and for RC and PT design where they do calculate deflections still use long term deflection multipliers for even for PT design even though the ACI code specifically says not to.
As well, investigations into buildings with deflection problems for designs done by simplified methods by several people like IDS, Peter Taylor, Ian Gilbert and others showed a lot of the deflection problems with buildings designed using simplified deflection design procedures.
That is not to say that all Australian concrete designs are done properly and do not have deflection problems, but there is a greater awareness here of the shortcomings of the simplified methodologies and the solutions.