Mathematical modelling of heating of air in enclosure 1

[Miran Fernando]

Hello all,

I’m looking for some guidance on a calculation that I’m trying to do. I posted a similar recent thread titled 'Heating of air in enclosure - calculation and experiment discrepancy'. This question is in relation to the same device as the one in that other thread, but the enclosure is different and does not concern an experiment that I have done, at least not yet.

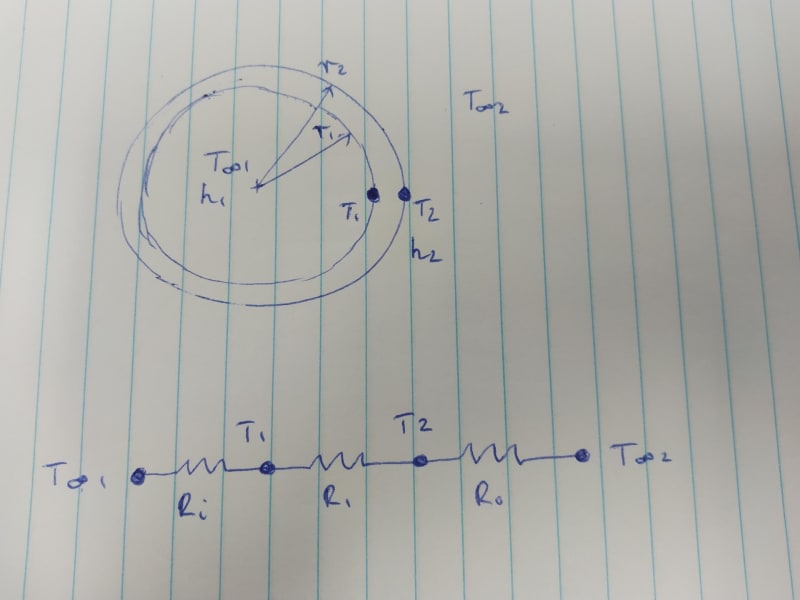
I have designed a small sealed cylindrical enclosure containing air and a small water-cooled device that needs to be kept cool in hot ambient conditions. I have a cooler that has around 75 W capacity to cool the device. The device’s peak power usage is around 60 W, as listed on the device datasheet and confirmed using a power meter (thanks to suggestion by IRStuff, EdStainless, and MintJulep in the other thread). The external ambient air temp is 40 °C. The enclosure is exposed to direct sunlight.

I would like to calculate what the air temperature is inside of the enclosure given:

Ambient air temperature is 40 °C,
Sunlight is incident on the enclosure,
Cooler liquid set point is 25 °C,

Some of the assumptions that I’m making are:

1. Half of the total surface area is exposed to solar irradiance 1000 W / m^2,
2. If the cooler liquid set point is 25 °C then the initial air temperature inside of the enclosure is also 25 °C,
3. All of the power dissipated by the device (60 W) is removed by the cooler, so any remaining capacity (75 – 60 = 15 W) is used to remove heat entering the enclosure from the outside environment,

My first thought on how to do this was to model it as a steady state problem and say that 'Energy in = Energy out' and rearrange to solve for the internal air temperature, but that conflicts with my second assumption (the assumption being that this is actually a transient problem and not steady state). I don’t think I’ve ever done a transient problem like this before. I calculated the Biot number to see if using lumped system analysis was appropriate, but I get a value much higher than 0.1 so it seems I should not use that method.

My second thought on how to do this was to create an expression saying 'Solar energy in = Convective energy out' to get to the internal air temperature when the system has reached steady state. I am again applying a steady state assumption, where the energy gained from solar irradiance equals the energy lost by convection of the enclosure to the surroundings. But I don't think that this takes into account the heat conducted through the walls of the enclosure into the internal air.

About the clearest thinking I have on this is that there is 15 W left of cooling power available. If the power entering the enclosure from outside is greater than 15 W, the air inside will begin heating up and the design needs to change.

I’m really not sure about how to proceed and would very much appreciate some guidance.

 

[IRstuff]

If you are interested in the steady-state air temperature, which presumably is the case, then this is not a transient problem.

You say small, but not how small, and you've not stipulated what color the cylinder is; white is better, but that might degrade over time. Also, is there wind for convection, or is it only natural convection?

In any case, you can brute force a first order solution using resistors to model each thermal interface and solve the simultaneous equations for the various nodes.

TTFN (ta ta for now)
I can do absolutely anything. I'm an expert!

https://www.youtube.com/watch?v=BKorP55Aqvg

faq731-376 forum1529 Entire Forum list

http://www.eng-tips.com/forumlist.cfm

 

[Miran Fernando]

If you are interested in the steady-state air temperature, which presumably is the case, then this is not a transient problem.

I want to estimate what the air temperature inside of the enclosure will reach given an initial temperature of 25 °C, when exposed to 40 °C ambient air and direct sunlight. If it gets too high then the design might need to change, or be scrapped altogether. If the air temperature changes, doesn’t that mean steady state can’t be assumed?

Assuming I’m not understanding and this can be considered a steady state problem:

You say small, but not how small, and you've not stipulated what color the cylinder is; white is better, but that might degrade over time. Also, is there wind for convection, or is it only natural convection?

The dimensions are:

Outside diameter = 200 mm
Inside diameter = 195 mm
Outside height = 175 mm
Inside height = 171 mm

I don’t have a choice with regard to the colour, it is drab olive. There is no wind, the air is stagnant so natural convection only. At this stage the material is ABS.

The device being enclosed is a cube that is about 100 mm to a side, so the actual mass of air is tiny.

In any case, you can brute force a first order solution using resistors to model each thermal interface and solve the simultaneous equations for the various nodes.

Okay I have calculated thermal resistance networks before. I think the first step should be calculating the external temperature of the enclosure given it is exposed to the sun:

E[sub]gained[/sub] = E[sub]lost[/sub] or αA[sub]s[/sub]q[sub]sun[/sub] = h[sub]combined[/sub]A[sub]s[/sub](T[sub]s[/sub] – T[sub]∞[/sub])

Where:

- A[sub]s[/sub] is surface area of enclosure (m^2)
- α is absorptivity
- h[sub]combined[/sub] is the combined convection and radiation heat transfer coefficient (W / (m^2 ∙ °C))
- q[sub]sun[/sub] is the solar irradiance (W / m^2)

I will assume 0.9 for absorptivity, that’s a bit high but the drab olive may be quite dark and matte. I will assume 50 W / (m^2 ∙ °C) for h[sub]combined[/sub], I’ve no idea what a good value for that would be but 50 was in a textbook. I will assume 1000 W / m^2 for solar irradiance, again probably a bit high but I am trying to be conservative.

A[sub]s[/sub] cancels, and rearranging for T[sub]s[/sub] gives:

T[sub]s[/sub] = T[sup]∞[/sup] + α(q[sub]sun[/sub]/h[sub]combined[/sub]) = 58 ׄ°C

Here is the schematic and thermal resistance network:

Where:

- T[sub]∞2[/sub] is the ambient air temperature, 40 °C,
- h[sub]2[/sub] is the convective heat transfer coefficient on the outside surface,
- T[sub]2[/sub] is the enclosure external surface temperature, 58 °C (calculated above),
- T[sub]1[/sub] is the enclosure internal surface temperature,
- T[sub]∞1[/sub] is the internal air temperature, initially 25 °C,
- h[sub]1[/sub] is the convective heat transfer coefficient on the inside surface,
- r[sub]1[/sub] is the internal enclosure radius,
- r[sub]2[/sub] is the external enclosure radius,
- R[sub]i[/sub] is the thermal resistance of the internal surface against heat convection,
- R[sub]o[/sub] is the thermal resistance of the external surface against heat convection,
- R[sub]1[/sub] is the thermal resistance of the enclosure material again heat conduction,

The heat transfer equation is:

Q = (T[sub]∞1[/sub] - T[sub]2[/sub]) / R[sub]tot[/sub]

I can rearrange and solve for T[sub]∞1[/sub] but I don’t know the rate of heat transfer. I guess I can just assume that half to a third of the enclosure is in direct sunlight and apply the solar irradiance to half to a third of the enclosure surface area, but that doesn’t seem right to me.

 

[IRstuff]

Your model is partially complete; it seems to be lacking details about the "water cooled" device and how it interacts with the air inside the container. It also doesn't seem to include any radiative transfer back to the atmosphere; typically, the absorbed solar load raises the surface temperature, resulting in thermal radiance away from the enclosure. Enclosures with such conditions might be highly polished and reflective internally, to push more radiation to the outside by having a drastic reflectance difference inside vs. outside.
> Is the water cooling completely enclosing the device?
> Is the device insulated?

Depending on the answers, the air temperature inside the enclosure might be moot; if, say, the device and its cooling were within a Styrofoam enclosure, there might be little or negligible heat transfer outside of the Styrofoam.

TTFN (ta ta for now)
I can do absolutely anything. I'm an expert!

https://www.youtube.com/watch?v=BKorP55Aqvg

faq731-376 forum1529 Entire Forum list

http://www.eng-tips.com/forumlist.cfm

 

[Miran Fernando]

Apologies for not replying sooner.

Your model is partially complete; it seems to be lacking details about the "water cooled" device and how it interacts with the air inside the container.

The device has an inlet and outlet for coolant and some type of internal heat exchanger, thermoelectric I think. At the moment, I'm just assuming that all heat generated by the device is removed by the cooling loop.

It also doesn't seem to include any radiative transfer back to the atmosphere; typically, the absorbed solar load raises the surface temperature, resulting in thermal radiance away from the enclosure

When I calculated the heat of the external surface of the enclosure, I did so by assuming that when exposed to the solar load eventually the heat entering the enclosure will equal the heat leaving it. I'm quoting myself below, but the h[sub]combined[/sub] term is the combined convection and radiation heat transfer coeffient:

I think the first step should be calculating the external temperature of the enclosure given it is exposed to the sun:

Egained = Elost or αAsqsun = hcombinedAs(Ts – T∞)

Where:

- As is surface area of enclosure (m^2)
- α is absorptivity
- hcombined is the combined convection and radiation heat transfer coefficient (W / (m^2 ∙ °C))

Enclosures with such conditions might be highly polished and reflective internally, to push more radiation to the outside by having a drastic reflectance difference inside vs. outside.

I don't understand this. Polishing and making the internal surface reflective prevents heat conduction through the enclosure from the outside?

> Is the water cooling completely enclosing the device?
> Is the device insulated?

It is completely enclosing the device. Due to the very small size of this enclosure, insulating the device isn't really practical.

 

[IRstuff]

Polishing and making the internal surface reflective prevents heat conduction through the enclosure from the outside?

Yes, because the conducted heat cannot be radiated to the interior, although it can be convected. The complete jacketing with water means that any convected/radiated heat from the outside will be absorbed by the coolant.

TTFN (ta ta for now)
I can do absolutely anything. I'm an expert!

https://www.youtube.com/watch?v=BKorP55Aqvg

faq731-376 forum1529 Entire Forum list

http://www.eng-tips.com/forumlist.cfm

 

[Miran Fernando]

Yes, because the conducted heat cannot be radiated to the interior, although it can be convected

Hmm yes I suppose that it would it. That is an excellent suggestion and something that could be easily implemented, thank you for pointing it out.

I will re-check my calculations and post the internal air temperature that I calculated.

 

[Miran Fernando]

I will re-check my calculations and post the internal air temperature that I calculated.

Assuming 1/3rd of the enclosure surface area is exposed to 1000 W/m[sup]2[/sup] and using the expression T[sub]∞1[/sub] = (α⋅q[sub]sun[/sub]⋅A[sub]s[/sub] - 10)⋅R[sub]tot[/sub] + T[sub]s[/sub], I get an internal air temperature of approximately 85 °C. A[sub]s[/sub] here is the surface area of the cylinder, R[sub]tot[/sub] is the sum of the conductive and convective thermal resistances, 10 is subtracted because there is 10 W of cooling overhead left provided by the chiller to cool the internal air.

I suppose this number sounds reasonable. The walls are pretty thin, and the mass of internal air is pretty small.

I'd be grateful to hear any opinions on the number I've calculated.

 

[IRstuff]

That's too high, in general. While exposed surfaces do get hot from the sun, they don't always get to the point of being able to cook eggs; that often requires extremely hot air temperatures to start with. You seem to be dumping all the heat from the sun into the enclosure, any I again do not see any heat loss from the surface back to the ambient environment.

TTFN (ta ta for now)
I can do absolutely anything. I'm an expert!

https://www.youtube.com/watch?v=BKorP55Aqvg

faq731-376 forum1529 Entire Forum list

http://www.eng-tips.com/forumlist.cfm

 

[Miran Fernando]

While exposed surfaces do get hot from the sun, they don't always get to the point of being able to cook eggs

Just to clarify, the 85 °C is the temperature of the air inside of the enclosure that I have calculated, not the outside surface of the enclosure. I calculated the external surface temperature of the enclosure in the original post, and it was about 58 °C. Seems reasonable given that the enclosure is in direct sun and is a dark colour.

You seem to be dumping all the heat from the sun into the enclosure, any I again do not see any heat loss from the surface back to the ambient environment.

To summarise how I calculated all of this again the steps I followed are:

1. Energy gained = energy lost. Energy gained is energy from the sun. Energy lost is by convection and radiation, I used a combined heat transfer coefficient that takes into account heat transfer via radiation and heat transfer via convection (the combined heat transfer coefficient could be way off though.) I rearranged my expression to find the temperature of the outside surface of the enclosure, 58 °C.

2. Used the thermal resistance network method to calculate the internal air temperature of the enclosure. I found the external surface temperature earlier and have all of the information needed to calculate the internal air temperature from heat conducted through the walls.

But! I still feel like 85 °C is a bit high.

 

[IRstuff]

It's not physically plausible. Given the 25C water temp, an 85C air temp would mean the enclosure surface temp would be upwards of 100C, which is not plausible.

TTFN (ta ta for now)
I can do absolutely anything. I'm an expert!

https://www.youtube.com/watch?v=BKorP55Aqvg

faq731-376 forum1529 Entire Forum list

http://www.eng-tips.com/forumlist.cfm

 

[Miran Fernando]

It's not physically plausible. Given the 25C water temp, an 85C air temp would mean the enclosure surface temp would be upwards of 100C, which is not plausible.

The device being cooled isn't submerged in water, it has hoses running in and out of it carrying water through an internal heat exchanger. The device itself is just surrounded by empty air inside of the enclosure.

I have been assuming that all heat generated by the device will be removed by the cooling system. Isn't this basically the same as saying the enclosure is empty? There will be a greater mass of air inside due to the absence of the device, but it won't be much greater.

I should be able to find a closed cylinder that is similar in size and material to the one I am considering, put it in direct sun and measuring the internal air using a thermocouple of thermistor.

 

[IRstuff]

This is what I would do for an empty enclosure

TTFN (ta ta for now)
I can do absolutely anything. I'm an expert!

https://www.youtube.com/watch?v=BKorP55Aqvg

faq731-376 forum1529 Entire Forum list

http://www.eng-tips.com/forumlist.cfm