Maximum allowable reinforcement strain

[breaking_point]

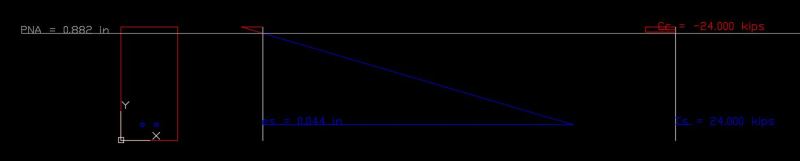
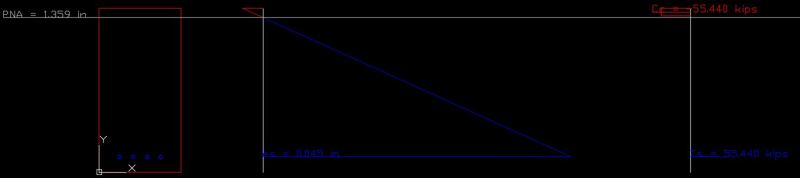
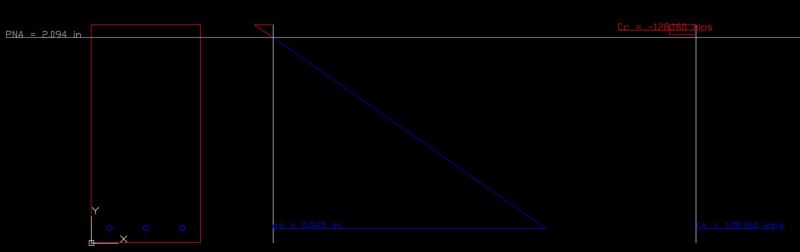
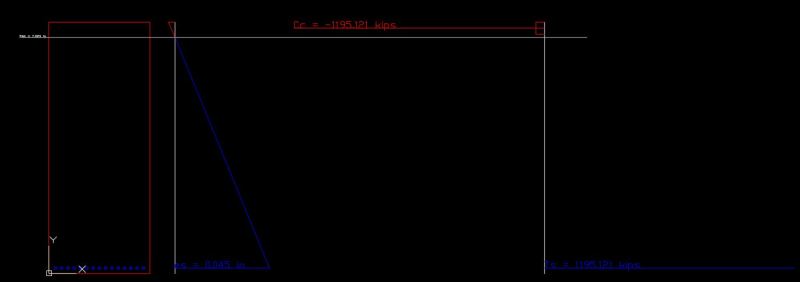
Hey. So I'm looking at RC beam design, and I'm aware that beams are typically designed to be tension controlled, i.e. at the concrete rupture strain (0.0035), the steel should be beyond the yield strain. I've read that it is good to aim to have the steel strain at around 0.005-0.008 when the concrete ruptures. What I want to know is, what is the maximum allowable strain in the steel? What strain does steel fracture at? I can't find a definitive answer.

Thanks

 

[sticksandtriangles]

I'm interested to see where this goes as well. I do not know if there is an answer to this.

 

[Celt83]

I was getting into this topic a couple months ago...didn't get to any real conclusions other than the stress-strain curves I could find all went up to strains around 0.13-0.2

I ran a bunch of different beam configurations and pretty much was always ending up with strains in the 0.0x to 0.00x range so well under the 0.1x range for rupture on diagrams I was finding. It was seeming that the minimum steel ratios and standard equations where set up to pretty much guaranty the steel isn't getting close to the rupture strain when the concrete reaches its rupture strain of 0.003-0.0035 but is hovering around the 0.038 to 0.045 range. See some examples below all with min steel and f'c of 5,000: 8x8, 12x12, 8x16, 12x24, 18x36, 12x120, 48x120

those images got horribly compressed - attached zipfile has the uncompressed images where you can read the numbers.

Open Source Structural Applications:

https://github.com/buddyd16/Structural-Engineering

 

[Tommy385]

Have a look at stress-strain diagram. Depending on the code, around 2%

 

[breaking_point]

@Celt83, Excellent! Thanks for the response. I figured that the minimum reinforcement equations would typically limit the steel from developing too much strain, but couldn't find any solid data.

 

[Celt83]

onemore try on the zip file:
Link

Open Source Structural Applications:

https://github.com/buddyd16/Structural-Engineering

 

[Celt83]

120x120 beam

pretty much get an es = 0.044-0.045 strain at minimum steel in every case so far.

Open Source Structural Applications:

https://github.com/buddyd16/Structural-Engineering

 

[Celt83]

that is for single reinforced once you start getting into compression bars in the bigger beams you start getting into higher steel strains. Which makes sense as compression steel will reduce the pna depth increasing the distance from the pna to the extreme tensions steel and since es and ec are proportioned based on their distance from the NA the strain in the steel starts exceeding the 0.045 singly reinforced number. The larger your depth and reinf. ratio is the higher the delta in the pna location with the addition of compression steel.

Skin reinforcement starts coming into play though which included or not in anaylsis can greatly impact the steel centroids and help rein that extreme strain back in, although at the expense of shear strength when considered.

Open Source Structural Applications:

https://github.com/buddyd16/Structural-Engineering

 

[Celt83]

dwg's for 120x120 w and w/o skin bars, again minimum steel only and F'c=5ksi Fy=60ksi 1.5" cover and #4 stirrups.

skin bars

w/o skin bars

Open Source Structural Applications:

https://github.com/buddyd16/Structural-Engineering

 

[rapt]

This is a problem that has existed in design codes for years.

There are no problems with higher ductility reinforcing products such as those used for Earthquake design which are normally in the order of 10 - 15% strain.

But for normal reinforcing steels there are reasons for limits but the design codes do not apply them.

At a minimum reinforcement level equivalent to the cracking moment of the section, the strain in the steel will be in the order of 5%. As the reinforcing level increases, this strain reduces.

If you add the effects of strain localisation at a crack, this strain actually increases so it is significantly higher than 5%.

For the lower ductility reinforcement products like welded wire products, European Class A (at 2.5%), Australian Class L (at 1.5%)these actual strain levels are a real problem and need to be addressed by design codes.

Unfortunately it always seems to be in the too hard basket. The lower the ductility of the reinforcement, the higher the minimum reinforcement requirement should be to account for this.

 

[cooperDBM]

I agree that this is a blind spot in the codes. I ran into it last year reviewing prestressed double tees. At ultimate the wide flange led to a shallow depth to the neutral axis and a strain at the strands of over 10% in theory. The actual rupture strain of strand is 5-7%. I recalculated with a strain limited to the minimum (proof) tensile strain in the ASTM standard for strand (3.5%). The strain rate (curvature) is then based on the tensile limit instead of the compression limit. Because the stress-strain curve at rupture is shallow the decrease in the ultimate flexural capacity was small.

 

[bkal]

If you are using Eurocodes, EC2 (EN 1992-1-1, Annex C) specifies values for strain at maximum force for three classes of bars; A >= 2.5%, B >=5% and C >= 7.5%.

 

[rapt]

bkal

They are the minimum strain requirements, but Eurocode does not require you to limit strain in the steel due to flexure if you use an elastic/plastic stress strain diagram. You only have to limit the strain if you allow for increasing strain past yield.