Modeling of Single Reinforced Interior Column - Buckling

[lexeng18]

Hi all,

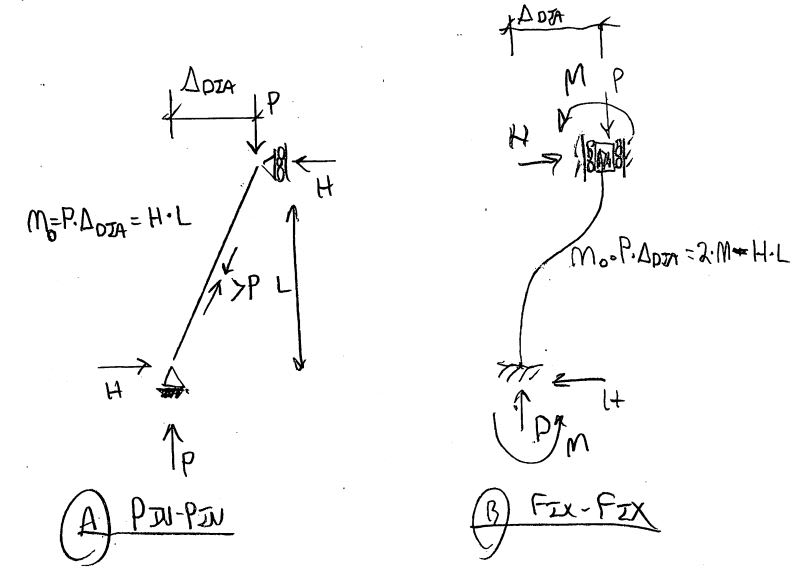
I have a situation where I need to reinforce a few interior columns in a large warehouse for new axial load due to large RTUs and associated snow drifts. The columns are pinned at the base by a standard baseplate and restrained laterally at the top by a flexible diaphragm. Due to obstructions of process equipment, I designed partial height plate reinforcement for these columns. The plate reinforcement extended from FFE to 14'-0", while the total column length is 30'-0". I originally approached the design as a stepped column analysis. I recently went to compare the results of my stepped column analysis to that of a linear buckling analysis from RAM Elements.

I understand that the results from the linear buckling analysis need to be modified in some way to account for things such as initial imperfections and inelastic buckling behavior. However, the proper implementation of these effects are causing me confusion. I have seen examples on this forum of backing out an appropriate K value from the Euler buckling equation if the buckling load is known, however this is only possible if the cross section is constant along its length. In my case, it varies.

Therefore I believe the only way to properly design these columns is to use the DAM procedures. I have no issue reducing the material stiffness of the column by 0.8 * tau_b which of course reduces my buckling load. However, the modeling of initial imperfections is causing me issues. If I attempt to use notional loads, the buckling load is unaffected by the small lateral force that I am applying at mid-height of the column. Is the proper procedure to use the beam-column interaction equations to check the column for unity considering the buckling load with a reduced stiffness material property and the moment due to the notional load? If so, I'm not sure how the fact that the column is partially reinforced would affect the flexural capacity of the column. What I mean is, while the software is accurately capturing the effects of varying stiffness cross section for the axial condition via the buckling analysis, it is not considering it for the flexure condition.

Am I going about this in the wrong way? Should I be modeling direct displacements in the nodes to account for the initial imperfections in the column instead of notional loads due to these issues?

I have done a great deal of searching on this topic before posting and feel that previous discussions on this topic did not address the varying cross section situation in a way that I understood. I would be happy to provide more information or sketches if it is helpful to facilitate discussion.

 

[KootK]

However how do you know which method is applicable to any given project, or for example when the effective length method is not a good choice?

1) Clips below from the paper that I linked in my last post.

2) All methods are allowed so long as they cover the various aspects of stability in some fashion, so no limits there.

3) For a non-sway column loaded at its ends, K-factor is perfectly sufficient. Only go fancier if you're basically getting DAM on such columns for free from a global analysis.

4) For members in low aspect ratio, concentrically braced lateral frames, K-factor is perfectly sufficient. Only go fancier if you're basically getting DAM on such columns for free from a global analysis.

5) In the space that is rectangular/tiered building design, it's really moment frames where one may want to go with the more modern methods for reasons other than expediency. The first order amplification method is really a simplified version of DAM so, in this respect, their range of applicability is similar. Both of these methods consider P-DELTA and P-delta explicitly which tends to lead to the greater accuracy that is desired when both of those things are present in significant measure.

6) A truly wonderful feature of DAM is that it can be simply and uniformly applied, in a highly automated fashion, to situations of almost any level of complexity. DAM was purpose-built to play well with our FEM toys. My prediction for the future is that DAM will take over. I see first order amplification dying off entirely and K-factor being retained only for the simplest of scenarios.

7) For truly complex frames outside the range of rectangular/tiered building stuff, DAM really shines. It will be more accurate than many of the other methods and will be capable of capturing things like the tendency for one lateral element to brace or be braced by it's neighbors at various points in a structures load history. Additionally, the simplicity and uniformity of applying DAM make it a method that, in my estimation, inspires confidence in highly complex situations highly prone to designer error.

 

[KootK]

I guess to put it bluntly, first order vs second order vs P-delta terms all seem to run together in my head (is P-delta in and of itself not a second order effect?) and I have a hard time separating one from the other because I only have a fundamental understanding of Chapter E3 and the ELM.

In this context, I believe that a method is second order if it takes into account the tendency of either nodal or intra-member deflections to amplify other force actions (mostly moments). That's it. As indicated in the table that I posted previously:

1) All of the methods account for second order effects.

2) K-factor and DAM require the direct calculation of second order effects. It is only the first order amplification method that does not. Rather, the first order method estimates the second order effects based on first order analyses.

I do not believe the original designers paid any attention to the fact that the diaphragm deflection under wind or seismic forces warrants additional consideration for the interior columns.

I all but guarantee tat was the case.

In my mind, if I imagine these columns under full axial load, then the top of the column deflects with the diaphragm under some wind load, how does this not cause a bending moment in the column equal to the axial load times the diaphragm deflection?

It absolutely does induce a moment. That moment is just expressed differently for columns having different end fixities as shown below.

 

[BAretired]

In KootK's (A) above, i.e. pin/roller, no moment is introduced in the column, but there is a story moment PΔ = HL.

BA