Pretty Girl
Structural
- Nov 22, 2022
- 148
This was taken from the book "Reinforced concrete design to eurocodes - by prab bhatt" at page 353. I'm trying to understand this equation. And trying to calculate moment capacity for a compression area of a uni-axial concrete column.
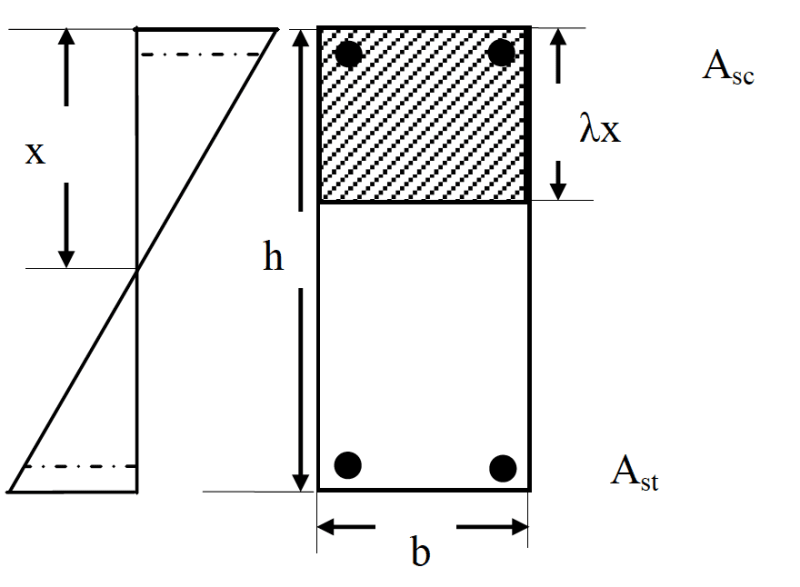
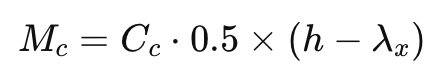
In that equation, the moment capacity should be "Cc x lever arm". Lever arm is mentioned as "0.5 x (h-lambda x". Isn't that lever arm should be the centroid from the compression block of concrete to the centroid of the tensile reinforcement? but that "0.5 x (h-lambda x" never reaches the tensile r/f. So I'm confused.

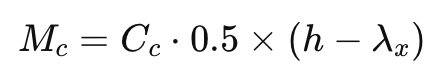
In that equation, the moment capacity should be "Cc x lever arm". Lever arm is mentioned as "0.5 x (h-lambda x". Isn't that lever arm should be the centroid from the compression block of concrete to the centroid of the tensile reinforcement? but that "0.5 x (h-lambda x" never reaches the tensile r/f. So I'm confused.