Hello,
I wanted to see if anyone has a good reference of how to determine the per unit impedance of 3 Winding XMFRs ?
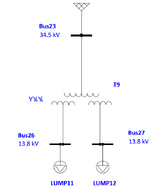
I have a very basic fictious system shown below and I'm trying to determine how to by hand calculate the LG and 3 Ph fault currents at Bus 23, 26, and Bus27 but I'm not clear on how to represent the 3 winding XFMR.
In the software package whats entered is :
MVA per winding
Zps, Zpt and Zst plus the X/R ratios.
Lets assume each lump load represents a 100% motor load
I found the following equations but cant seem to match the software's result which tells me im making a mistake when finding the three impedances they propose as :

 www.linkedin.com
www.linkedin.com

Where im stuck is how to determine which of the three equations above to use to determine the equivalent impedances for the positive and negative sequence networks.
Something tells me that depending on the fault location some combination of these impedances applies.
Any guidance on this would be much appreciated.
I wanted to see if anyone has a good reference of how to determine the per unit impedance of 3 Winding XMFRs ?
I have a very basic fictious system shown below and I'm trying to determine how to by hand calculate the LG and 3 Ph fault currents at Bus 23, 26, and Bus27 but I'm not clear on how to represent the 3 winding XFMR.

In the software package whats entered is :
MVA per winding
Zps, Zpt and Zst plus the X/R ratios.
Lets assume each lump load represents a 100% motor load
I found the following equations but cant seem to match the software's result which tells me im making a mistake when finding the three impedances they propose as :

CALCULATION OF LINE-GROUND FAULT CURRENTS IN A THREE-WINDING TRANSFORMER (WYE-WYE WITH BURIED DELTA)
AUTHORS: Nikhil Ardeshna P.Eng, P.
 www.linkedin.com
www.linkedin.com

Where im stuck is how to determine which of the three equations above to use to determine the equivalent impedances for the positive and negative sequence networks.
Something tells me that depending on the fault location some combination of these impedances applies.
Any guidance on this would be much appreciated.
