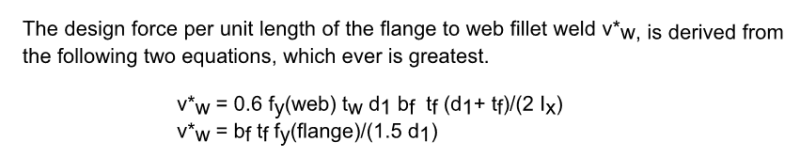iStruct
Structural
- Jan 2, 2020
- 12
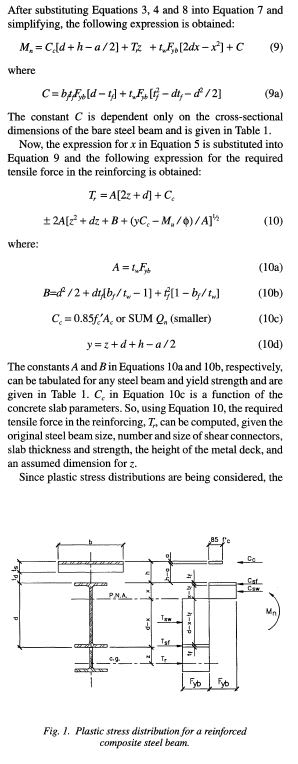
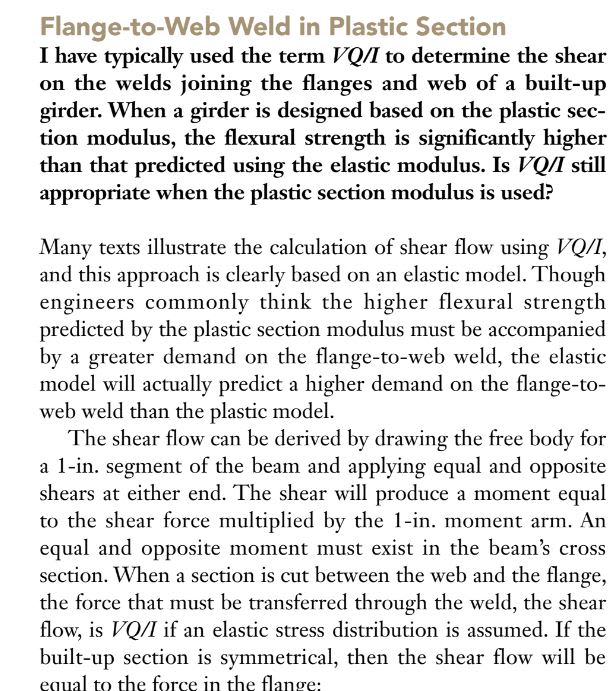
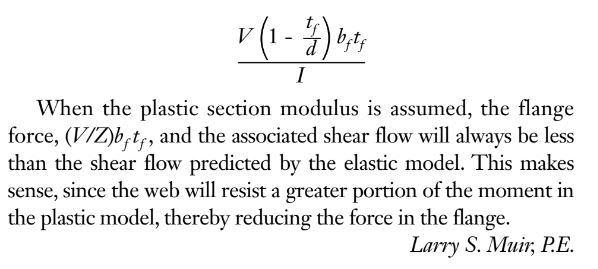
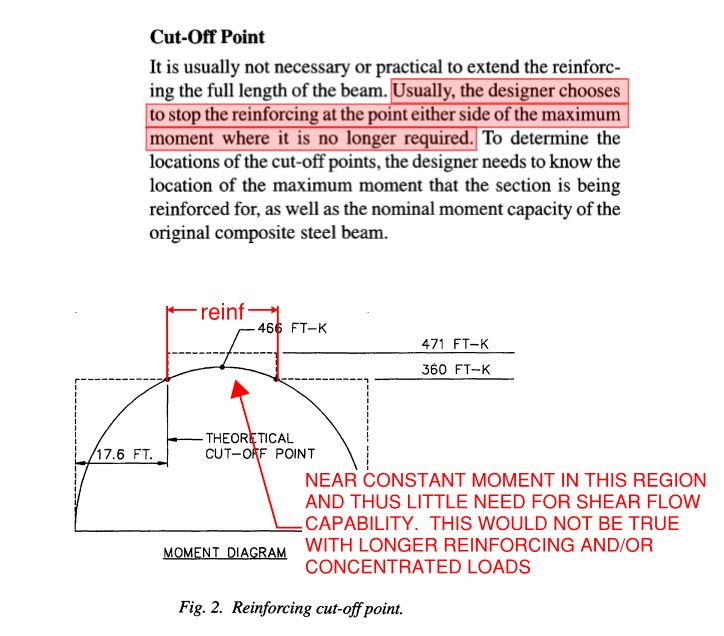
I have done a lot of steel retrofit and usually this involves welding a plate to a beam or similar. Often it is more economical to use the full plastic capacity to get the plate size if top flange is braced.
If I am using the plastic capacity of the plate it seems wrong to me to then use VQ/I to size the welds considering I need to transfer more through the weld than if it were just elastic bending. I am thinking that the welds would be sized similar to how studs in a composite beam are sized/spaced. Am I crazy? I have seen some text book examples where VQ/I is used where it is expected the beam develop full plastic capacity.
If I am using the plastic capacity of the plate it seems wrong to me to then use VQ/I to size the welds considering I need to transfer more through the weld than if it were just elastic bending. I am thinking that the welds would be sized similar to how studs in a composite beam are sized/spaced. Am I crazy? I have seen some text book examples where VQ/I is used where it is expected the beam develop full plastic capacity.