Plastic hinge in reinforced concrete 2

[Ilight]

Hey everyone:

So for typical reinforced concrete member, is there a way to calculate the moment at which plastic hinge will form? I've heard that it is similar to flexural moment, with strain in extreme fibre being 0.003.

Thank you!

 

[dauwerda]

I'm not sure I am understanding your question correctly, but a plastic hinge will form in a beam when the tension reinforcement yields (assuming the beam is designed correctly and the steel yields before the concrete crushes).

 

[BAretired]

Agree with dauwerda.

BA

 

[BAretired]

So for typical reinforced concrete member, is there a way to calculate the moment at which plastic hinge will form? I've heard that it is similar to flexural moment, with strain in extreme fibre being 0.003.

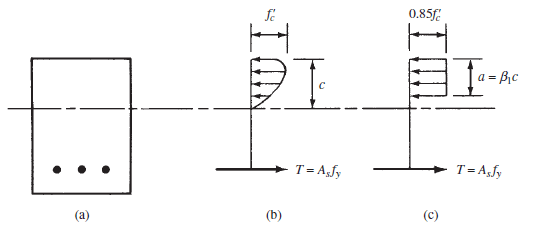
EDIT: The maximum strain assumed to exist at the extreme compression fibre is 0.003 (some say 0.0035). Balanced strain conditions exist when the tension reinforcement reaches yield strain just as the concrete reaches maximum strain. An equivalent rectangular concrete stress distribution known as the Whitney stress block is used in North America to approximate the actual stress-strain curves used in some jurisdictions.

When the strain in the extreme fibre reaches a value of 0.003 (some say 0.0035), it is assumed that the stress ceases to increase linearly, in fact it is assumed (at least in N. America) that the stress in the concrete remains constant as strain continues to increase. It is an approximation of concrete behaviour leading to a rectangular stress block at ultimate moment as opposed to a parabolic stress block used in some parts of the world.

BA

 

[ThomasH]

BAretired,

Your statement surprised me a bit.

In the Eurocode there are a few different stress-strain relations. None of them are linear up to 0.003 (or 0.0035 which is used in that code). But assuming that a "normal" has E-modulus at approximately 30 GPa. With sigma = E * epsilon that would mean linear until 0.003 * 30 GPa = 90 MPa, that is not normal concrete for me. The simplest, bilinear relationship, in the Eurocode uses 0.00175 for linear proportionality limit. The more complex functions have no truly linear section except when reaching ultimate stress. Ultimate strain, 0.0035, means crushing of concrete, basically failure.

The way I read codes from the US, like ACI 318, the numbers are similar, and 0.003 means "crushing of the concrete".

Have I misunderstood something in the US codes?

Thomas

 

[KootK]

1) What is your application here? Seismic design plastic hinge or something else?

2) For many applications, I'd follow my local code's lead with respect to seismic plastic hinges. Usually this amounts to just using 1.25xfy rather than 0.9fy on the rebar or something like that.

3) If you'll have significant axial load on the section, you may need to consider that as well.

 

[Ilight]

plastic hinge will form in a beam when the tension reinforcement yields

I thought plastic hinge is the point when the member doesn't take anymore moment however it can still undergo rotation with excessive moment being applied. When the steel reinforcement yields (strain at 0.0025), it is in a plastic state, but it can still take an increased moment. Which leads me to think that it is the concrete strain 0.003, which defines plastic hinge formation.

 

[KootK]

Which leads me to think that it is the concrete strain 0.003, which defines plastic hinge formation. ponder

Nope. You want the rebar to yield and the concrete to not crush. Look at the rebar and ensure that your section is under-reinforced to some degree.

 

[IDS]

The increase in design moment capacity after the steel yields is small, so for practical purposes you can take the ultimate moment capacity as defined in the applicable code as the plastic hinge moment.

If you are designing other members not to yield when the plastic hinge forms, the moment needs to be factored up, which is covered in the seismic design provisions of all codes that I know of.

Doug Jenkins
Interactive Design Services

http://newtonexcelbach.wordpress.com/

 

[kissymoose]

When the strain in the extreme fibre reaches a value of 0.003 (some say 0.0035), it is assumed that the stress ceases to increase linearly

BAretired, this isn't correct. We assume the strain increases linearly up to 0.003, as a function of distance from the plastic neutral axis. For stress, ACI 318 considers linear behavior up to 45% of the concrete's ultimate strength. I don't doubt this is what you meant as you mention the equivalent stress block, which represents the total compressive stress/strain relationship of concrete to failure as a constant stress over a smaller depth.

R19.2.2.1 Studies leading to the expression for modulus of elasticity of concrete are summarized in Pauw (1960), where Ec is defined as the slope of the line drawn from a stress of zero to a compressive stress of 0.45fc′.

So ThomasH, for normal weight concrete (145pcf) and say a strength of 6000psi/41.4MPa, we're looking at a modulus of 4415ksi/30.4GPa and strain of 0.0014.

 

[BAretired]

The way I read codes from the US, like ACI 318, the numbers are similar, and 0.003 means "crushing of the concrete".

You are correct. I have corrected my previous post.

BAretired, this isn't correct. We assume the strain increases linearly up to 0.003, as a function of distance from the plastic neutral axis.

Yes, you are correct.

BA

 

[ThomasH]

BAretired
Thanks for clarifying.

As for plastic hinges. Once they have formed you probably need to check the rotational capacity. That is not infinite and the limitation can be the ability to deform/rotate.

Thomas

 

[r13]

I think the parallel between ultimate strength design method and plastic design method ends in both methods consider the entire cross section is at imminent failure under the respective limiting moment (Mn, Mp). Beyond that, the design parameters and approaches are completely different for these two design methods.

 

[IDS]

retired13 - I would say plastic design is a sub-set of Ultimate Limit State design, not a different method. ULS design defines the conditions to be considered in the analysis, but allows different methods of analysis to determine the actions at any section.

Doug Jenkins
Interactive Design Services

http://newtonexcelbach.wordpress.com/

 

[r13]

IDS - I agree both methods (USD and Plastic Design) are under the same universe - limit state (see my first sentence), but the design approaches and parameters are quite different after the similarity (again in agreement with the latter part of your second sentence). Also, the differ in design parameters (As, d in USD, and Z in plastic design) leads me to think these are two different design methods. Please correct me, your further comments are appreciated.