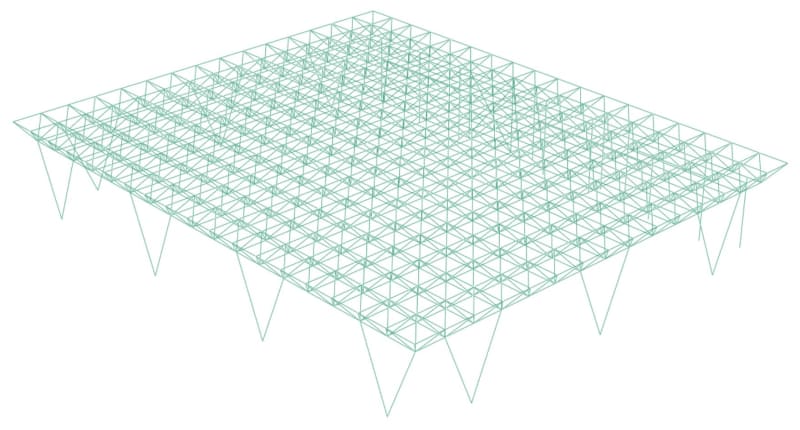
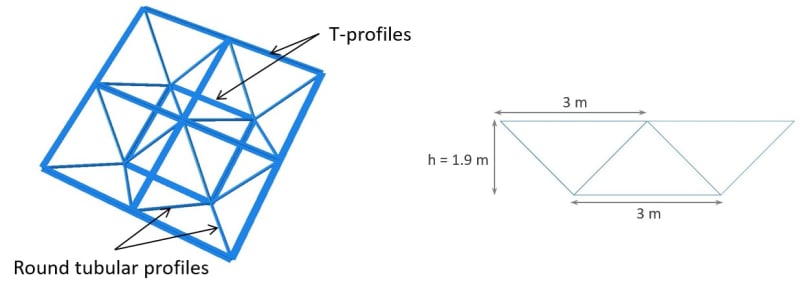
I’m modeling a metallic structure whose roof collapsed under snow weight. I modeled this structure using beam elements and I specify that the roof elements are bolted together (no ball joint).
The calculation is made in static and the snow loading is applied using linear forces.
In small displacements, the code diverges while I have not reached the steel ultimate strength; I get the following error message: “The strain increment is so large that the program will not attempt the plasticity calculation at 171 points. The plasticity/creep/connector friction algorithm did not converge at 55 points.” I tried to increase the time period, to decrease the initial and minimum increment sizes, to increase AI or to reduce the size of the mesh… Nothing works! And in big displacements, the code diverges even earlier! As a result, I can’t perform risk analysis integrating imperfections.
I would appreciate a recommendation on how to improve convergence in small and big displacements?
The calculation is made in static and the snow loading is applied using linear forces.
In small displacements, the code diverges while I have not reached the steel ultimate strength; I get the following error message: “The strain increment is so large that the program will not attempt the plasticity calculation at 171 points. The plasticity/creep/connector friction algorithm did not converge at 55 points.” I tried to increase the time period, to decrease the initial and minimum increment sizes, to increase AI or to reduce the size of the mesh… Nothing works! And in big displacements, the code diverges even earlier! As a result, I can’t perform risk analysis integrating imperfections.
I would appreciate a recommendation on how to improve convergence in small and big displacements?