pumpkinwiz1
Mechanical
Hello, I am trying to solve the laminar sudden contraction case as described in Idelchik's textbook.
For some reason, I am getting a very large negative value for the coefficient.
I have the following variables defined:
Re=1961
F0=1.45e-4 (meters2)
F1=.0018 (meters2)
I am ignoring coefficient due to friction for this case.
I am getting A= -2833 and B=1.2893, which gives the coefficient of -3300, which is wrong.
According to the graph in the textbook, it should give me a coefficient value of ~0.5
I think I might be doing something wrong in the summation:
When there is a sigma sum of aij indices as shown, I was assuming both A and B should result in a scalar value? I think I might be wrong because the equation to solve for the overall coefficient asks for A dot B(1-f0/f1).
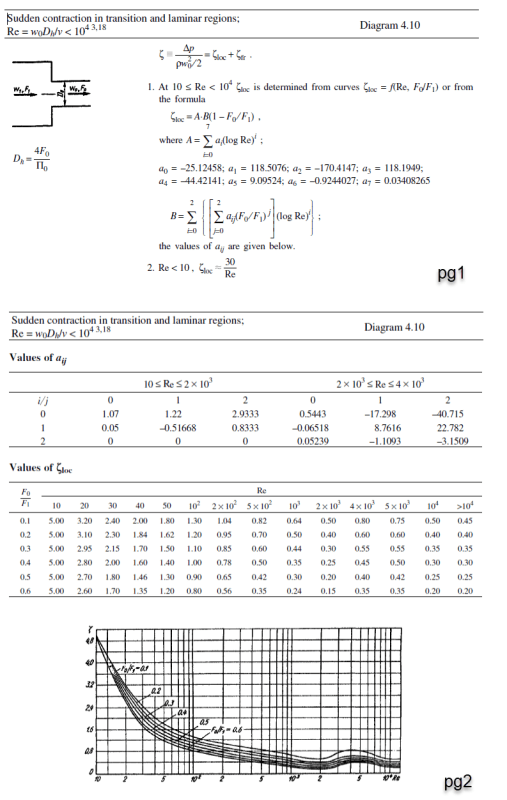
Will appreciate any advice/feedback. Thank you!
For some reason, I am getting a very large negative value for the coefficient.
I have the following variables defined:
Re=1961
F0=1.45e-4 (meters2)
F1=.0018 (meters2)
I am ignoring coefficient due to friction for this case.
I am getting A= -2833 and B=1.2893, which gives the coefficient of -3300, which is wrong.
According to the graph in the textbook, it should give me a coefficient value of ~0.5
I think I might be doing something wrong in the summation:
When there is a sigma sum of aij indices as shown, I was assuming both A and B should result in a scalar value? I think I might be wrong because the equation to solve for the overall coefficient asks for A dot B(1-f0/f1).

Will appreciate any advice/feedback. Thank you!

