fracture_point
Structural
- Mar 7, 2019
- 58
I was doing some additional background reading on ULS design, and found the following quote on wikipedia:
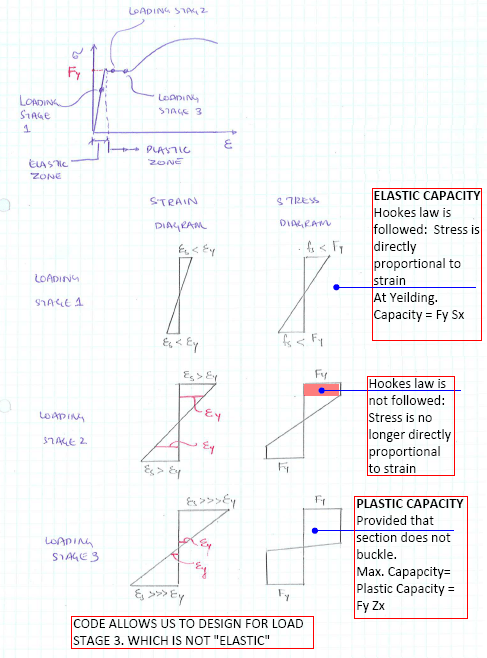
I can't find a reference for the approximation of 15%. We perform sectional analysis of members based on the plastic condition, and these loads are based on partial factors of safety to materials and loads. But I can't find anywhere that provides details about it remaining elastic.
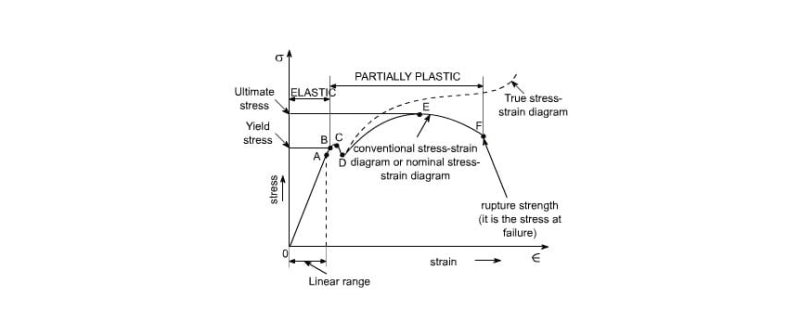
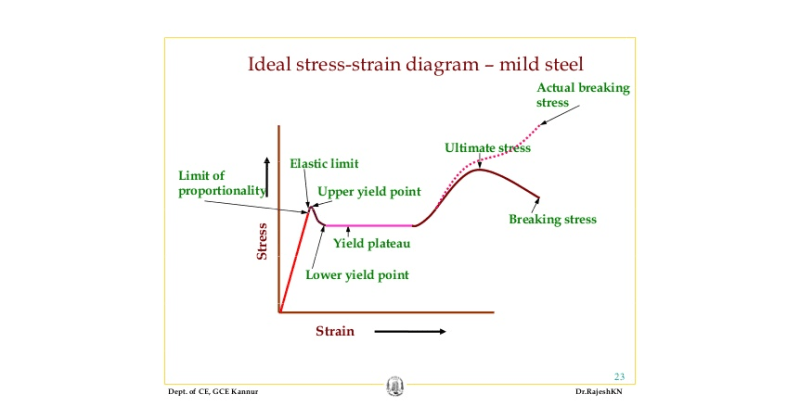
Wikipedia said:The ULS condition is computationally checked at a certain point along the behavior function of the structural scheme, located at the upper part of its elastic zone at approximately 15% lower than the elastic limit. That means that the ULS is a purely elastic condition, located on the behavior function far below the real Ultimate point, which is located deep within the plastic zone.
I can't find a reference for the approximation of 15%. We perform sectional analysis of members based on the plastic condition, and these loads are based on partial factors of safety to materials and loads. But I can't find anywhere that provides details about it remaining elastic.