Reaction forces of a table

[SC83]

All,

I need to check if a table with a motor mounted on top of it will stand still or not under a rotational torque, i.e. starting torque of the motor.
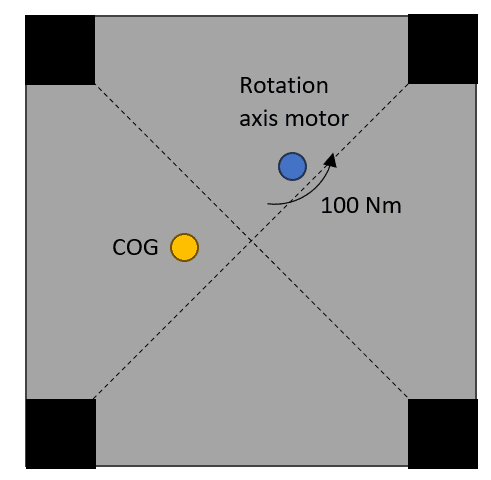
I added a top view of the square table with 4 legs (black). The rotation axis of the motor is indicated with a blue dot and is not in the middle of the table.
The COG of the table with motor and other equipment is indicated with a yellow dot and is also not in the middle of the table, nor in the rotation axis of the motor.
How can I calculate the reaction forces of the 4 legs on the floor under the torque of 100 Nm? I need to check if the table will stand still or not as the legs cannot be bolted in the floor.

 

[PersonalProfile]

The following may assist as a start.

Action:
1. Assume only one off of the legs transfers the action?, and
Considering unknown construction etc tolerances etc.
2. Calculate action using physics etc.
I.e. Consider relocating torque/moment to convenient location, and calculate action according to physics/free body diagram etc.

Capacity:
1. Calculate the lowest leg normal force, considering the COG, using physics/free body diagram etc,
2. Calculate capacity by applying coefficient of friction, and
3. Provide fasteners if action is more than capacity, or due to other loads e.g. vibration etc.

Assumptions:
1. Industrial application, and hence conservative answer is acceptable instead of calculation effort, and
2. Unfactored etc, if important local standards etc must be considered.

 

[GregLocock]

The point at which a torque is applied to a rigid body makes no difference.

Cheers

Greg Locock


New here? Try reading these, they might help FAQ731-376

http://eng-tips.com/market.cfm?

 

[hydtools]

If the composite rotational inertia of the table exceeds the motor starting torque reaction, the table will not move.

Ted

 

[desertfox]

Hi SC83

Without any dimensional or mass of components etc it’s hard for us to help very much however if the torque reaction from the motor is not high enough to overcome the friction force between the table legs and the floor then the table won’t move. The table might however vibrate slightly it depends on the torsional resistance of the table legs. For simplicity for the moment assume that the table is rigid and won’t deflect, now take the mass of the table and the mass of the components placed upon it and divide that by the four legs. You now have the mass of the table on each lag which you can convert to a force, then multiply this force by the coefficient of friction and this would roughly be the tangential force provided by the motor to overcome sliding friction on one leg, so multiply this force by four (four legs), take moments about the motor shaft centreline and calculate the tangential force from the motor for each leg and compare that with the tangential force obtained from the friction coefficient earlier, if the tangential motor force is higher the table will move.
This method is not accurate but should give you some idea whether the table needs more mass.

“Do not worry about your problems with mathematics, I assure you mine are far greater.” Albert Einstein

 

[robyengIT]

for me never, never a rigid table is sitting on 4 legs with equal load but on 3. furthermore, looking to the sketch, the CoG is unbalanced in relation to the the motor axis : the leg close to the motor is always lifted/unloaded

 

[rb1957]

very simple questions can have very complicated answers ...

torque will create shear loads, ie in the plane of the floor. And the key to this is friction ...
what is the weight (the normal force at the legs) ? what is a reasonable CoF ?

If you're so concerned about this (this is the 2nd or 3rd thread on this subject/project) then I'd be concerned about the (lateral) stiffness of the table legs ...
their ability to transfer shear loads to the floor.

"Hoffen wir mal, dass alles gut geht !"
General Paulus, Nov 1942, outside Stalingrad after the launch of Operation Uranus.

 

[desertfox]

Whilst I agree about three legs, it won’t make any difference in this case because I was merely getting a rough idea about the tangential force to overcome friction, if it’s divided by three all it will mean is a higher tangential force from the motor to overcome friction, given that the motor rotation point is off centre it’s the top to legs will see the highest rotational force from the motor, by using the four legs my assumption will err on the safe side, for all we know at present the table might weight a couple of tons.

“Do not worry about your problems with mathematics, I assure you mine are far greater.” Albert Einstein

 

[rb1957]

I think each leg (whether it's three or four) reacts the same load. Friction is only the limiting load.

Now, if the table warps then ... all bets are off !?

Oh, and aren't there moments also acting on the table ?

is this better suited for the student forum ? It doesn't sound like a professional problem.

"Hoffen wir mal, dass alles gut geht !"
General Paulus, Nov 1942, outside Stalingrad after the launch of Operation Uranus.

 

[robyengIT]

may be I am rusted and wrong, but the total tangential force is not depending by the area (that is by 3 or 4 legs) but by the friction coeff

 

[desertfox]

No your not wrong robyengT, it’s nothing to do with the area of the legs dividing the total mass of the table and components just gives me a vertical reaction on each leg,from which has you say the coefficient of friction rules. All I am saying is if you divide the total vertical load be three and not four, the value of the vertical reaction will be higher than dividing by four that’s all.

“Do not worry about your problems with mathematics, I assure you mine are far greater.” Albert Einstein

 

[rb1957]

3 vs 4 ... yes, whilst 3 legs gives you a higher normal force (W/3) and so a higher friction "allowable", it also has a higher shear force (T/(sqrt(3)*L)
compared with W/4 and T/(2*sqrt(2)*L) ...

so 3 legs is critical.

"Hoffen wir mal, dass alles gut geht !"
General Paulus, Nov 1942, outside Stalingrad after the launch of Operation Uranus.

 

[desertfox]

I don’t believe the OP is concerned about the torsional stiffness of the table because rereading the post he is concerned about the fact the table isn’t fastened down and further if the table was intended to be fastened down then the torsional stiffness of the table would have been considered, so now I am of the opinion that he just wants to ensure the table doesn’t walk, course we now need more information otherwise we are playing the guessing game

“Do not worry about your problems with mathematics, I assure you mine are far greater.” Albert Einstein

 

[3DDave]

It appears to be related to putting a robot arm onto the table.

It's possible to program that arm to get most any table to walk or overturn by finding natural frequencies.

I'd suggest, if the table cannot be bolted down that a plate be welded to the legs, 4 short rubber feed added to the plate and 500 pounds of sand be placed on the plate.

This can be done in whatever order one likes - before or after the table moves or falls over.

 

[rb1957]

I'd just strap a 50lb shot bag to each leg

"Hoffen wir mal, dass alles gut geht !"
General Paulus, Nov 1942, outside Stalingrad after the launch of Operation Uranus.

 

[Stress_Eng]

This is only a quick pass over the problem, but, from what I understand, you could try the following.
On the assumption that the legs have adjustment, sufficient to enable four legs to be effective.
The arrangement is similar to a bolt group analysis, under gravitational force only (z axis). With the known mass and CG, moments about the X and Y axes through the bolt group centre (BGC) can be calculated (leg contact points with ground). Distribute the moments and Z axis force to the four legs. A free body diagram will show the table to be in equilibrium. With the reactions and the use of a static CoF, the resultant resisting torque can be compared to the applied. With only three legs active, the same approach can be applied.
Hope this gives some ideas.