The equation for shear strength of a RC section without shear reinforcement in the various codes takes into account the ratio of longitudinal reinforcement "in tension", which acts as a crack width limiting factor or through dowel action to aid in shear strength.
Example from EN1992-1-1:
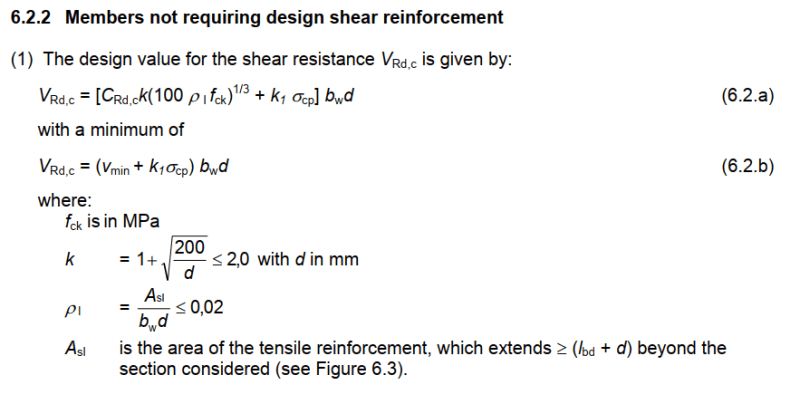
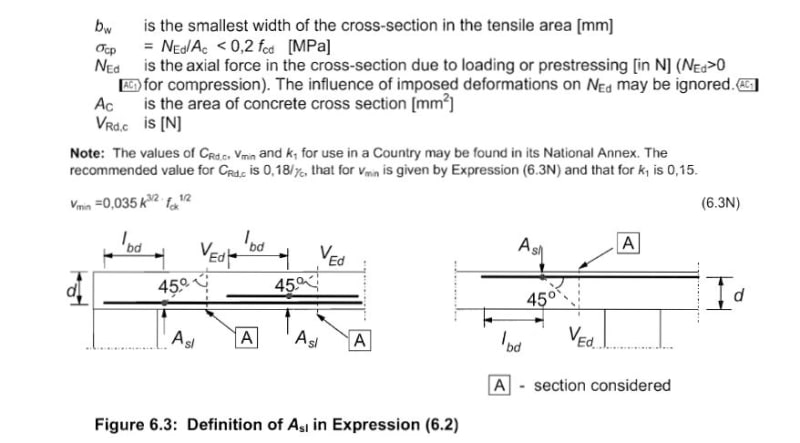
The shear strength is analyzed by means of the truss analogy, which in the general case of flexure works with the uncracked section of concrete above the neutral axis in compression. One question is, what happens when the section is fully in tension and the neutral axis is outside of the section? The code formulas seem to be able to be calculated even with tension as axial force, although this doesn't seem very convincing (in the case of EN1992-1-1, it is possible to break the equation with too much tension and get to absurdly low strength, which would yield orders of magnitude different results with another code).
And my main question, which reinforcement should be plugged in the equation as the reinforcement "in tension"? Take the general case of a beam in tension with As,top << As,bot. It is evident that the strain in the reinforcements will also satisfy εs,top >> εs,bot, implying that cracking will be substantially worse in the top side. Would that be a clue that the top reinforcement should be plugged in the equation, no matter the sign of flexure?
Example from EN1992-1-1:

The shear strength is analyzed by means of the truss analogy, which in the general case of flexure works with the uncracked section of concrete above the neutral axis in compression. One question is, what happens when the section is fully in tension and the neutral axis is outside of the section? The code formulas seem to be able to be calculated even with tension as axial force, although this doesn't seem very convincing (in the case of EN1992-1-1, it is possible to break the equation with too much tension and get to absurdly low strength, which would yield orders of magnitude different results with another code).
And my main question, which reinforcement should be plugged in the equation as the reinforcement "in tension"? Take the general case of a beam in tension with As,top << As,bot. It is evident that the strain in the reinforcements will also satisfy εs,top >> εs,bot, implying that cracking will be substantially worse in the top side. Would that be a clue that the top reinforcement should be plugged in the equation, no matter the sign of flexure?