Snow Drift Fetch Length for Stepped Roofs and Parapets 1

[RFreund]

There was an article in the March 2025 article of Structure Mag by O'Rourke titled Roof Snow Drift due to Ground Snow Load.
See here: https://www.structuremag.org/article/roof-snow-drifts-due-toground-snow/

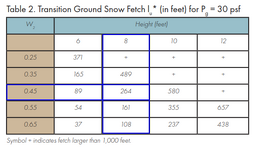
This got me thinking about stepped roofs and what fetch distances should be used. In the article they say that ground snow won't contribute to roof drift until a drift "ramp" is created. They say that the windward drift at the ground has 100% trapping efficiency until a certain ramp height is reached. Then at that point, the ground snow load can be transported to the roof and will contribute to drift on the roof. They solve for what those upwind fetch distances are and provide a table (see below).
I find it a bit odd that they say windward drift has 100% trapping efficiency. In O'Rourke's Snow Loads Guide, he mentions that windward drifts are less efficient at trapping snow and that is why we use 0.75 x the drift height for windward drifts. Can anyone offer any thoughts on this seemingly contradictory information?

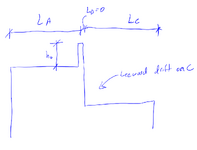
My next question is, can these ground fetch distances be used to determine what fetch distances should be used in the case of stepped roofs and parapets? Here are a couple common examples:




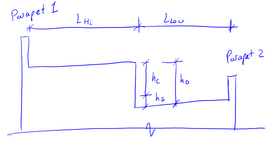
What is the windward fetch distance you should use for parapet 1? Let's say we have the following geometry:
L_Hi = 100'
L_Low = 150'
h_o = 8'
If I look at the table for ground snow fetch length, I would need a fetch length of 264'. Since the lower roof is less than that, the ramp does not form and thus all the snow on the lower roof is trapped at the windward drift. Therefore, my fetch length for parapet 1 = L_hi (100'). Is that correct?
For the fetch distance of parapet 2 I would use 0.75*L_hi + L_low per O'Rourke design guide recommendation.

Another case is show in the article. My question is what is the fetch distance for Roof C leeward drift?


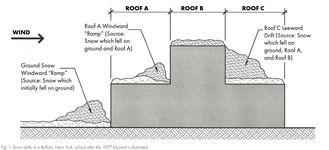
Similar to the previous argument, if the length of Roof A is less than the required fetch distance given in the table (264 feet), then the roof C leeward drift would only be the length of Roof B.
Having said that you can see that he comments that the roof C drift could be due to Roof A, B, and the ground snow. Any thoughts on this?

Thanks!

 

[ANE91]

WW drift is 100% efficient as it fills. LW drift is presumably about 50% efficient as it fills. For both, efficiency drops to nominally 0 once the drift polygon is "filled up." When snow particles begin to "saltate" or jump over the WW wall/step via ramp (Chapter 8 / Paragraph 5), the trapping efficiencies become a bit nebulous. The 0.75 factor is based on perceived differences in trapping efficiencies. Measured drifts in the case history may not have been fully formed; see Fig. G8-2.

Fetch distances are absolutely applicable to stepped roofs/parapets. If a sufficient ramp cannot form at the lower roof, then there's no reason to include snow from that roof in the windward drift on Parapet 1.

Fig. G7-9 (Page 73) covers your final example, from the article, in part. FAQ 9. addresses this question in detail.

I've never seen two engineers do these calculations the same. My $0.02 is that you can't be too conservative with snow.

 

[RFreund]

Thanks ANE91 for the response! I agree, with the adage of no two engineers doing this the same. But now with this required fetch length information, and the 0.85 and 0.75 factors from the design guide, this provides a lot of clarity for many situations.

FAQ 9 actually highlights the discrepancy though in the two methods (i.e. 0.85 reduction vs checking fetch length). I have an older edition of O'Rourke's design guide (it's for the 2005 ASCE 7 snow loads so please correct me if it has changed). Below is the figure used in FAQ 9 and the question is "what is the leeward drift on roof C"


There is a windward obstruction/trap caused by the parapet. In the FAQ they explain that the windward trap is somewhat efficient at trapping snow, therefore we use 0.85*L_A + L_B (which is 0 feet) to find the Leeward drift. This approach neglects the height of the parapet. This solution seems to contradict the notion that a ramp must first be formed on roof A before the snow is transported to roof B which in this case is 0' long so it goes to roof C. As I worked out in the first example where roof B was not 0' long, the fetch distance for the leeward drift on C was only equal to L_B (because h_o was sufficiently tall), so in FAQ9 example the leeward drift would be 0.
If I go back to my example which has the parapets 1 and parapet 2. The windward drift would be L_hi + 0.85*L_low if I use the FAQ9 logic, but if I use the required fetch distance it would only be L_hi.

Having said all this I agree that it seems way too unconservative that with only a fairly short parapet you could ignore leeward drift and your only concern is windward drift. However, I'm curious to know if that is what the data says. Or maybe we don't have enough yet. Or maybe the case of having L_b = 0' is different than having L_b > 0'.
Any thoughts on this?

Intuitively I feel like for my first example with the parapets using L_hi + 0.85*L_low feels too conservative, but if feels correct for the FAQ9 problem. Likewise, if you have L_b > 0 using 0.85*L_A + L_B feels too conservative and looking at the fetch distances seems to make more sense. But physics doesn't care about my intuition, so I'm curious if one is more correct than the other.

 

[ANE91]

this provides a lot of clarity for many situations

I agree!

Below is the figure used in FAQ 9

Looks like this has changed for ASCE 7-16. See below:

Having said all this I agree that it seems way too unconservative that with only a fairly short parapet you could ignore leeward drift and your only concern is windward drift.

I believe the rationale that O’rourke uses is that one of the two will control so there’s no reason to consider them simultaneously, except for in the case of RTUs. The drift provisions are predominantly empirical.


All this is true for the US only. Norway measured much higher drifts, comparatively. So who knows…