xiloergos
Civil/Environmental
- Mar 3, 2024
- 17
Hello everyone. I'm new here. Really cool website. I'm just a carpenter, don't ask too much of me! = )
I'm designing a geothermal system for a possible greenhouse I'll build, but I don't have much money, so I need to make calculations very precisely.
I find myself in need of help, to figure out what the temperature change in air flowing through a corrugated pipe underground would be.
I have this information:
Air: Air will be entering the pipe at 10 degrees Fahrenheit.
Pipe: High density Polyethylene corrugated (solid - without any piercing or knifing) with a thermal conductivity coefficient of 0.5 W/m^K (temperature of the pipe is negligible - meaning it's not insulated or heated)
Surface area of the pipe: The pipe is 100' long with a diameter of 4" (so I believe the surface area is 2.777778 square feet - contact with the ground surrounding it)
Flow: The air is moved by a 270 CFM fan (so I believe traveling at 270 cubic feet per minute)
Surface: The surface surrounding the pipe is soil (I'm going to assume the soil will have a thermal conductivity coefficient of 1.5 W/m^K) and the temperature of the soil is permanently 45 degrees Fahrenheit.
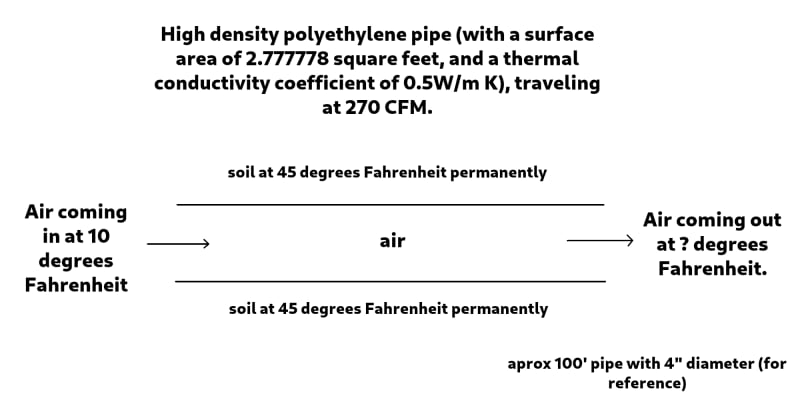
I have attached a very simple diagram with most of this information.
I need to know at what temperature will the air come out?
I really tried to use my head for this, and even tried the chatgpt thingy online, but I think I need the help of real engineers!
Thank you in advance!
Xilo
I'm designing a geothermal system for a possible greenhouse I'll build, but I don't have much money, so I need to make calculations very precisely.
I find myself in need of help, to figure out what the temperature change in air flowing through a corrugated pipe underground would be.
I have this information:
Air: Air will be entering the pipe at 10 degrees Fahrenheit.
Pipe: High density Polyethylene corrugated (solid - without any piercing or knifing) with a thermal conductivity coefficient of 0.5 W/m^K (temperature of the pipe is negligible - meaning it's not insulated or heated)
Surface area of the pipe: The pipe is 100' long with a diameter of 4" (so I believe the surface area is 2.777778 square feet - contact with the ground surrounding it)
Flow: The air is moved by a 270 CFM fan (so I believe traveling at 270 cubic feet per minute)
Surface: The surface surrounding the pipe is soil (I'm going to assume the soil will have a thermal conductivity coefficient of 1.5 W/m^K) and the temperature of the soil is permanently 45 degrees Fahrenheit.

I have attached a very simple diagram with most of this information.
I need to know at what temperature will the air come out?
I really tried to use my head for this, and even tried the chatgpt thingy online, but I think I need the help of real engineers!
Thank you in advance!
Xilo