Strain Compatibilty Method for Reinforced Concrete

[ZeroStress]

Hello and thanks for your valuable advise.

I am struggling with something that has been bothering me for a while. This is regarding finding out the ultimate bending resistance of a rienforced concrete section. I have attached the hand calcs.

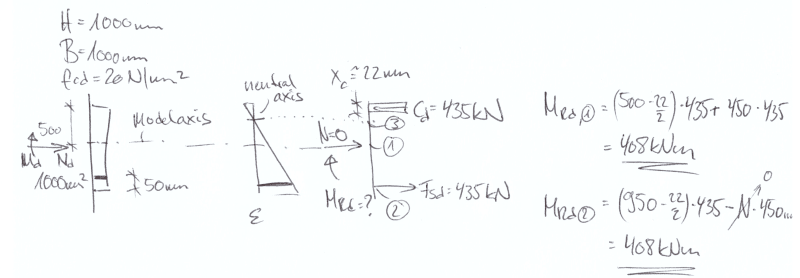
The concrete section in the question already has an axial compression load of 300kN. In my first iteration of x=466mm, I have not been able to balance the section and hence it needs another iteration for the neutral axis depth "x".

However, my actual question is the way I am calculating the bending resistance of the section. Lets say that the equilibrium of the section has been achieved at x = 466mm as in the calcs.

In order to calculate the bending resistance of the section, is it correct to multiply each force with its lever arm as in the "Stress Diagram" figure?

The reason I am asking this question is that I have used AutoDESK Structural Bridge Design software for the same section and the way it calculates the bending resistance is it multiplies each force with its lever arm measured from the centroid of the force to the farthest "opposite stress" fibre.

For example, ASBD software would multimply:
Fsc with lever arm of 732 - 63 = 669mm
Fcc with lever arm of 732 - 186.5 = 545mm
etc

This gives very different bending resistance. Could anyone help please. Thanks.

 

[gargarot89]

Hi

It doesn't matter "around" which point you calculate your bending resistance.
You calculate it about the neutral axis, the ASBD maybe around the bottom reinforcement. In the end its the same.

Your x is way off:
Simple calculation: As_bottom*fsd = 2100*0.435 = 913 kN
N = -300 kN
Fc_sum = 913+300 ~ -1200 kN
xc ~ Fc/fcd/b = 1200/24/0.5 = 100 mm -> x ~ 120 mm (not around 460mm)
You have not yet found the right equilibrium. In your case (x=466mm) your N is about -9 MN and not -300 kN.

And you have forgotten to add P*z to your M_Sum (P acts at the center of your beam/column).

Your resistance should be somewhere about Mrd = -700 kNm.

In most cases, its ok to neglect the reinforcement in compression and moreover in simple cases like yours to ignore the strains. Just assume that all the reinforcement is yielding and the compressionblock is rectangular and about 0.8x to get a pretty good first impression.

 

[Settingsun]

It appears that you've used 1000mm as the section width to calculate Fcc instead of 500mm.

That aside, the moment is calculated about the centroid when the axial force is non-zero. The result will be sensitive to this when the axial force is large. I've seen both the plastic centroid and the elastic centroid of the gross section used for this - plastic section apparently the old way, with gross section more modern on the assumption that's where the member was located in the computer model. In your section, the plastic centroid is at 422mm depth vs 400mm for gross section. There is no difference if the reinforcement is symmetrical.

The Autodesk Bridge method sounds wrong the way you've described it. Consider a section in pure bending: the capacity is the steel capacity multiplied by lever arm, approximately Fs*(0.9*d). The method you've described would give a number about twice this.

At 300kN axial compression, I get 720kNm design bending capacity.

 

[gargarot89]

steveh49 is right about the centroids make a slight difference.

However, the Autodesk Bridge method does not have to be wrong.

If you consider a simple cross section like the following, its all about to sum up all the forces with the right lever arm, but for the calculation itself it does not matter around which point you do it:

My bending resistance is allways the same.
In your example you choose point 3 (the neutral axis). Point 1 (gross/net centroid/model axis) is also possible. Autodesk does it around point 2 as i think and would also give the same result.

back to your question:
"is it correct to multiply each force with its lever arm as in the "Stress Diagram" figure?"
Yes, but you have to consider all the forces in right places and assume the right x for your given axial force and as always: make no mistakes ;]