Symetry versus derived median plane flatness

[Kedu]

From a likedin discussion

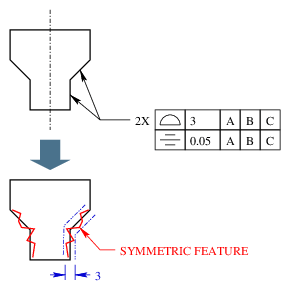
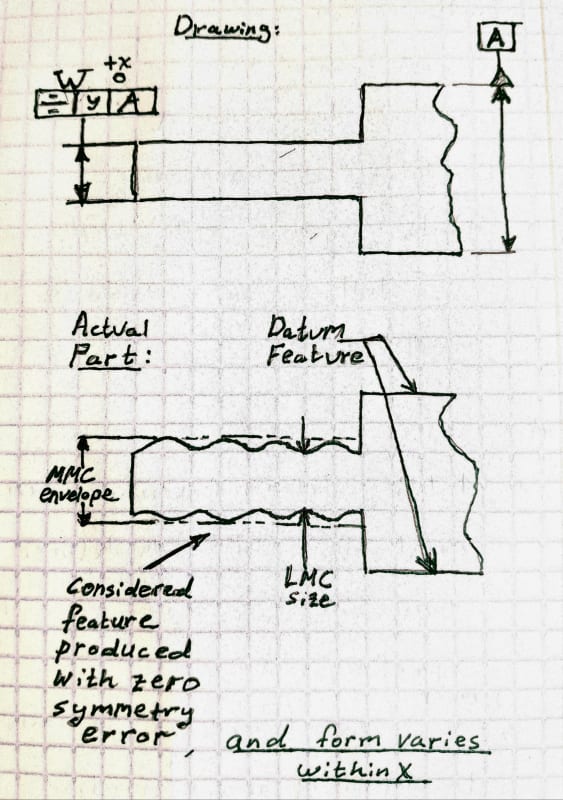
"Conceptually, symmetry is extremely similar to DMP flatness, which is why we're discussing it- just in reference to a datum. If DMP flatness is a form tolerance - and it is - symmetry is also a form tolerance"

Do you guys agree with the statement above?

Sometimes I trust more engtips people than linkedin even some members are the same.
And yes, even some of the linkedin people are y14.5 certified.....

 

[Kedu]

"Datum - because DMPF is only form, and symmetry is strictly form and location

Different cross sections - because DMPF is only form, so orientation is self-referencing, and symmetry is form and location, so orientation is externally referenced.
They still equally control relative profile of the surfaces in exactly the same way "

Another fishy statement ......
How would you comment?
I have my own opinion but I would like to hear other members

 

[Burunduk]

Apart from the obvious difference that one controls location and the other doesn't, an important difference is:

The derived median plane is formed by the center points of line segments which are normal to the center plane of the unrelated actual mating envelope

Symmetry tolerance of a width-type feature controls median points of opposed elements.

If the feature is produced bent, the elements connected by the line segments involved in DMP flatness are non-opposed.
Altogether with the location aspect, the discrepancy is too great for the two to be described as "extremely similar".

 

[chez311]

Actually Y14.5.1 imposes a requirement that is more specific (and perhaps slightly different) than the statement "opposed elements" in Y14.5 taken by itself might suggest.

Concentricity is that condition where the median points (centroids) of all diametrically opposed elements of a figure of revolution (or correspondingly located elements of two or more radially disposed features) are congruent with a datum axis or center point. Symmetry is that condition where one or more features is equally disposed about a datum plane.
[...]
A concentricity or symmetry tolerance specifies that the centroid of corresponding point elements on the surfaces of the actual features must lie in some symmetry tolerance zone.
[...]
Corresponding point elements are obtained by intersecting a pattern of symmetry rays with the actual feature. The rays of symmetry are determined according to Table 5-12.

Thus for a width-shaped feature I can get behind the statement Kedu referenced from linkedin "because DMPF is only form, so orientation is self-referencing, and symmetry is form and location, so orientation is externally referenced". This is because DMP flatness and symmetry would theoretically be equivalent if the referenced datum feature for symmetry was the feature itself (self-referencing) as the datum plane referenced in table 5-12 would simply be the UAME center plane.

This is a result of the fact that for a width-shaped feature the centroids/median points of the rays for symmetry would create a similar imperfect (abstract) plane as the center points of all line segments for a derived median plane - the main difference being that the reference to which these rays/line segments are normal to is external (symmetry) instead of internal (DMP flatness).

This relationship does not carry over to concentricity and DML straightness as the center points of "rays from, and perpendicular to, the datum axis" and the center points of "all cross sections of the feature" do not create analogous geometry. Simply put, the symmetry definition would evaluate multiple center points of rays at each cross section from which a derived median line CANNOT be extracted whereas DML straightness of course evaluates a single center point at each cross section from which a derived median line can (and must) obviously be extracted.

 

[drawoh]

Kedu,

Are we discussing the specification of symmetry? Symmetry has been removed from the ASME Y14.5 standard as of 2018.

In discussions here previously, I have pointed out that I cannot see a situation where I would specify symmetry. Conceivably, I could have a symmetric feature and a composite FCF with a sloppy profile tolerance, and an accurate symmetry tolerance. Why would I do this? If I need a part to be statically or dynamically balanced, I would specify a test of some sort.

If we agree to not specify symmetry, your problem goes away.

--
JHG

 

[chez311]

Hey drawoh, thats my line!

Typically I'm pretty quick to point out it was removed in 2018, I guess I got caught up with the theory and assumed Kedu having been around these parts quite a bit was aware. I figured the OP was just diving into the theoretical specification (judging by the responses provided from the linkedin discussion) itself and not its usefulness on any one application.

I agree the usefulness of symmetry/concentricity in an actual application is dubious and could typically be better served by a different control combined with as you say a balance spec or similar.

 

[Kedu]

Yes, I do know that symetry has been removed from Y14.5 -2108, but the question is still valid for 1994/2009.

Did you guys ever heard about self-referencing symetry applied to a wedge shape feature?

 

[Burunduk]

Yes, I do know that symetry has been removed from Y14.5 -2108, but the question is still valid for 1994/2009.

Did you guys ever heard about self-referencing symetry applied to a wedge shape feature?

Nope, because I haven't got the 2108 standard yet

 

[Kedu]

Per some people"self-referencing symmetry is compliant-consistent with yhe principles in Y14.5 "

And I am talking about 2009 so Burunduk you do not need 2018.

 

[drawoh]

Kedu,

Here is the solution. What is the problem?

I am not trying very hard to understand this. If I want to control straightness or flatness, I need to specify straightness, flatness, or profile, or apply an accurate dimension. Note how rule[ ]1 controls straightness. If the profile is accurate, straightness and symmetry are controlled.

In the above example, symmetry requires that the RHS error be repeated on the LHS. Straightness and flatness are controlled by the profile.

--
JHG

 

[Kedu]

Guys,
A follow up question:
Is the symmetry a location AND form control or a location control only?

In my opinion symmetry can be applied to a rFOS only and the feature's form is controlled by rule#1 and not by the symmetry tolerance value?

What do you think?

Am I correct in saying that that symmetry can be applied to regular features of size rFOS?
Also what about its clasification? Is it location AND form or not?

 

[drawoh]

Kedu,

In ASME Y14.5-2009, symmetry is described in the section on tolerances of location. In ASME Y14.5-2018, it is not described anywhere.

--
JHG

 

[Burunduk]

Kedu,
Suppose that a feature is specified with a directly toleranced dimension and a symmetry tolerance. Can its form vary within the bounds of the envelope of perfect form at MMC by the entire size tolerance without any additional restriction? If yes, the symmetry tolerance does not add any restriction on the form variation i.e. it does not control form.

Edit: The figure is intended to represent a situation that all median points along the considered feature form a flat plane centered to datum A.

 

[chez311]

I'd say its as much a form control as DMP flatness. As I noted before the only difference is that the center plane reference is external (datum plane) vs internal (UAME center plane).

 

[Kedu]

Thank you very much.
The other point of contention has been if derived median plane flatness DMPF could be legally (according to Y14.5) applied to a wedge dimensioned with basic size dimension and not associated with a plus - minus dimension?
As I currently know and seen, all examples for DMPF are shown as flatness FCF associated with +/- dimension, but what about basic dimension?
Is it valid or in agreement with the standard such a combo?

 

[pmarc]

Burunduk,
In you illustration, what do you mean by "and form varies within X"? Which feature's form variation do you have in mind?

 

[Burunduk]

pmarc,
I mean the surface variation, not the variation of the theoretical surface formed by the median points related to the symmetry tolerance. I know that surface variation is not what symmetry controls, but I think that for the purpose of making a decision whether or not symmetry can be considered a form control (which is not how the standard classifies it), all types of possible form restrictions should be considered.

The symmetry "form control" of imperfect resolved geometry aspect needs to be addressed too.
I know that DMP flatness does not control the surface variation either, and symmetry can easily be thought of as limiting the imperfect resolved geometry variation but - there is a fundamental difference: DMP flatness overrides rule#1 and becomes the sole form control where no other form controls are specified. Symmetry overrides nothing and depending on its tolerance value and the size tolerance value it may or may not have any influence on the form variation. As such, I don't think it can be considered a form control.

 

[chez311]

Burunduk,

The fact that DMP flatness overrides rule #1 is an interesting notable difference, but it does not change the underlying principle which is common within the two controls. It just means that the form deviation allowed by symmetry is limited by rule #1 even if the symmetry tolerance applied is greater than MMC-LMC, unless the Independency symbol is applied.

Symmetry overrides nothing and depending on its tolerance value and the size tolerance value it may or may not have any influence on the form variation

Again, I already showed how a self referencing symmetry control would be identical to DMP flatness. This influence on form deviation would not be any greater or less than DMP flatness. If the tolerance applied were greater than MMC-LMC, then all one would have to do is apply the Independency symbol to symmetry and they would again be identical as symmetry does not by default override rule #1.

 

[Burunduk]

It just means that the form deviation allowed by symmetry is limited by rule #1 even if the symmetry tolerance applied is greater than MMC-LMC, unless the Independency symbol is applied.

Another way to look at it is that the form deviation allowed by rule #1 is unaffected by the symmetry control when the symmetry tolerance is greater than MMC-LMC, unless the independency symbol is applied.

Again, I already showed how a self referencing symmetry control would be identical to DMP flatness

It would be so if the symmetry control:
1. Would be allowed to be self-referencing.
2. Would override rule #1 and not require the independency symbol to act the same.
3. Would be required that when used in conjunction with an orientation tolerance, the symmetry tolerance must be smaller.
4. Would be allowed to be applied with an MMC modifier.

That is a lot of "woulds".
DMP flatness comes with all sorts of auxiliary rules that indicate that its role is to control form. Symmetry comes with none of them.