ForestMoon
Mechanical
- Jun 29, 2016
- 5
Hi all,
I was reading the original thread (Link) regarding the origin of the 2% bracing force and no definitive answer was given as to its origin, which led me to reading the Guide to Stability Design Criteria for Metal Structures 6th Edition (2010) by Ronald D. Ziemian.
Chapter 12 seems to offer an approximate answer to this question in its first paragraph (whilst still not definitive):
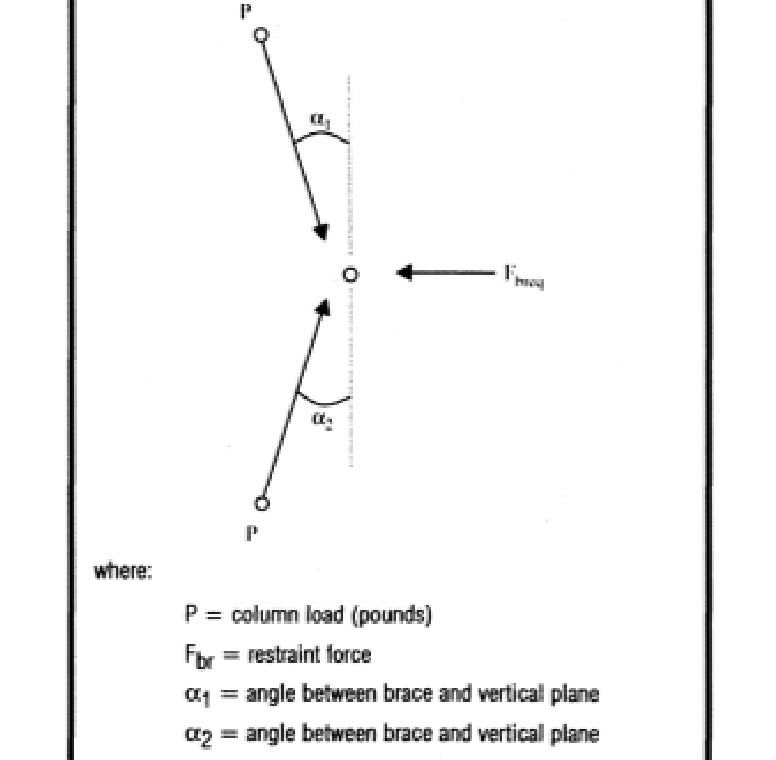
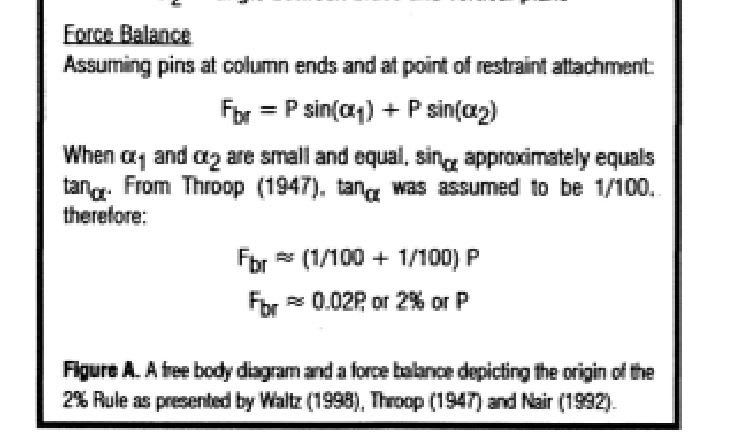
In Chapter XVI of Bridge Engineering (1916) by Waddell, the following equation is given to calculate the shear S to be carried by the lacing in percentages of P, the total load on the member, as a function of the slenderness l/r of the compression member:
For a typical l/r = 75, S/P = 1.7 %.
Hope it can help someone in the future.
I was reading the original thread (Link) regarding the origin of the 2% bracing force and no definitive answer was given as to its origin, which led me to reading the Guide to Stability Design Criteria for Metal Structures 6th Edition (2010) by Ronald D. Ziemian.
Chapter 12 seems to offer an approximate answer to this question in its first paragraph (whilst still not definitive):
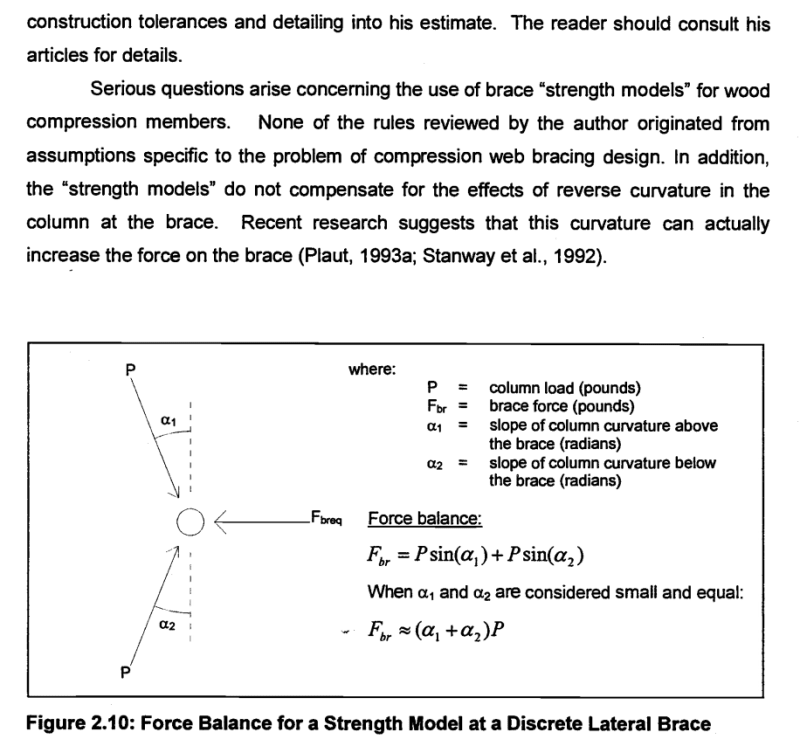
Ronald D. Ziemian (2010) said:Stability bracing requirements first appeared in the early 1900s related to the design of lacing in the built-up members of trusses (Waddell, 1916). Numerous railroad truss failures prompted the development of the 2% rule—the lacing shear force equals 2% of the force in the column. The lacing rule was most likely simply extended by structural engineers to all stability bracing situations, primarily as a result of steel design specifications in the United States not containing general bracing requirements until 1999. In the 1970s, the New York City building code contained the 2% rule for stability bracing but no stiffness requirements.
In Chapter XVI of Bridge Engineering (1916) by Waddell, the following equation is given to calculate the shear S to be carried by the lacing in percentages of P, the total load on the member, as a function of the slenderness l/r of the compression member:
J. A. L. Waddell (1916) said:S = (200P)/(16,000-60 l/r)
For a typical l/r = 75, S/P = 1.7 %.
Hope it can help someone in the future.