BLUEPUPIL
Structural
- Mar 29, 2015
- 13

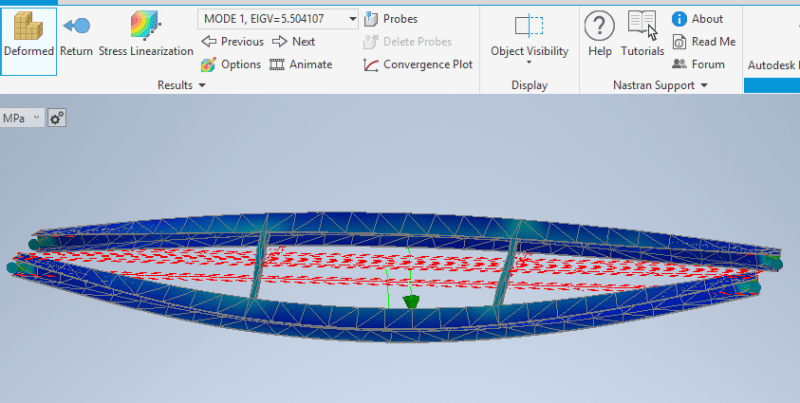
Can I assume (from the picture) that the unbraced length equals the distance between diaphragms?
Follow along with the video below to see how to install our site as a web app on your home screen.
Note: This feature may not be available in some browsers.
retired13 said:The weak axis (single girder) becomes strong axis (two girders assembly)...
BridgeSmith said:It's actually only subject to LTB as a system if the girders are deep enough and close enough together that the vertical axis remains the strong axis of the system.

BridgeSmith said:Likely you can assume the unbraced length for the individual girders is the distance between the diaphragms.
STrctPono said:In other terms, if you're particularly interested in checking lateral torsional buckling of a single girder, yes, you can assume that the unbraced length is equal to the distance between the diaphragms.
Good post. And good blog posts. (I think I've already said that before, but no harm it extra compliments.)Agent666 said:So instead of guessing a sometimes conservative effective length based on applying various factors to the member length based on the type of restraints, you're zeroing in on the theoretical effective length based on your boundary conditions/restraints that you modelled. I recently did a series of blog posts on buckling analyses, the concepts are fairly universal even though I focused on the NZ & Australian steel codes, many other codes around the world deal with buckling analyses in a similar manner. Posts 1 & 2 are about the basics of an axial buckling analyses, posts 3, 4 & 5 are about flexural buckling analyses.
KootK said:Are you not able to brace the top flange of your beam to the floor or roof structure above? That's normally the case.
Agent666 said:Your best beat to nut out the true buckling behaviour and hence the design capacity is to do a buckling analysis in these scenarios.
human909 said:I did have a quick play with this behaviour. With I-beams, reasonable diaphragm spacing, and without the top restrain from a deck you need to have pretty long spans to get the the global LTB effect.
Agent666 said:So as Klitor noted I'd expect the equivalent effective length is more likely to be over several diaphragm bays.

I’m not quite confident, that timber joists have enough bracing potential to restrain steel girders.
Am I on the safe side, if I skip buckling analysis and provide lateral restraints to the twin girder, doubling Lb. 2x1.5м=3м? (see the drawing)
Agreed.BridgeSmith said:If they're connected, they should be adequate bracing. It takes very little restraint to keep the beams straight, unless they are allowed to move significantly
BLUEPUPIL said:To simplify it a bit:
Girder Span= 9м
Load [LL+DL]= 20kN/m
1.First variant- Single girder- HEA360; weight=112kg/m.
2..Second variant- Twin girder with diaphragms (where the unbraced length is equal to the distance between the diaphragms 1.5м)- 2xHEA220; weight=2x50.5=101kg/m.