Ally0138
Petroleum
- Aug 30, 2017
- 9
Hi,
Does anyone have formulae for calculating the following:
[ol 1]
[li]The volume of liquid in a partially filled horizontal conical frustum?[/li]
[li]The corresponding 'wetted area' of the frustum?[/li]
[/ol]
Refer to below diagram. I wish to calculate the volume of liquid and the wetted area of the frustum for a liquid height of h within a horizontal frustum of large diameter d1, small diameter d2 and length L.
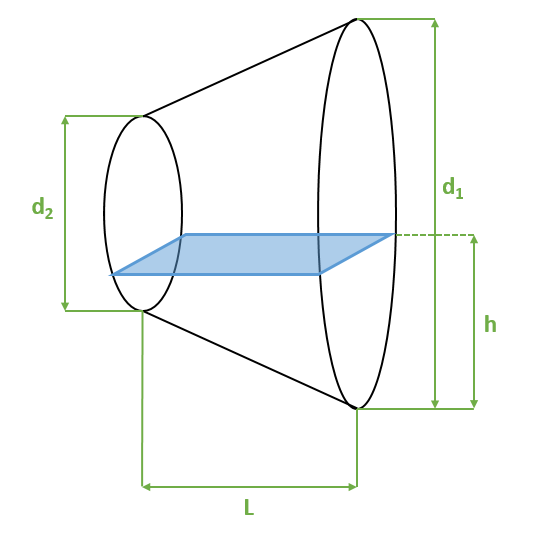
Thanking you in advance for your suggestions.
-Ally-
Does anyone have formulae for calculating the following:
[ol 1]
[li]The volume of liquid in a partially filled horizontal conical frustum?[/li]
[li]The corresponding 'wetted area' of the frustum?[/li]
[/ol]
Refer to below diagram. I wish to calculate the volume of liquid and the wetted area of the frustum for a liquid height of h within a horizontal frustum of large diameter d1, small diameter d2 and length L.

Thanking you in advance for your suggestions.
-Ally-
